Background: Studies on the nutritional and health benefits of pulses have stimulated interest in using whole pulses and pulse fractions in novel value-added food products. In this study the effects of using different types and concentrations of gums (including xanthan gum [XG], mixtures of XG and gum arabic [GA], XG and propylene glycol alginate [PGA], XG and pectin [PE], and XG and guar gum [GG]) on the physical properties (i.e., rheology, texture, color and particle size distribution) of lentil flour-supplemented (3.5w/w%) salad dressings were systematically examined. Response surface methodology was used to study the main effect of the two independent variables (x₁, oil concentration; x₂, gum concentration) on the generated responses and to optimize emulsion composition using commercial salad dressing parameters as reference.

Results: An increase in both gum and oil concentrations enhanced emulsion firmness and viscosity by contributing to a more compact networked structure in the emulsion. Large droplets formed in the presence of higher gum concentrations at lower oil content. Color characteristics were affected to different extents depending on type and concentration of gum.

Conclusion: The validation test showed overall adequacy of the final response surface models employed to predict properties of the lentil-supplemented salad dressing formulations.

Keywords: salad dressing, lentil supplementation, rheology, particle size, food hydrocolloids, response surface methodology.

Growing consumer demand for low-fat and cholesterol-free food products have increased interest in the use of plant-derived food ingredients. Pulses (including dry bean, pea, chickpea, and lentil) are the second largest source of human food and animal feed worldwide.¹ Due to their unique nutritional and functional properties, pulse ingredients such as whole flours, protein, starch and fiber fractions are being explored in various novel value-added processed food products, Including bread, meat products, yogurt, pasta and salad dressings.^2-4

Salad dressings encompass a broad range of oil-in-water emulsion products, which vary in fat content (20-65%) and viscosity. Emulsions are thermodynamically unstable systems, and the incorporation of emulsifiers and/or thickening agents is critical for obtaining a stable emulsion with acceptable quality. Emulsifiers are able to decrease the interfacial tension between the oil and water phases, and are able to prevent droplet aggregation by forming a protective coating around the droplets. Thickeners can impart long-term stability by thickening the emulsion system (i.e., reducing the movement of the system) and by forming viscous, ordered networks in the continuous phase to prevent oil separation.⁵ Non-starch polysaccharides, one of the most widely used thickeners in food applications, are able to impart textural attributes and mouth-feel to food systems. Most of these are hydrophilic, except for gum arabic, Propylene glycol alginate, and hydroxypropyl methylcellulose, which are amphiphilic and can prevent droplet aggregation by steric and/or electrostatic forces. The degree of texture modification associated with these hydrocolloids is dependent on the gum concentration used, the molecular weight of the polysaccharides and their functional groups, as well as on the degree of interaction between mixed gums.⁶

Mixtures of hydrocolloids may act synergistically to increase viscosity or antagonistically to reduce it. Their interactions have been studied extensively in an effort to generate new functionality or to manipulate the texture and rheology of food systems, with the ultimate goal of replacing expensive polysaccharides by cheaper alternatives.⁷

Xanthan gum is the extracellular anionic heteropolysaccharide produced by fermentation of the bacterium Xanthomonas campestris. Xanthan consists of pentasaccharide repeating units formed by a (1-4)-β-D-glucan backbone linked to a charged trisaccharide side chain (β-D-mannopyrannosyl-(1-4)-α-D-glucopyrannosyl-(1-2)-β-D-mannopyrannosyl-6-O-acetate) at the 3 position on alternate glucose residues.⁸ Xanthan gum exhibits pseudoplasticity and thixotropy and xanthan gum solutions have high yield stress making them useful for stabilizing salad dressings.⁹

Gum arabic is a natural exudate obtained from the stems of Acacia senegal. Structurally, it is a high molecular weight charged heteropolysaccharide consisting of branched galactanheteropolymers. Hydrolysis results in D-galactose with lesser amounts of L-arabinose, D-glucuronic acid and L-rhamnose, along with a small amount of 4-O-methyl-D-glucuronic acid.^8-10 Gum arabic solutions are the least viscous of the natural food-grade polysaccharides.¹⁰ The structure of gum arabic comprises an approximately 2% protein component which is covalently linked to the polysaccharide moiety.¹¹

Propylene glycol alginate is a derivative of alginic acid with an average molecular mass ranging from 30,000 to 200, 000 Daltons. It is a surface active biopolymer which has both hydrophobic and hydrophilic groups, and could therefore cause a reduction in the surface tension of the oil and water surfaces.^12,13

Pectin is a naturally occurring polysaccharide which is present in the primary cell walls of almost all terrestrial plants. It is usually extracted from citric fruits and apples. Pectins are a group of heteropolysaccharides which contain at least 65% by weight of galacturonic acid-based units, which may be partially esterified with a methoxyl group. Pectins are often classified according to their degree of esterification (DE): the ones with DE of up to 50% are classified as high methoxylpectins (HMP), and those with DE of less than 50% DE are classified as low methoxylpectins (LMP).^14,15

Guar gum is a galactomannan polysaccharide which is formed by galactose and mannose molecules. It is obtained from the endosperm of the seed of Cyamopsis tetragonolobus. The principal backbone of guar gum is a chain of (1-4)-β-D-mannopyranosyl units, with single (1-6)-α-D-galactopyranosyl units linked to the principal chain.^16,17 The interactions between xanthan and guar gum have been studied extensively,^17-19 with several types of evidence supporting the existence of intermolecular binding between xanthan and galactomannans.

Salad dressings offer an opportunity to expand the utilization of pulse ingredients. Although several studies have examined the thickening effect of different hydrocolloids and combinations of hydrocolloids used in food product applications (such as gravies, dairy products, food drinks and pet foods), no systematic studies have evaluated the impact of using different types and concentrations of gums or combinations of gums on the physical properties of salad dressings made using pulse ingredients. The role of lentil flour in the formulation of salad dressing as thickening agent was investigated in preliminary studies (data not published). Owing to the complex composition and structure of food systems, food hydrocolloids may exhibit a wide range of structural transitions and rheological properties under different conditions and at various concentrations in food emulsions. The present study was, therefore, undertaken to investigate the influence of different types and levels of single or hydrocolloid mixtures (including xanthan gum [XG], mixtures of XG and gum arabic [GA], XG and propylene glycol alginate [PGA], XG and pectin [PE] and XG and guar gum [GG]) on the physical properties (i.e., rheological, textural, color and particle size characteristics) of lentil flour-supplemented salad dressing, with the aim of assessing the feasibility of using such pulse ingredients in salad dressing formulations.

Raw materials

Whole green lentil flour was provided by the Canadian International Grains Institute (Winnipeg, MB, Canada). Spray-dried egg yolk powder was obtained from Canadian Inovatech Inc. (Winnipeg, MB, Canada). The following gums were kindly provided by Tic Gums (Belcamp, MD, USA): xanthan gum (TIC Pretested® Ticaxan® Xanthan Powder), gum arabic (TIC Pretested® Gum Arabic FT Powder), PGA (TICA-algin® PGA LV powder), guar gum (TIC Pretested® Guar gum 8/24 powder), pectin (TIC Pretested® pectin HM Slow Set Powder). Potassium sorbate was provided by Nealanders International Inc. (Mississauga, ON, Canada). All other ingredients used in the preparation of the salad dressings were purchased from a local supermarket. All other chemicals used were of analytical grade.

Salad dressing sample preparation

Salad dressings were prepared using different combinations of oil (7.5%-35.0%) and gums (0.2%-0.5%) as described in section 2.7. The five different types and combinations (ratios) of gums used were XG; XG: GA=0.5:1; XG: PGA= 0.5:1; XG: PE=1:1; XG: GG=1:1. The specific ratios were selected based on the work reported previously.^20-22 The other ingredients in the recipe were as follows (expressed as a percentage [w/w]): whole green lentil flour 3.5%, vinegar 7.0% (with 5% acetic acid), lemon juice 5.0%, salt 1.0%, and sugar 3.5%. The gums, or combinations of them with appropriate concentrations (0.2%, 0.35%, and 0.5%), and sugar were prepared in advance by mixing the desired amount of dry sample with deionized water while continuously dispersing the gum and sugar solution with a magnetic stirrer at ambient temperature for 2 h. The resulting dispersions were stored overnight to ensure complete hydration prior to adding other ingredients. All other ingredients except oil were then added and mixed until homogeneous. Potassium sorbate (0.02 wt %) was added as an antimicrobial agent. Lastly, canola oil was added and emulsification was achieved using an Ultra-Turrax homogenizer (Model T25, Janke& Kunkel, Ika-Labortechnik, Staufen, Germany) equipped with a S25-18G dispersing tool at 13,800 rpm for 3 min. All measurements were performed the day the samples were prepared.

Texture analysis

Texture analysis was performed with a TA-XT2 texture analyser (Stable Micro System, UK). Salad dressing samples were placed in cylindrical bottles (60 mm diameter × 10 mm height) and were punctured with a cylindrical probe (25 mm diameter × 35 mm height) in a 5000 g load cell at a crosshead speed of 1.0 mm/s. Firmness values were measured and were taken as the height of the peak force during the first compression.

Rheological measurements

Rheological measurements were performed with an AR 1000 rheometer (TA Instruments, New Castle, DE, USA) equipped with a plate/cone system. Steady-state flow tests and dynamic oscillatory tests were conducted using a stainless steel parallel plate with a diameter of 4 cm. The gap setting was 1 mm. One tablespoon of sample was placed at the center of the circular plate, and excess sample was removed from the edges of the plate. The steady-state flow tests were performed at increasing shear rates (0.02 to 300 s-1). Experimental flow curves were fitted using the power law:

$\eta =m{\gamma }^{(n-1)}$

wheren is the flow behavior index (dimensionless), η is the shear viscosity (Pa.s), m is the consistency coefficient (Pa.sⁿ), and $\gamma$  is the shear rate (s^-1). Dynamic oscillatory tests were then performed within the LVE range at 0.1% strain with angular frequency increasing from 0.1 to 100 rad/s. Storage modulus (G′) and loss modulus (G″) vs. angular frequency were measured for all samples.

Color measurements

The color of the salad dressing samples was measured with the L*, a*, b* tristimulus system using a Minolta CM-503c spectrophotometer (Minolta Co. Ltd., Osaka, Japan). A fixed amount of salad dressing was poured into the measuring cup, which was then surrounded with a black paper strip. In this color system, L* is a measure of lightness to darkness (0=black and 100=white), a* is a measure of redness (+ve) to greenness (−ve), and b* is a measure of yellowness (+ve) to blueness (-ve). The data were also characterized in terms of chroma (C) and color difference (ΔE) to highlight differences between the samples:

$C={(a^2+b^2)}^{1/2}$

$\Delta E={(\Delta L^2+\Delta a^2+\Delta b^2)}^{1/2}$

where ΔL, Δa, Δb are the color differences when compared with the color parameters of milk.

Droplet size distribution

The particle size of the salad dressings was analyzed by laser light-scattering using the Mastersizer 2000 MU particle size analyzer (Malvern Instruments Ltd, Worcestershire, UK) with the Hydro 2000MU accessory using distilled water as a dispersant at the speed of 2000 rpm. In keeping with the procedure described by Worrasinchai et al.²³ the results were reported as the specific weighted mean diameter (or Sauter mean diameter D[3,2]) and the volume-weighted mean diameter (D[4,3]), respectively,

$D[3,2]={\displaystyle \sum n_i{d}_i^3}/{\displaystyle \sum n_i{d}_i^2}$ ,

$D[4,3]={\displaystyle \sum n_i{d}_i^4}/{\displaystyle \sum n_i{d}_i^3}$

Where n_i is the number of droplets of diameter d_i. The specific surface area (m²/ml) was also calculated using the following equations:

$Specificsurfacearea=\frac{6\times oilfraction}{D[3,2]}$

Statistical analysis

Response surface methodology (RSM) was used to study the main effect of the component variables on the physical properties of lentil flour-supplemented salad dressings prepared with XG, XG-GA, XG-PGA, XG-PE, and XG-GG. A RSM Miscellaneous experimental design with 3² full factorials and 5 center runs was used with two independent variables (x₁, oil concentration: 7.5%, 21.25%, 35%; x₂, gum concentration: 0.2%, 0.85%, 1.5%) at three coded levels (-1, 0, +1) and five replicates at the center point(oil concentration, 21.25wt%; gum concentration, 0.85wt%). A complete design consisted of 14 experimental runs for dressings formulated with each gum.

The effect of the two independent variables on the following responses was modeled and optimized using RSM: firmness (Y1), flow behavior index n (Y₂), consistency coefficient m(Y₃), apparent viscosity ηap (Y₄), plateau modulus  (Y₅), L* value (Y₆), a* value (Y₇), b* value (Y8), ΔE (Y₉), chroma (Y₁₀), D[3,2] (Y₁₁), D[4,3] (Y₁₂), and specific surface area (Y13). All the response surface plots were generated by keeping one variable constant at the center point and varying the other two variables within the experimental range. The regression model selected for predicting individual Y variables is given by the following equation:

$Y={\beta }_0+{{\displaystyle \sum {\beta }_{i}x}}_i+{{\displaystyle \sum {\beta }_{ii}{}^{2}x}}^2{}_{ii}+{\displaystyle \sum {{\displaystyle \sum {\beta }_{ij}x}}_i}{x}_j+\epsilon$

Where Y is the predicted response, β0 ­is the constant, β_i, β_ii, β_ij are the regression coefficients, and x_i, x_jare the levels of independent variables.²⁴ One-way analysis of variance (ANOVA) was used to evaluate the significant terms in the model for each response. The adequacy of models was ensured by removing the non-significant terms (P>0.05) using a step-wise “backward” multiple reduction algorithm.

The overall optimization was carried out within the range of experimental conditions. The responses obtained for five types of pourable commercial salad dressing were used as the target values to predict the optimal formulation during optimization procedure. The optimal levels of the two independent variables leading to the desired properties were obtained for each gum or combination of gums. Design Expert, version 7 (Stat-Ease Inc., Minneapolis, MN, USA), was used to design the experiments, generate the response surfaces and optimize the variables.

The associated R² and adjusted R² for selected responses for the 14 × 5 experiments carried out using the Miscellaneous experimental design as described in section 2.7were computed. Coefficients for the linear, quadratic, and interaction terms of each model were calculated and tabulated for this response in Table 1. Some responses could not be fitted satisfactorily to mathematical models due to lack of fit or poor fit with low R² or adjusted R² values. The discussion therefore only addresses responses which exhibited statistically significant predicted models (P<0.05) with non-significant lack of fit (P>0.05).

Regression models of firmness

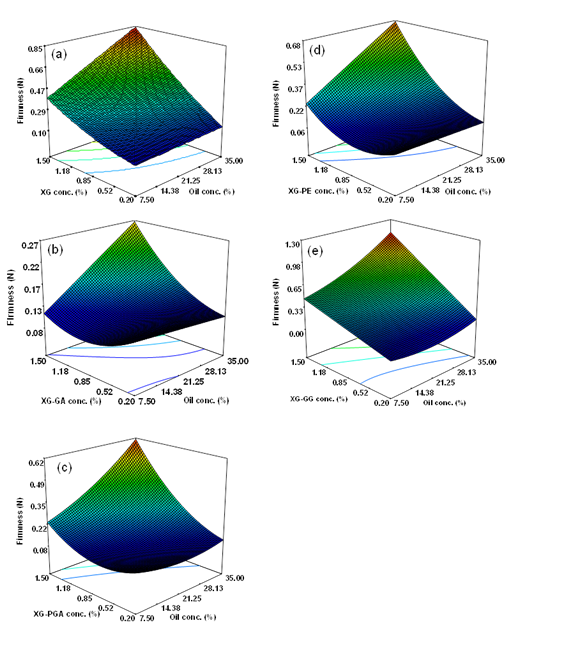
Figure 1 Response surface plots of firmness (N) for dressings prepared with (A) xanthan gum (XG), (B) xanthan gum-gum Arabic (XG-GA), (C) xanthan gum-propylene glycol alginate (XG-PGA), (D) xanthan gum-pectin (XG-PE), and (E) xanthan gum-guar gum (XG-GG).

Figure 1 shows the response surfaces of firmness for the dressing emulsions prepared with different gums as a function of the component variables (x₁, oil; x₂, gum). As shown, firmness (Y₁) for all gums and combinations thereof exhibited a similar increasing trend in response to increasing oil concentrations (x₁) at higher gum content. For dressings prepared with XG-GA, XG-PGA, and XG-PE (Figure 1B-1D), an increase in the gum concentrations (x₂) yielded higher firmness (Y₁) values at higher oil content (x₁), whereas dressings with XG and XG-GG showed an increasing trend in Y₁ with an increase in gum content (x₂) at all oil concentrations within the experimental range studied (Figure 1A & 1E). The results are consistent with those of Raymundo et al.²⁵ which showed that firmness increased with increasing protein, xanthan gum, and oil concentrations in a low-fat oil-in-water emulsion reportedly.

The significant second-order regression models with R² of 0.982, 0.849, 0.987, 0.956, and 0.974 for dressings prepared with XG, XG-GA, XG-PGA, XG-PE, and XG-GG are presented in Eq. 1, 2, 3, 4, 5, respectively.

$Y_1=0.103-0.0014x_1-0.031x_2+0.0117x_1{x}_2+0.0961x_2{}^2$ (Eq. 1)

$Y_1=0.165-0.0017x_1-0.176x_2+0.0049x_1{x}_2+0.076x_2{}^2$ (Eq. 2)

$Y_1=0.266-0.012x_1-0.359x_2+0.0111x_1{x}_2+0.0002x_1{}^2+0.203x_2{}^2$ (Eq. 3)

$Y_1=0.223-0.0036x_1-0.472x_2+0.0129x_1{x}_2+0.27x_2{}^2$ (Eq. 4)

$Y_1=0.153-0.019x_1+0.168x_2+0.016x_1{x}_2+0.0004x_1{}^2$ (Eq. 5)

Regression models of rheological parameters

The regression models for responses, including flow behavior index (n value), consistency coefficient (m value), apparent viscosity (ηap), and plateau modulus ( $G_N^0$ ), are discussed below.

Regression model of the flow behavior index (n value): The flow behavior index (n) obtained using the power law model was less than 1 (0.1 to 0.7) for all the salad dressings indicative of pseudoplastic behavior. An acceptable food emulsion with high viscosity and good mouth feel should have a low n value as gum solutions with high n value tend to be slimy in the mouth.²⁶

The multiple regression equations for dressings prepared with XG, XG-PGA, XG-PE, and XG-GG (R²values of 0.9668, 0.755, 0.651, and 0.963, respectively) are presented in Eq. 6, 7, 8, and 9, respectively.

$Y_2=0.506-0.003x_1-0.608x_2+0.003x_1{x}_2+0.232x_2{}^2$  (Eq. 6)

$Y_2=0.475-0.159x_2$  (Eq. 7)

$Y_2=0.554-0.277x_2$  (Eq. 8)

$Y_2=0.588-0.007x_1-0.567x_2+0.006x_1{x}_2+0.158x_2{}^2$  (Eq. 9)

From the above equations, it is evident that for dressings prepared with XG-PGA or XG-PE, the flow behavior index (Y₂) was dependent (P<0.05) solely on gum concentration (x₂) (Eq. 7, 8). Quadratic equations (Eq. 6, 9) were obtained for dressings prepared with XG and mixed XG-GG.

As shown in Figure 2, the statistical surface representing Y2was dependent on oil (x₁) and gum content (x₂) for dressings formulated with XG and XG-GG (Figure 2A & 2D). As predicted by the statistical model, n value was dependent only on gum concentration (x₂) for XG-PGA and XG-PE (Figure 2B & 2C). In general, for all dressings, an increase in the concentration of x₂was accompanied by an increase in pseudoplasticity, as evidenced by a decrease in the values of the flow behavior index. This suggests that the magnitude of change in apparent viscosity corresponding to the change in shear rate tended to increase as the gum concentration increased in the continuous phase of the emulsion. For the dressing with XG alone, the tendency for the n value to decrease was less pronounced when the gum concentration was between 1.18% and 1.50%, which suggests that the pseudoplastic behavior remained quite stable above a threshold XG concentration in the salad dressing system.

In a dressing system, gums may interact with proteins from egg yolk and pulses, with starch from pulses, as well as with each another. The extent of these interactions, which is dependent on the gum concentration, polysaccharide molecular weight and the presence of functional groups of polysaccharides, may explain the differences in the rheological behavior of the dressing systems prepared with different gums.

Regression models of the consistency coefficient (m value): The secondary-order polynomial models fitted to the m value (Y₃) are presented in Eq. 10, 11, 12, 13, and 14 for dressings prepared with XG, XG-GA, XG-PGA, XG-PE, and XG-GG (R² values of 0.995, 0.977, 0.989, 0.986, and 0.993, respectively.

$Y_3=-0.906-0.175x_1+0.432x_2+1.408x_1{x}_2+20.899x_2{}^2$  (Eq. 10)

$Y_3=7.308-0.752x_1-12.306x_2+0.531x_1{x}_2+0.0162x_1{}^2+7.406x_2{}^2$  (Eq. 11)

$Y_3=13.715-1.223x_1-33.92x_2+1.12x_1{x}_2+0.025x_1{}^2+25.183x_2{}^2$  (Eq. 12)

$Y_3=22.081-1.937x_1-46.443x_2+1.797x_1{x}_2+0.035x_1{}^2+29.839x_2{}^2$  (Eq. 13)

$Y_3=8.166-0.172x_1-79.796x_2+2.536x_1{x}_2+78.926x_2{}^2$  (Eq. 14)

All the dressings had similar response surface plots for them value (Y₃) as that shown in Figure 3(A). A synergistic effect between gum(s) and oil can be observed in the response plots, with maximum mvalues achieved at the highest combination level for the two variables. This behavior was most pronounced for dressings prepared with XG and XG-GG. This observation is supported by earlier reports on hydrocolloids solutions indicating that the magnitude of the consistency coefficient increased with an increase in the concentration of the GA-GG combination, as well as the GA-XG combination.⁶ Marcotte et al.²⁷ also reported that m value increased with increasing gum content for carrageenan, pectin, gelatin, starch and xanthan gum. The increase in the viscous nature of the salad dressings (m value) with increasing oil content, especially at high gum concentration(s), is suggestive of the formation of a more compact network structure. Overall, the magnitude of the consistency coefficient (Y₃) was highest for dressings prepared with XG-GG and XG and lowest for XG-GA within the range of concentrations studied. The dressings prepared with XG-PE and XG-PGA had intermediate values.

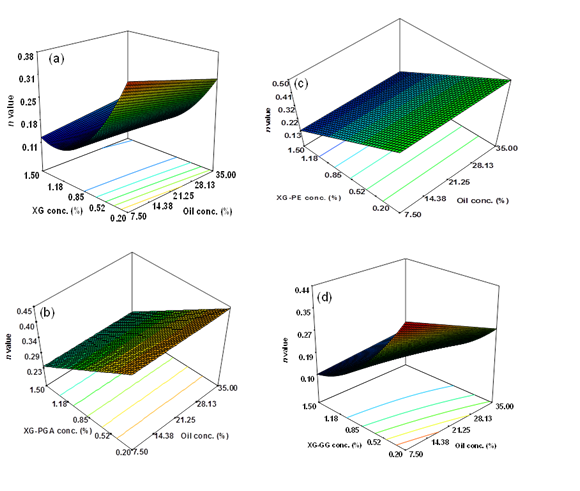
Figure 2 Response surface plots of power law model flow behavior index (n value) for dressings prepared with

1. Xanthan gum (XG),
2. Xanthan gum-propylene glycol alginate (XG-PGA),
3. Xanthan gum-pectin (XG-PE), and
4. Xanthan gum-guar gum (XG-GG).

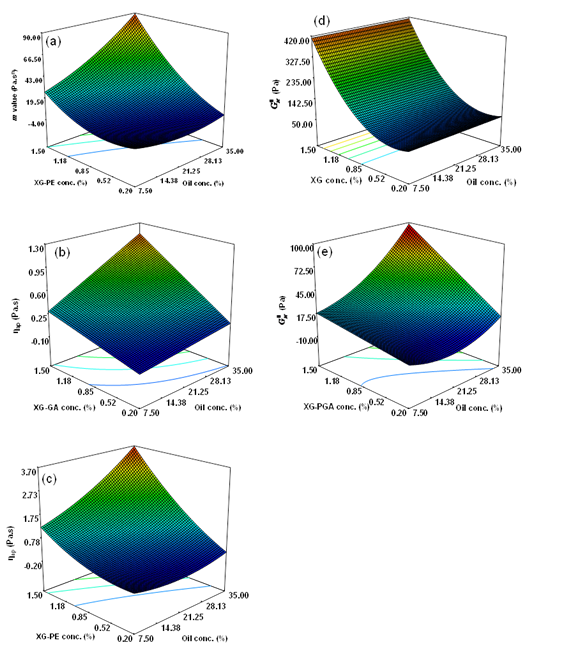
Figure 3 Response surface plots of

1. Power law model consistency coefficient (m value) for dressings prepared with xanthan gum-pectin (XG-PE).
2. Power law model apparent viscosity (ηap) obtained at shear rate of 46.16s-1 for dressings prepared xanthan gum-gum Arabic (XG-GA).
3. Power law model ηap for dressings prepared xanthan gum-pectin (XG-PE).
4. Plateau modulus ( $G_{N_{}}^0$ ) obtained during dynamic oscillatory testing for dressings prepared with xanthan gum (XG), (e) $G_{N_{}}^0$ for dressings prepared with xanthan gum-propylene glycol alginate (XG-PGA).

Regression models of the apparent viscosity (η_ap): The apparent viscosity (η_ap) at a shear rate of 46.16s^-1, which as reported is based on the shear rate of the perceived in-mouth thickness of normal fluids, was calculated according to the power law model.²⁸ Multiple regression equations with R²of 0.995, 0.855, 0.931, 0.994, and 0.998 for dressings prepared with XG, XG-GA, XG-PGA, XG-PE, and XG-GG are presented in Eq. 15, 16, 17, 18, and 19, respectively.

$Y_4=-0.23+0.0057x_1+0.449x_2+0.0366x_1{x}_2+0.623x_2{}^2$  (Eq. 15)

$Y_4=-0.152+0.0032x_1+0.151x_2+0.018x_1{x}_2$  (Eq. 16)

$Y_4=0.279-0.081x_1+0.675x_2+0.039x_1{x}_2+0.002x_2{}^2$  (Eq. 17)

$Y_4=0.623-0.0696x_1-0.97x_2+0.065x_1{x}_2+0.0014x_1{}^2+0.806x_2{}^2$  (Eq. 18)

$Y_4=0.916-0.0796x_1-2.452x_2+0.118x_1{x}_2+0.0015x_1{}^2+2.217x_2{}^2$  (Eq. 19)

All the response surface plots were similar to that shown in Figure 3B & Figure 3C, with an increase in gum(s) content and oil components yielding a linear (Figure 3B) or non-linear increase (Figure 3C) in ηap (Y₄). The results indicate that the perceived mouth feel thickness (i.e., ηap at 46.16s^-1) increased with increasing fat and hydrocolloid concentrations. The effect of oil on η_ap (Y₄) is in good agreement with the findings reported by Wendin et al.²⁹ specifically that an increase in fat content increased perceived thickness, fattiness, and toughness during sensory analysis. The flow behavior of emulsions is determined by the colloidal nature of the continuous phase as well as by the average particle size distribution.³⁰ An increase in oil (x₁) and hydrocolloid content (x₂) can lead to an increased degree of chain entanglement (i.e., hydrogen bonding with hydroxyl groups) and the distortion in the velocity pattern of the liquid by hydrated molecules of the solute in the emulsion system.^31,32 It is, thus, likely that as the gum concentration increased, larger numbers of high molecular weight molecules formed in the emulsion, increasing the resistance to flow and, therefore, the apparent viscosity of the emulsion.

Regression models of the plateau modulus ( $G_o^N$ ): Both the storage modulus (G′) and the loss modulus (G″), as measured during the dynamic oscillatory tests, were frequency-dependent and they both increased with increasing frequency (results not shown). G′ was significantly greater than G″ across the tested frequency range for all samples indicative of a predominantly elastic character. A plateau region observed in the oscillation curves at high frequencies may reflect a gel-like structure of a flocculated emulsion with the development of an entangled network.^25,33 The statistically significant second-order polynomial models with R² of 0.931 and 0.903 for dressings prepared with XG or XG-PGA are presented in Eq. 20 and 21, respectively.

$Y_5=96.826-216.49x_2+284.53x_2{}^2$  (Eq. 20)

$Y_5=19.643-3.219x_1-0.915x_2+1.843x_1{x}_2+0.078x_1{}^2$  (Eq. 21)

Figure 3D & 3E shows the three-dimensional response surface plots for the independent variables and their interactions with the predictive model for the plateau modulus ( $G_o^N$ ). $G_N^0$  (Y5) increased with an increase in gum content, especially for XG (Figure 3D) which showed significant increases at all oil concentrations within the experimental range studied. For the dressings prepared with XG-PGA, higher oil concentrations resulted in higher increases in $G_o^N$ (Figure 3E). $G_o^N$ is a measure of the intensity of the entangled network that develops between the adsorbed and non-adsorbed protein molecules.³³ The results are supported by the study of Raymundo et al.²⁵ & Gallegos et al.³⁴ who found that the $G_o^N$ values of mayonnaise increased with increasing xanthan gum and oil content in commercial mayonnaise and low-fat oil-in-water emulsions based on different formulations, due to an increase in both viscoelastic functions (i.e., G′ and G″).

Regression models of color parameters (L*, a*, and b* values) : The appearance of salad dressings is very important for consumer acceptability and color has a major impact on perceived appearance. The multiple regression models applied to the L* value (Y₆) with R² of 0.645, 0.696, 0.887, 0.866, and 0.980for dressings prepared with XG, XG-GA, XG-PGA, XG-PE, and XG-GG, respectively, are given by the following equations:

$Y_6=47.457+0.316x_1+7.584x_2$  (Eq. 22)

$Y_6=43.727+0.419x_1+6.452x_2$  (Eq. 23)

$Y_6=46.777+0.484x_1+5.753x_2$  (Eq. 24)

$Y_6=43.568+0.495x_1+7.147x_2$  (Eq. 25)

$Y_6=55.486+0.741x_1-5.343x_2+0.12x_1{x}_2-0.014x_1{}^2$  (Eq. 26)

In general, the dressings prepared with XG, XG-PGA, and XG-PE yielded response surface plots similar to that shown in Figure 4A for dressings with XG-GA. The positive coefficients for the two independent variables (x₁, x₂) in the equation (Eq. 22-25) indicate that the response variable Y₆ (L* value) increased with an increase in both variables (oil, x₁; and gum, x₂). In the case of dressings prepared with XG-GG, as observed in Eq. 26, both the oil (x₁) and gum (x₂) concentrations individually and the quadratic terms of x₁, as well as the interaction terms of x₁ and x₂,significantly influenced the lightness of the lentil supplemented dressings which explains the difference in the response surface plot (Figure 4B).

Figure 4C-G and Eq. (27-31) present the response surfaces and statistical models of a* values (Y7) for dressings prepared with XG, XG-GA, XG-PGA, XG-PE, and XG-GG, respectively.

$Y_{{}_7}=-0.982+0.009x_1+0.788x_2-0.442x_2{}^2$  (Eq. 27)

$Y_{{}_7}=-1.567+0.017x_1+1.084x_2-0.434x_2{}^2$  (Eq. 28)

$Y_{{}_7}=-0.941+0.048x_1-0.001x_1{}^2$  (Eq. 29)

$Y_{{}_7}=-1.860+0.067x_1+1.327x_2-0.0158x_1{x}_2-0.001x_1{}^2-0.453x_2{}^2$  (Eq. 30)

$Y_{{}_7}=-0.881+0.011x_1+0.463x_2-0.321x_2{}^2$  (Eq. 31)

In general, the a* value (Y7) increased with oil concentration for dressings with XG (Figure 4C), XG-GA (Figure 4D), XG-PE (Figure 4F), and XG-GG (Figure 4G). The effect of oil on Y₇ for the dressing with XG-PGA was quite different (Figure 4E) (i.e., the a* value increased with an increase in oil up to an oil content of 24.7% but decreased afterwards).

The effects of the oil and gum concentrations on b* values (Y8) are shown in Figure 5A & B. The multiple regression equations for dressings prepared with XG-PE and XG-GG are presented below (Eq. 32, and 33, respectively).

$Y_{{}_8}=1.372+0.354x_1+7.917x_2-0.152x_1{x}_2-0.004x_1{}^2-1.994x_2{}^2$ (Eq. 32)

$Y_8=7.131+0.18x_1-0.004x_1{}^2$ (Eq. 33)

The regression equations and the three-dimensional plots for ΔE values (Y₉) for dressings prepared with XG-PGA and XG-GG are presented in Eq. (34, 35) and Figure 5C & D respectively.

$Y_9=7.506+0.142x_1+1.782x_2$ (Eq. 34)

$Y_9=7.044+0.318x_1-0.005x_1{}^2$ (Eq. 35)

An increase in oil/gum concentration resulted in higher b* (Y₈) values at lower gum/oil content for dressings prepared with XG-PE (Figure 5A). The Y₈ values increased with an increase in oil up to an oil content of 21.25% but decreased afterwards for dressings prepared with XG-GG (Figure 5B). An increase in oil content generally yielded greater ΔE value (Y₉) for dressings with XG-PGA and XG-GG (Figure 5C & D).

Chroma, which represents the color intensity of samples, gives a better description of the spatial position of the measured color. Figure 5E-H and Eq. (36-39) present the response surfaces and multiple regression equations for chroma (Y₁₀) for dressings prepared with XG-GA, XG-PGA, XG-PE, and XG-GG, respectively.

$Y_{10}=11.986+0.136x_1$ (Eq. 36)

$Y_{10}=7.749+0.488x_1+1.704x_2-0.008x_1{}^2$ (Eq. 37)

$Y_{10}=10.366+0.109x_1+2.325x_2$ (Eq. 38)

$Y_{10}=7.19+0.177x_1-0.004x_1{}^2$ (Eq. 39)

As can be observed in Figure 5F,G chroma (Y₁₀) increased with oil (x₁) and gum (x₂) concentrations for dressings with XG-PE and XG-PGA, whereas for dressings prepared with XG-GA the response was solely dependent on oil content (Figure 5E). For dressings with XG-GG (Figure 5H), the effect of oil on chroma (Y₁₀) was different; it increased up to an oil content of 21.25% and then decreased.

Thus, the effect of the gums was complex and varied for different gums and combinations thereof. The color characteristics of an emulsion are the result of interactions between light waves and the emulsion. Color is generally governed by the unique composition and structure of food emulsions, and can be modified by the presence of droplets or other particulate matter.^35,36 As reported previously,^35,36 the blueness and greenness of an emulsion system are closely related to the droplet size and the droplet concentration of the emulsion system. Due to the interactions that occur between mixed gums and other ingredients, the conformation that the mixed gums adopt will depend on the particular environmental conditions. This may explain the variation observed in the generated three-dimensional plots and the regression models of color parameters for the dressings prepared with different gums.

Regression models of particle size parameters: The significant mathematical models (R2 of 0.935, 0.736, 0.816 and 0.941)of D[3, 2] for dressings prepared with XG, XG-GA, XG-PE, as well as XG-GG, are presented in Eq. 40, 41, 42, and 43, respectively.

$Y_{11}=23.952-0.405x_1-10.893x_2+4.214x_2{}^2$ (Eq. 40)

$Y_{11}=6.856+0.0664x_1+9.071x_2-0.374x_1{x}_2$ (Eq. 41)

$Y_{11}=10.659-0.062x_1+3.365x_2-0.21x_1{x}_2$ (Eq. 42)

$Y_{11}=6.847-0.407x_1+10.212x_2-0.287x_1{x}_2+0.01x_1{}^2$ (Eq. 43)

The response surface plots (Figure 6) show that an increase in gum concentration generally led to an increase in the Sauter mean diameter D[3,2] (Y11), especially at lower oil concentrations for XG-GA, XG-PE, and XG-GG (Figure 6B-D). Thus, larger droplets formed in response to increased gum concentrations at lower oil contents. A possible explanation for the observed trends is that the increased viscosity induced by the higher gum concentrations may have reduced the efficiency of the homogenization process and led to the formation of larger oil droplets. An increase in oil occurring at a higher gum content yielded lower values of D[3,2]for XG-GA, XG-PE, and XG-GG (Figure 6B-D), while an increased tendency of droplet size with oil content was observed at lower gum concentrations for dressings prepared with XG-PE (Figure 6C). The dressings prepared with XG showed a slightly increased D[3,2] (Y₁₁) with increasing oil concentration particular at higher gum contents, as shown in Figure 6A.

Figure 7A-E and Eq. (44-48) present the three-dimensional plots and multiple regression equations for the volume-weighted mean diameter D[4,3] (Y₁₂), with R² of 0.828, 0.745, 0.692, 0.952 and 0.819 for dressings prepared with XG, XG-GA, XG-PGA, XG-PE, and XG-GG, respectively.

$Y_{12}=205.89-4.67x_1$ (Eq. 44)

$Y_{12}=38.014-0.143x_1+90.634x_2-3.189x_1{x}_2$ (Eq. 45)

$Y_{12}=55.968-0.755x_1-35.443x_2+24.404x_2{}^2$ (Eq. 46)

$Y_{12}=41.362-0.446x_1+75.123x_2-2.071x_1{x}_2$ (Eq. 47)

$Y_{12}=28.865-29.085x_2+65.402x_2{}^2$ (Eq. 48)

In general, an increase in oil concentration led to a decrease in D[4,3](Y₁₂), except in the case of the dressing prepared with XG-GG (Figure 7E), which was dependent only on gum content, as evidenced by the single variable regression found between gum concentration (x₂) and the response Y₁₂ (Eq. 48).An increasing trend in D[4,3] (Y12) in response to increased gum content, especially at lower oil concentrations for XG-GA, XG-PGA, XG-PE was also observed in Figure 7.

The regression models generated for specific surface area (Y₁₃) with R² of 0.991, 0.988, 0.972, 0.988, and 0.839 for dressings prepared with XG, XG-GA, XG-PGA, XG-PE, XG-GG are presented in Eq. 49, 50, 51, 52, 53, respectively.

$Y_{13}=0.085-0.01x_1-0.058x_2+0.0068x_1{x}_2+0.00044x_1{}^2$ (Eq. 49)

$Y_{13}=0.07-0.001x_1-0.08x_2+0.0057x_1{x}_2+0.0002x_1{}^2$ (Eq. 50)

$Y_{13}=-0.03+0.0092x_1-0.043x_2+0.0058x_1{x}_2$ (Eq. 51)

$Y_{13}=8.617-0.588x_1-7.585x_2+0.687x_1{x}_2+0.03x_1{}^2$ (Eq. 52)

$Y_{13}=-0.058+0.013x_1$ (Eq. 53)

The response surface plots (not shown)for all five dressings showed an increase in response (Y13) with increasing oil (x₁) concentration, as well as an increased trend in Y13 with gum content (x₂) particularly at higher oil concentrations (x₁).

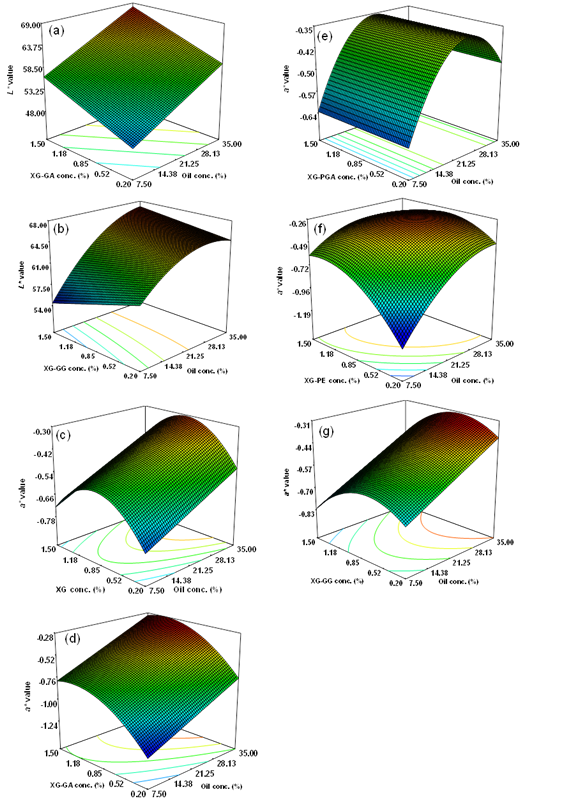
Figure 4 Response surface plots of

1. L* value for dressings prepared with xanthan gum-propylene glycol alginate (XG-PGA).
2. L* value for dressings prepared with xanthan gum-guar gum (XG-GG).
3. a* value for dressings prepared with xanthan gum (XG).
4. a* value for dressings prepared with xanthan gum-gum Arabic (XG-GA).
5. a* value for dressings prepared with xanthan gum-propylene glycol alginate (XG-PGA).
6. a* value for dressings prepared with xanthan gum-pectin (XG-PE).
7. a* value for dressings prepared with xanthan gum-guar gum (XG-GG).

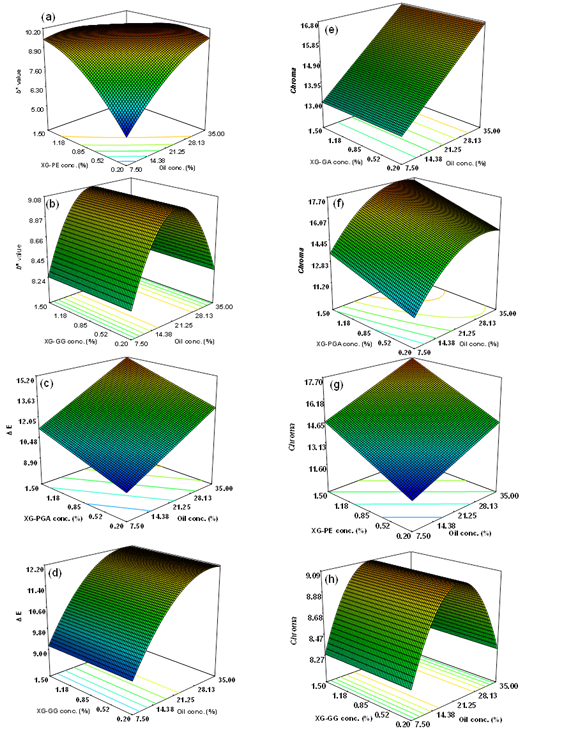
Figure 5 Response surface plots of

1. b* value for dressings prepared with xanthan gum-pectin (XG-PE).
2. b* value for dressings prepared with xanthan gum-guar gum (XG-GG).
3. ΔE value for dressings prepared with xanthan gum-propylene glycol alginate (XG-PGA).
4. ΔE value for dressings prepared with xanthan gum-guar gum (XG-GG).
5. chroma for dressings prepared with xanthan gum-gum Arabic (XG-GA).
6. chroma for dressings prepared with xanthan gum-propylene glycol alginate (XG-PGA).
7. chroma for dressings prepared with xanthan gum-pectin (XG-PE).
8. chroma for dressings prepared with xanthan gum-guar gum (XG-GG).

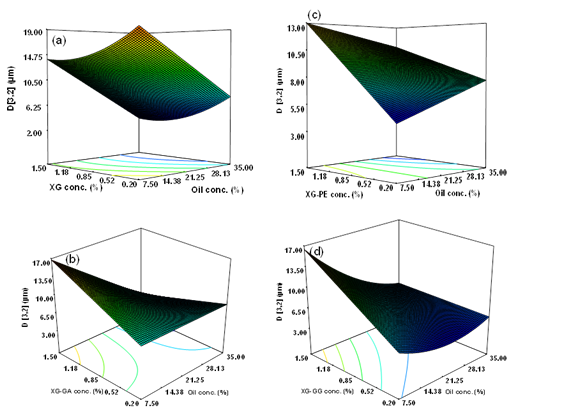
Figure 6 Response surface plots of Sauter diameter D[3,2] for dressings prepared with

1. Xanthan gum (XG).
2. Xanthan gum-gum Arabic (XG-GA).
3. Xanthan gum-pectin (XG-PE).
4. Xanthan gum-guar gum (XG-GG).

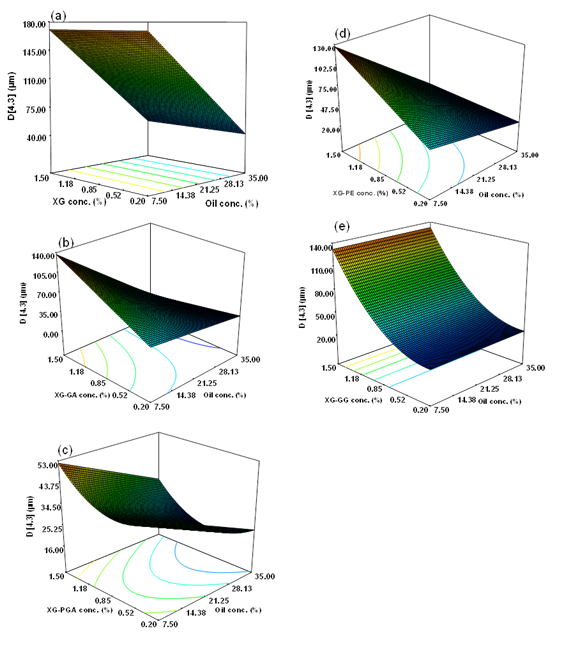
Figure 7 Response surface plots of volume-weighted mean diameter D[4,3] for dressings prepared with

1. Xanthan gum (XG).
2. Xanthan gum-gum Arabic (XG-GA).
3. Xanthan gum-propylene glycol alginate (XG-PGA).
4. Xanthan gum-pectin (XG-PE).
5. Xanthan gum-guar gum (XG-GG).

Comparison of different gums and gum combinations

Figure 8 compares the responses obtained at the center point of the experimental design (i.e., 21.25% oil and 0.85% gum). As can be seen in Figure 8A, the dressings prepared with XG and XG-GG exhibited the significantly highest m value (viscous nature), followed by XG-PE, and XG-PGA; the lowest m value was obtained for dressings prepared with XG-GA. The observation of the high viscous properties of XG and XG-GG were in accordance with their high pseudoplasticity, as discussed earlier. A previous study³⁷ on pure solutions of the hydrocolloids also reported that XG and GG showed the highest viscous properties (η΄) in a concentration-dependent manner. The synergistic effect of the two polysaccharides (GG and XG) has been reported extensively in earlier studies. Guar gum can change the helix-coil equilibrium of xanthan gum to a more flexible conformation for efficient binding.¹⁹ The highly extensive molecular structure and mechanical inflexibility of PGA, as well as potential entanglement with XG, may explain the relatively high viscosity of dressing emulsions formulated with XG-PGA observed in this study.

The dressing prepared with XG showed the highest (P<0.05) volume-weighted mean diameter (D[4,3]), whereas the XG-PGA combination had the lowest D[4,3]value (Figure 8A). For color, no major differences were observed for dressings prepared with the different gum combinations (Figure 8A) except for dressings prepared with XG-PGA and XG-PE which exhibited significantly higher a* value, indicative of a less greener hue (Figure 8B).

Dressings prepared with XG-GA and XG-PGA gave the highest flow behavior index (n) values (P<0.05), indicating that they had the lowest pseudoplasticity. In contrast, the dressings prepared with XG and XG-GG had the lowest n values (Figure 8B). This observation is in agreement with the findings of Pettitt et al.²¹ & Ahmed et al.⁶ who reported that the addition of GA and PGA to fixed levels of XG decreased pseudoplasticity, as evidenced by a significantly increased flow index (n). In terms of firmness (Figure 8B), dressings prepared with XG-GG gave the highest (P<0.05) values, followed by dressings with XG, while dressings formulated with XG-GA and XG-PGA had the lowest values (P<0.05). The dressing prepared with XG-PE had an intermediate value.

Figure 8 The variation of different responses including

1. consistency coefficient (m value), D[4,3], D[3,2], L* value, b* value, Delta E, Chroma, and
2. a*value, the flow behavior index (n value), firmness for dressings prepared with different gums based on the combination at the center point of the experimental design (21.25% of oil and 0.85% of gum). The results were the average values of six replications. For each parameter, mean values bearing different letters are significantly different (P<0.05) as per Tukey's multiple comparison test. In the graphic, XG: xanthan gum; XG-GA: xanthan gum-gum Arabic; XG-PGA: xanthan gum-propylene glycol alginate; XG-PE: xanthan gum-pectin; XG-GG: xanthan gum-guar gum.

Optimization and validation tests

A multiple-response optimization was applied within the experimental range of the independent variables studied (x₁ and x₂). For each response (Y) the mean values for tested commercial dressings were used as “target” as shown in Table 2. An optimum formulation for the composition of lentil supplemented dressing was obtained by superimposing all contour plots with the predicted equations of each response to yield the mean values for each independent variable (x) and the predicted values for each dependent variable (Y). A set of combinations of oil (x₁) and gum (x₂) concentrations was obtained as presented in Table 2.

Dressings were prepared using the optimized formulations and the responses for these were tested. The adequacy of the response surface models was evaluated by comparing the responses for the predicted values and the experimental values. As can be seen in Table 2, the response values obtained from the validation tests were quite similar to the predicted values for dressing with each gum, except for the experimental values for D[3,2] and D[4,3]which were generally higher than the predicted values. In addition, for dressings prepared with XG-PE, the consistency coefficients (m value) obtained in the validation tests were different from the predicted ones; however, they were very similar to the targeted values. In general, the results showed that the regression models employed to predict the physical properties of the lentil-supplemented salad dressing emulsions were adequate.

The validation test showed the adequacy of the models used in predicting dressing behavior and further demonstrated that stable lentil flour-supplemented salad dressings could be prepared with a variety of gum blends and using different oil concentrations. Perhaps, the most interesting aspect of the study is that the dressings developed had physical properties similar to those of the commercial dressings studied. Overall, the study provides timely and useful information for predicting the textural, rheological, color and particle size characteristics of emulsions that could be used in the development of novel lentil flour-supplemented salad dressings. Studies on sensory evaluation of such products would be of interest for future work. As there are several types of pulses that can be similarly used in salad dressing preparations, the study further provides a model approach that could be translated to determine ideal conditions for the preparation of other pulse-supplemented salad dressings.

This study was funded by the Agriculture and Agri-Food Canada Agricultural Bio-product Innovation Program. Financial support from the Chinese Government Scholarship Program is also gratefully acknowledged. We also thank the Canadian International Grains Institute (Winnipeg, MB, Canada) for supplying the lentil flour used for the study.

The author declares no conflict of interest.

1. Berrios JJ. Extrusion cooking of legumes: Dry bean flours. Encyclopedia of Agricultural Food and Biological Engineering 2nd ed. 2006;1:1–8.
2. Petitot M, Boyer L, Minier C, et al. Fortification of pasta with split pea and faba bean flours: Pasta processing and quality evaluation. Food Res Int. 2010;43(2):634–641.
3. Sosulski FW, Wu KK. High-fiber breads containing field pea hulls, wheat, corn, and wild oat brans. Cereal Chem. 1998;65(3):186–191.
4. Zare F, Boye JI, Orsat V, et al. Microbial, physical and sensory properties of yogurt supplemented with lentil flour. Food Res Int. 2011;44(8):2482–2488.
5. Dickinson E, Stainsby G. Advances in food emulsions and foams. Barking, Essex: Elsevier Applied Science Publishers Ltd; 1988.
6. Ahmed J, Ramaswamy HS, Ngadi MO. Rheological characteristics of Arabic gum in combination with guar and xanthan gum using response surface methodology: effect of temperature and concentration. Int J Food Prop. 2005;8(2):179–192.
7. Cairns P, Miles MJ, Morris VJ, et al. X-ray fibre-diffraction studies of synergistic, binary polysaccharide gels. Carbohydr Res. 1987;160:411–423.
8. Williams PA, Phillips GO. Gum arabic. In: GO Phillips, et a. editors. Handbook of Hydrocolloids. (Chapter 11). Boca Raton, UK: CRC press; 2009.
9. Parker A, Gunning PA, Ng K, et al. How does xanthan stabilise salad dressing? Food hydrocolloids. 1995;9:333–342.
10. Fennema OW. Food chemistry. New York, USA: Marcel Dekker Inc; 1985.
11. Akiyama Y, Eda S, Kato K. Gum arabic is a kind of arabinogalactan-protein. Agric Biol Chem. 1984;48(1):235–237.
12. Pettitt DJ, Wayne JOEB, Nantz JJR, et al. Rheological properties of solutions and emulsions stabilized with xanthan gum and propylene glycol alginate. J Food Sci. 1995;60(3):528–531.
13. Yilmazer G, Carrillo ANAR, Kokini JL. Effect of propylene glycol alginate and xanthan gum on stability of O/W emulsions. J Food Sci. 1991;56(2):513–517.
14. Thakur BR, Singh RK, Handa AK, et al. Chemistry and uses of pectin-a review. Crit Rev Food Sci Nutr. 1997;37(1):47–73.
15. Guimarães GC, Coelho Júnior MC, et al. Density and kinematic viscosity of pectin aqueous solution. J Chem Eng Data. 2008;54(2):662–667.
16. McCleary BV, Amado R, Waibel R, et al. Effect of galactose content on the solution and interaction properties of guar and carob galactomannans. Carbohydr Res. 1981;92(2):269–285.
17. Casas JA, Mohedano AF, García-Ochoa F. Viscosity of guar gum and xanthan/guar gum mixture solutions. J Sci Food Agric. 200;80(12):1722–1727.
18. Tako M, Nakamura S. Synergistic interaction between xanthan and guar gum. Carbohydr Res. 1985;138(2):207–213.
19. Wang F, Wang YJ, Sun Z. Conformational role of xanthan gum in its interaction with guar gum. J Food Sci. 2002;67(9):3289–3294.
20. Paraskevopoulou D, Boskou D, Paraskevopoulou A. Oxidative stability of olive oil-lemon juice salad dressings stabilized with polysaccharides. Food Chem. 2007;101(3):1197–1204.
21. Jamison JD, Towle GA, Vermeychuk JG. Pourable salad dressing composition. US patent No 4129663; 1978.
22. Ballard BF, Schweid JM, Dec AF. Dry mix for low-oil salad dressing. US patent No 4596715A; 1986.
23. Worrasinchai S, Suphantharika M, Pinjai S, et al. bet]-Glucan prepared from spent brewer's yeast as a fat replacer in mayonnaise. Food Hydrocolloids. 2006;20(1):68–78.
24. Myers RH, Montgomery DC, Anderson-Cook CM. Response surface methodology: process and product optimization using designed experiments. New York, USA: John Wiley & Sons Inc; 2009. 704 p.
25. Raymundo A, Franco JM, Empis J, et al. Optimization of the composition of low-fat oil-in-water emulsions stabilized by white lupin protein. J Am Oil Chem Soc. 2002;79(8):783–790.
26. Szczesniak AS, Farkas E. Objective characterization of the mouthfeel of gum solutions. J Food Sci. 1962;27:381–s385.
27. Marcotte M, Taherian Hoshahili AR, Ramaswamy HS. Rheological properties of selected hydrocolloids as a function of concentration and temperature. Food Res Int. 2001;34(8):695–703.
28. Baines Z, Morris E. Effects of polysaccharide thickeners on organoleptic attributes. In: GO Phillips, et al.editors.Gums and Stabilisers for the Food Industry 4. Oxford, UK: IRL Press; 1988. p.192–201.
29. Wendin K, Aaby K, Edris A, et al. Low-fat mayonnaise: Influences of fat content, aroma compounds and thickeners. Food hydrocolloids. 1997;11(1):87–99.
30. Coia KA, Stauffer KR. Shelf life study of oil/water emulsions using various commercial hydrocolloids. J Food Sci. 1987;52(1):166–172.
31. Azoubel PM, Cipriani DC, El-Aouar ÂA, et al. Effect of concentration on the physical properties of cashew juice. J Food Eng. 2005;66(4):413–417.
32. Zhang S, Li FX, Yu JY. Rheological properties of cellulose-NaOH complex solutions: From dilute to concentrated states. Cellul Chem Technol. 2011;45(5-6):313–320.
33. Franco JM, Berjano M, Gallegos C. Linear viscoelasticity of salad dressing emulsions. J Agric Food Chem. 1997;45(3):713–719.
34. Gallegos C, Berjano M, Choplin L. Linear viscoelastic behavior of commercial and model mayonnaise. J Rheol. 1992;36(3):465–478.
35. Chantrapornchai W, Clydesdale F, McClements DJ. Influence of droplet size and concentration on the color of oil-in-water emulsions. J Agric Food Chem. 1998;46(8):2914–2920.
36. McClements DJ. Food emulsions principles, practices, and techniques. 2nd ed. Boca Raton, UK: CRC Press; 2005. 632 p.
37. Yaseen EI, Herald TJ, Aramouni FM, et al. Rheological properties of selected gum solutions. Food Res Int. 2005;38(2):111–119.
38. Paraskevopoulou D, Boskou D, Paraskevopoulou A. Oxidative stability of olive oil–lemon juice salad dressings stabilized with polysaccharides. Food Chem. 2007;101(3):1197–1204.