The solubility of cholesterol in methoxyethanol, ethoxyethanol and butoxy ethanol was measured by a gravimetrical method from (298.15 to 318.15)K at atmospheric pressure. It is observed that solubility increases with increase in temperature. The mole fraction solubility was found to be higher in butoxyethanol and minimum in methoxyethanol. The correlation coefficient in methoxyethanol, ethoxyethanol and butoxyethanol were observed in the range 0.994-0.997. The experimental solubility data were correlated with temperature by modified Apelblat and Buchowski-Ksiazczak λh equations. It is observed that the theoretical values evaluated by these equations are in good agreement with the experimental solubility values. Further, some thermodynamic parameters such as Gibb’s free energy, enthalpy and entropy of dissolution were evaluated from solubility data. It is observed that the evaluated thermodynamic parameters are negative. The negative enthalpy and Gibb’s free energy suggest exothermic and spontaneous dissolution of cholesterol in all the three glycol ethers whereas negative entropy indicates that dissolution causes more ordered structure in these solvents.

Keywords: cholesterol, solubility, glycol ethers, apelblat equation, buchowski-ksiazczak λh equation, thermodynamic parameters

Cholesterol (Chemical Name: Cholest-5-en-3b-ol) is a sterol and a lipid found in the cell membranes of all body tissues, and transported in the blood plasma of all animals. Small amount of cholesterol is also found in plant membranes. It is also required to build and maintain cell membranes and act as an antioxidant.¹ It has been reported^2,3 that due to its presence in cell membrane, it participates in various types of interactions such as hydrogen bonding, Van der Waals, dipole-dipole etc. The hydrogen bonding and Vander Waals interactions are important for the cholesterol biological functions. Further, cholesterol is the major component of most gallstones and it is sparingly soluble in water.^4,5

In the present work, the solubilities of cholesterol in some glycol ethers, such as methoxyethanol ethoxyethanol and butoxyethanol have been measured from (298.15 to 318.15)K at atmospheric pressure. The Apelblat and Buchowski-Ksiazczak λh equations were used for correlation of experimental solubility data with temperature. Using these solubility data, some thermodynamic parameters have also been evaluated.

Materials

Cholesterol, with a mass fraction purity of 99.5%, was purchased from HiMedia Pvt. Ltd. (Mumbai, India). All the solvents, i.e., methoxyethanol, ethoxyethanol and butoxyethanol selected for the present study were analytical grade reagents which were purified by fractional distillation. Their purities were checked by SHIMADZU GC-MS (Model No QP-2010) and were found to be greater than 99.80% (Figure 1).

Figure 1 Structure of Cholesterol.

Cholesterol was recrystallized and its melting temperature was determined using Differential Scanning Calorimeter (DSC) (Model-Shimadzu-DSC-60). The observed value was found to be 149°C which is in good agreement with the reported value of 149.8°C.⁶ The purity of solvents was checked by GC-MS (SHIMADZU Model No.-QP-2010) and found to be greater than 99.0%.

Apparatus and procedure

The solubility was measured by gravimetric method in methoxyethanol, ethoxyethanol and butoxyethanol from (298.15 to 318.15)K at atmospheric pressure of 0.1MPa. For each measurement, an excess amount of cholesterol was added to a known amount of solvent in an equilibrium cell, which was heated to a constant temperature with continuous stirring. After, at least 3 h (the temperature of the water bath approached constant value, then the actual value of the temperature was recorded), the stirring was stopped and the solution was kept still for 2h. A portion of this solution was filtered and by a preheated injector, 2ml of this clear solution was taken in another weighted measuring vial. The vial was quickly and tightly closed and weighted to determine the mass of the sample. Then, the vial was covered with a piece of filter paper to prevent dust contamination and placed at room temperature to evaporate the solvent. After the solvent in the vial had completely evaporated, the vial was dried and reweighed to determine the mass of the constant residue solid. All the masses were taken using an electronic balance (Mettler Toledo AB204-S, Switzerland) with an uncertainty of ±0.0001g. Thus, the solid concentration of the sample solution of mole fraction, x, could be determined from eq 1.

$x=\frac{m_2/M_2}{m_1/M_1+m_2/M_2}$ (1)

where m₁ and m₂ represent the masses of pure solvent and cholesterol respectively. M₁ and M₂ are the molecular weights of pure solvent and cholesterol respectively. At each temperature, the measurement was repeated three times and an average value is given in Table 1 along with uncertainty.

The experimental mole fraction solubilities x of cholesterol in methoxyethanol, ethoxyethanol and butoxyethanol at different temperatures (298.15 to 318.15K) are summarized in Table 1. The variation of solubility with temperature is also shown in Figure 2. It is observed that solubility increases linearly with increase in temperature. Further, solubility is higher in butoxyethanol and minimum in methoxyethanol. Thus, solubility increases as number of carbon increases. The solubility in these three glycol ethers can also be related to their dielectric constants and dipole moments⁷ which are given in Table 2. It is observed that dielectric constant is maximum for methoxyethanol in which solubility is minimum. Whereas for butoxyethanol, dielectric constant is minimum and solubility is maximum. Thus in the present study, solubility is reverse of dielectric constant. The dipole moment of all the three solvents is not much different and no concrete relation between solubility and dipole moment can be decided. Further, the increase of chain length causes a decrease in acidity and polarity of molecules and an increase in basicity of hydroxyl oxygen. Cholesterol has ability for self-association and acts as proton donor in the association process with proton acceptors as reported by Garalski.² So, it readily associates with the selected solvents and association increases with increase in chain length. This association takes place mainly by hydrogen bonding. Also, the growing number of carbon atoms causes a decrease in self association i.e., solvent-solvent interactions, which can be attributed to the presence of intermolecular hydrogen bonds. This may results in an increase in solubility of cholesterol in butoxyethanol.

Figure 2 Variation of mole fraction solubilities (x) with temperature for cholesterol in glycol ethers.•: methoxyethanol; ▲: ethoxyethanol; ∎: butoxyethanol. Corresponding dotted (­-----) lines are for calculated solubility by Apelblat equation.

The temperature dependence of cholesterol solubility in solvents is described by modified Apelblat equation:^8,9

$ln{x}_i^a=A+B/T+C ln(T)$ (2)

where x^a_ci is the mole fraction solubility calculated by equation 2 and T is the absolute temperature. A, B and C are the empirical model parameters determined by least square method and the values are given in Table 3. The values of A and B represent the variation in the solution activity and the solution behavior resulting from the non idealities on the solubility of solute, and the value of C represents the association between the temperatures and the enthalpy of fusion.

The solubility is also correlated with temperature by Buchowski–Ksiazczak λh equation^10,11 which describes the solid-liquid equilibrium behavior by only two adjustable parameters λ and h. The Buchowski equation can be written as:

$\begin{array}{l}\ln \left(1+\frac{\lambda (1-{{\displaystyle x}}_{ci}^b)}{{{\displaystyle x}}_{ci}^b}\right)=\lambda h\left[\frac{1}{(T/K)}-\frac{1}{(T_m/K)}\right]\\ \ln \left(1+\lambda \left(1-x\right)\right)\end{array}$ (3)

where x^b_ci is calculated mole fraction solubility by equation 3. T and T_m are experimental temperature and melting temperature of compound in K. λ and h are the parameters of Buchowski-Ksiazczak λh model which are given in Table 4.

The solubility of compounds calculated by Apelblat equation (x^a_ci) and Buchowski-Ksiazczak λh equation (x^b_ci) are also given in Table 1 and Figure 2, Figure 3. It is observed from the Table 1 and Figure 2, Figure 3 that solubility of compound increases nonlinearly with increasing temperature. Further, it is shown from the Table 1 that the values obtained by modified Apelblat equation and Buchowski-Ksiazczak λh equation shows good agreement with each other and also with experimental solubility data.

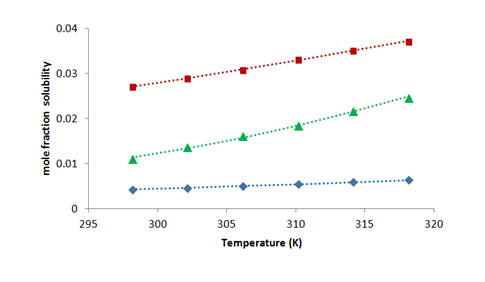
Figure 3 Variation of mole fraction solubilities (x) with temperature for cholesterol in glycol ethers.•: methoxyethanol; ▲: ethoxyethanol; ∎: butoxyethanol. Corresponding dotted (­-----) lines are for calculated solubility by Buchowski equation.

The relative deviations (RD), root-mean-square deviations (RMSD) and relative average deviations (ARD) between the experimental and calculated solubility are evaluated for both modified Apelblat and λh equations by the following equations:

$RD=\raisebox{1ex}{$\left(x_i-x_{ci}^{a/b}\right)$}\!\left/ \!\raisebox{-1ex}{${{\displaystyle x}}_i$}\right.$ (4)

$RMSD={\left[{\displaystyle \sum _{i=1}^N\raisebox{1ex}{${{\displaystyle ({{\displaystyle x}}_{ci}-xi)}}^2$}\!\left/ \!\raisebox{-1ex}{$N-1$}\right.}\right]}^{1/2}$ (5)

$ARD=\frac{1}{N}{\displaystyle \sum _i^N\raisebox{1ex}{$(xi-{{\displaystyle xc}}_i)$}\!\left/ \!\raisebox{-1ex}{$xi$}\right.}$ (6)

where N is the number of experimental points. All these evaluated values are given Table 3, Table 4 & Table 5. It is evident from these Tables that the results evaluated by modified Apelblat equation and λh equations are in good agreement with experimental solubility.

The dissolution of compound in a solvent is associated with changes in thermodynamic functions such as enthalpy (DH_sol), Gibb’s energy (ΔG_sol) and entropy of solution (DS_sol).

The enthalpy of solution (DH_sol) was calculated by the following modified Van’t Hoff equation:

${{\displaystyle \left(\raisebox{1ex}{$\partial \ln xi$}\!\left/ \!\raisebox{-1ex}{$\partial \left(\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$T$}\right.-\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$T_{hm}$}\right.\right)$}\right.\right)}}_P=-\raisebox{1ex}{$\Delta {{\displaystyle H}}_{sol}$}\!\left/ \!\raisebox{-1ex}{$R$}\right.$ (7)

where T is the experimental temperature, R is universal gas constant (8.314 J/mol K) and T_m is mean harmonic temperature¹² which is calculated by equation:

$T_{hm}=\raisebox{1ex}{$n$}\!\left/ \!\raisebox{-1ex}{${\displaystyle {\sum }_{i=1}^n\left(\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$T$}\right.\right)}$}\right.$ (8)

where n is the number of experimental temperatures studied. In the present study, value of T_hm calculated by this equation is found to be 307.998K.

The slope of the plot of lnx versus (1/T - 1/T_hm) gives the enthalpy of solution (DH_sol) whereas Gibb’s free energy is calculated by intercept using the following relation:

$\Delta G_{sol}=-R\times T_{hm}\times Intercept$ (9)

Finally, the entropy of solution (DS_sol) was obtained from these evaluated DH_sol and DG_sol values at T_hm.^13,14

$\Delta S_{sol}=\frac{\Delta H_{sol}-\Delta G_{sol}}{T_{hm}}$ (10)

These evaluated thermodynamic parameters are given in Table 6.

It is observed from Table 6 that all the three thermodynamic parameters i.e., DH_sol, DG_sol and DS_sol are negative for all the three solvents.

Comparison of solubility data with Gibb’s energy values shows that these two are inversely related. The ΔG_sol is more negative in buthoxyethanol which further suggests more spontaneous dissolution than in methoxyethanol and ethoxyethanol. The negative enthalpy of dissolution (DH_sol) indicates exothermic dissolution process whereas negative entropy is due to more order in solutions.¹⁵

Solubility of cholesterol increases linearly with increase in temperature and solubility is higher in butoxyethanol. The solubility data calculated by modified Apelblat and λh equations are in good agreement with experimental values. Thermodynamic parameters suggest that dissolution of cholesterol in studied glycol ethers is spontaneous and exothermic. Further, solutions of cholesterol in these solvents are more ordered.

None.

Author declares that there is no conflict of interest.

1. Smith LL. Another cholesterol hypothesis: Cholesterol as antioxidant. Free Radical Biology and Medicine. 1991;11(1):47–61.
2. Garalski P. A calorimetric study of cholesterol dissolved in an alcohol—the solute–solvent interaction. The Journal of Chemical Thermodynamics. 1993;25(3):367–371.
3. Krause MR, Regen SL. The Structural Role of Cholesterol in Cell Membranes: From Condensed Bilayers to Lipid Rafts. Acc Chem Res. 2014;47(12):3512–3521.
4. Carey CM, Small MD. The physical chemistry of cholesterol solubility in bile. Relationship to gallstone formation and dissolution in man. JCI. 1978;61(4):998–1026.
5. Atamanalp SS, Keles MS, Atamanalp RS, et al. The effects of serum cholesterol, LDL, and HDL levels on gallstone cholesterol concentration. Pak J Med Sci. 2013;29(1):187–190.
6. Williams JH, Kuchmak M, Witter RF, et al. Purity of cholesterol to be used as a primary standard. J Lipid Res. 1965;6(4): 461– 465.
7. WM Haynes, editor. CRC Handbook of Chemistry and Physics. 91st ed. UK: CRC press Boka Raton; 2010.
8. Apelblat A, Manzurola E. Solubilities ofo-acetylsalicylic, 4-aminosalicylic, 3,5-dinitrosalicylic, andp-toluic acid, and magnesium-DL-aspartate in water fromT=(278 to 348) K. The Journal of Chemical Thermodynamics. 1999;31(1):85–91.
9. Gao J, Wang ZW, Xu DM, et al. Solubilities of Triphenylphosphine in Ethanol, 2-Propanol, Acetone, Benzene, and Toluene. J Chem Eng Data. 2007;52(1):189–191.
10. Buchowski H, Ksiazczak A, Pietrzyk S, et al. Solvent activity along a saturation line and solubility of hydrogen-bonding solids. J Phys Chem. 1980;84(9):975–979.
11. Ksiazczak A, Moorthi K, Nagata I, et al. Solid-solid transition and solubility of even n-alkanes. Fluid Phase Equilibria. 1994;95:15–29.
12. Krug RR, Hunter WG, Grieger RA, et al. Enthalpy-entropy compensation. 2. Separation of the chemical from the statistical effect. J Phys Chem. 1976;80:2341–2351.
13. Bustamante SP, Romero AP, Escalera B, et al. Enthalpy–entropy compensation for the solubility of drugs in solvent mixtures: Paracetamol, acetanilide, and nalidixic acid in dioxane–water. Journal of Pharmaceutical Sciences. 1998;87(12):1590–1596.
14. Meng Z, Hu Y, Kai Y, et al. Investigation on vapor–liquid equilibria for binary systems of metal ion-containing ionic liquid [bmim]Zn2Cl5/NH3 by experiment and modified UNIFAC model. Fluid Phase Equilibria. 2013;352:1–6.
15. El–Bindary A, El–Sonbati AZ, El–Mosalamy EH, et al. Solvent Extraction of Microamounts of Cesium from Water into Nitrobenzene by Using Hydrogen Dicarbollylcobaltate in the Presence of Dicyclohexyl-18-crown-6. Chemical Papers. 2003;57(4):255–258.