Filtration models were applied to a gravel inlet system to estimate attachment and/or detachment of particles onto collectors (gravel grain). Two methods were used to estimate total solidstrapping efficiency at the gravel inlet: mass concentration and particle count. The first method provided trapping estimate between 11% and 22% based on two averaging computations. The second method, particle count, showed that detachment of total solids occurred mostly with the clay size category and early duringrainfall events. Detachment reveals the quality of effluent and can be interpreted as particles being detached either from previous total solids deposit or not being retained by the collector. Based on a model by Rajagopalan and Tien, trapping ability of gravel inlet was expected to be relatively low (<50%) for particles and aggregates smaller than 100 μm. Five rainfall events in 2002 were analyzed and showed that the first event had a retention capacity of 32% with a significant statistical difference between pairs of samples from “above” and “below” the gravel, based on a paired t-test. The following rainfall events had not seen any significant difference based on the same statistical test between the above and below water samples; however, the pattern of retention within pairs of samples showed that large filtration values were associated with incoming large solids concentrations, which, in turn, are related to rainfall bursts. The laser diffractometer technique allowed the particle count method to estimate number of particles retained or detached with respect to the gravel media. Particle count was obtained by direct measurement in the fine silt and clay size region and by extrapolation of measured data for large size in the silt-sand region including small particles and aggregates. Two rainfall events (August 3 and 21) showed important detachment based on particle counting method.

Keywords: gravel inlet system, Rajagopalan and Tien filtration model, attachmentand detachment, trapping efficiency, mass concentration method, particle count method

Filtration is an operation intended 1/ to remove particles in the influent suspension passing through a porous (or granular) media to obtain a lower concentration of effluent particles and 2/ to monitor the performance of the filter over time with respect to deposition or detachment of particles. Influent and effluent are here defined as the incoming flow and exiting flow through the filter media, respectively. Filtration depends on several factors such as the carrier fluid (flow rate, viscosity, density), particle suspension (concentration, size, shape of particles), and the porous medium filter (porosity, diameter of pores, size and shape of grains, retention capacity).^1,2 In filtration literature, filter grain or media grain (sand, coal, gravel, and other materials) is also referred to as the collector, and thus these terms are used alternatively through the rest of the exposition.

Two research projects closely related to this gravel inlet investigation were conducted by other research workers in Minnesota: Gieseke³ and Oveson.⁴ The first project was a direct comparison of surface inlet and gravel inlet on two adjacent watersheds in Carver County, Minnesota. The second project dealt with an evaluation of gravel media in an inlet system for a laboratory setup.

An open inlet and a gravel inlet were installed to compare losses pollutants from two adjacent basins in the Chaska Creek Watershed.³ The newly installed gravel inlet had an infiltration rate of more than 3.11cm s^-1; however, the same gravel inlet had a lower infiltration rate (0.57cm s^-1) in a 2 year-period, which corresponds to 82% reduction of infiltration rate. On a per hectare basis, gravel inlet reduced total solids and ortho-phosphate loading by 88% and 64%, respectively, compared to open surface inlet. A simulated runoff event was monitored and showed that the gravel inlet was effective in reducing total solids load by 98% and total phosphorus load by 69%.

A laboratory experiment was undertaken to evaluate trapping efficiency of gravel inlets using several parameters, among which were gravel media size, sediment size, and sediment loading rate.⁴ Two models were developed 1/ to predict reduction of gravel inlet permeability with sediment loading and 2/ to estimate average trapping efficiency of gravel inlets. The first parameter in the investigation was gravel media size. The median particle diameters (d₅₀) were 16.59 mm, 13.60 mm, 5.06 mm. Clean water permeability for the gravel ranged between 1.2 cm sec^-1 and 8.7 cm sec^-1 and gravel porosity varied from 35.7% to 41.0 %. The second parameter was sediment particle size while the third parameter in the investigation was loading rate of sediment. An experiment run was discontinued when rock core permeability, K, was less than 10% that of the clean water value, K_c. No change in permeability was observed with clay or sand used in water suspension across the three gravel sizes. Silt, however, reduced permeability down to the criterion value (K/K_c<10%) with loading rate of 0.18 kg min^-1 and 0.46 kg min^-1.Sediment mass deposited ranged between 5 kg and 65 kg for silt size sediment.

Low accumulation in filtration process refers to the absence of flocculation treatment of the effluent suspension before entering the porous media. It is then assumed that no chemical reaction leading to aggregation of particles (particle-particle interactions) is occurring. The following exposition aims at analyzing a field setup and its recorded events with collected water samples to assess the filtration capacity of gravel inlets.

There are two major mechanisms in filtration, which are straining and attachment.⁵ In straining process, particle sizes to be filtered are greater than the collector opening (interstitial distance between adjacent grains or mesh size of filter paper). Particles are literally deposited on the collector surface and can be mechanically removed later. This is equivalent to coffee filtration in everyday application. On the other hand, attachment process involves both the transport of particles by the fluid and their capture unto the collector surface. Particles are many times smaller than the size of the collector pores through which they move with the fluid. The relative dimensions of particles diameter, collector diameter, and pore sizes become important in the attachment process (Figure 1). As an example, colloid particle sizes range between 0.01 to 10μm, effective size of sand used in filtration is approximately 500μm, pore dimensions in the sand range from 35-50μm.⁶ Straining mechanism is thus excluded in water suspension filtration. Instead, gravity, surface, hydrodynamic, and chemical forces are involved in capturing the particle to attach unto the collector surface.

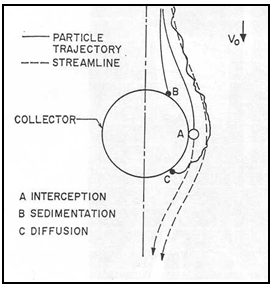
Figure 1 Model of attachment processes as sedimentation (gravitation), diffusion, interception.²⁹

Herzig et al.¹ and Jegatheesan and Vigneswaran⁷ provided an overview of possible mechanisms of attachment or detachment of particles on or from the collector as well as the sites of such reactions. Possible retention sites on collector are surface, crevice, and cavern. Retention forces that attach particles on those sites are axial pressures of fluid (fluid pressure holding a particle against the surface), friction forces, surface forces (Van der Waals and electrostatic or electrokinetic), and chemical forces (any possible chemical bonding). Capture mechanisms onto the collector’s surface (or attachment) include sedimentation, direct interception, and diffusion by Brownian motion.⁸ Sedimentation is a major component of capture process owing to the difference in density between the fluid and particles; particles are subjected to gravity and associated settling velocities, and thus may be deposited on the collector surface. Direct interception is considered as a boundary condition of attachment in the theoretical treatment of sedimentation and diffusion.⁹ Brownian motions account for diffusion process, which is stochastic in nature, and is more important for particles less than 1 μm for attaching to collector surfaces. Particles deposited on the collector’s surface act as additional collector and can increase removal of incoming particle from the flow.

Rajagopalan and Tien Filtration Model (R&T)

A review of filtration equations by Logan et al.¹⁰ compared several filtration models and showed that the model built by Rajagopalan and Tien¹¹ gave the closest values to experimental data and allowed the use of sticking coefficient close to 1 (range: 0.46 – 1.1). It was also consistent with necessary assumptions for solution derivation. The approach is to base removal of particles on restricted flow around concentric spherical spaces surrounding the collectors. This setup considers the neighborhood of collector grains, thus more realistic. One of the key parameter in the models was the calculation of single collector collision efficiency termed “collector efficiency” or “collision efficiency “in the remaining text; collector efficiency is a combination of dimensionless numbers (or terms) representing diffusion, London and van der Waals forces, gravitation, and interception.

The model is based on the integration of flow trajectory over one collector and on the presence of neighboring collectors.¹² This equation computes the ratio of effluent to influent concentration (Table 1). The percentage of incoming particle retained in the filter medium is the complement to 1.0 of this ratio. Based on the form of the equation, the value of this ratio is inversely proportional to collector size. This relationship implies that large collector will have less filtering capacity compared to small collector. Also, the ratio is directly proportional to the collector efficiency, the attachment coefficient, and the physical depth of the filter. The major parameters in this equation are collector size, porosity of the collector media, collector efficiency, the depth of the filter media, and the attachment coefficient. The equation was solved for four collector sizes: 1.36 cm, 0.85 cm, 0.50 cm, and 0.10 cm. The collector size of 1.36 cm and 0.50 cm came from Oveson’s laboratory experiment; gravel of 0.85 cm average diameter comes from the field experiment set in Le Sueur County. The size of 0.10 cm for collector was introduced in the simulation to evaluate the effect of small size gravel in the simulation.

Based on the formulation of the four dimensionless numbers in the collector efficiency, two of them are related to collector size (gravel) while the other two are independent of collector size. Peclet and interception numbers are dependent on collector size and their values are different for each of the four collector diameters listed previously. Peclet number expresses the random movement of sub-micron particles in a moving fluid and is computed as the ratio of inertia forces to diffusion forces. This number increases with particle size based on its formulation; however, its contribution to the collector efficiency number is an inverse of the value obtained in the formulation, thus contribution of Peclet number is comparatively greater with small particles in the collector efficiency computation. Interception dimensionless number is the ratio of particle size to collector size. Large particles have more opportunity to be intercepted by the collectors and be retained on their surface.

The other two dimensionless numbers that are not function of collector size are those of London-van der Waals and of gravitation. London-van der Waals force stems from the fluctuation of electron clouds surrounding the nucleus of electrically neutral atom. This force is attractive in nature and operates over a short distance. Its magnitude decreases rapidly away from the surface. The interaction energy between a sphere (modeling a moving particle in the fluid) and a surface (modeling the collector’s surface) is expressed as a product of the Hamaker constant and the sphere diameter divided by the distance of the sphere from the surface. Thus, the interaction energy becomes infinite as the particle approaches the surface (denominator close to zero). The Hamaker constant is itself dependent on the nature of interacting particles and surface and has a value between 10^-20 and 10^-19 Joules. A ratio of van der Waals force to Stokes drag force on a 1 μm particle with a velocity of 1 meter/second in water shows that the former force can be hundred times greater than the latter force.¹³ The van der Waals dimensionless number is presented as the ratio of the Hamaker constant to the drag force in the collector efficiency formulation and decreases with increasing particle size. The gravitation dimensionless number is proportional to the difference between the density of particle and water and the particle diameter, thus dense and large particles have more opportunity to contribute to collector efficiency. The values of these two dimensionless numbers are not changing over the collector diameters considered.

The use of Rajagopalan and Tien (RT) model allowed the estimation of dimensionless numbers for the four terms stipulated previously and the derivation of a collector’s efficiency for the gravel inlet (Table 1). It also gives an approximation of trapping efficiency over all particle and aggregate size range. Some gravel parameters measured in Oveson experiments (2001) were used to compare collector’s efficiency of different collector sizes (for gravel of d₅₀ = 1.36 cm and 0.50 cm). A third value of collector diameter, 0.85 cm, tested in the R& T model comes from the field set-up in Le Sueur County. Again, collector’s efficiency is a combination of four dimensionless numbers including diffusion, London and van der Waals forces, gravitation, and interception. The contributions of London-Van der Waals (Figure 2) and gravitation forces (Figure 3) to the collector efficiency as dimensionless numbers remained the same over all collector sizes. However, Peclet (Figure 2) and interception (Figure 3) dimensionless numbers were dependent on collector size. The curves of collector efficiency showed a minimum at below 0.1 μm particle size for the three sizes listed above (a curve for 0.1 cm collector diameter size was added for comparison); however, collector efficiency increased almost linearly above 0.1 μm particle size (Figure 4). The largest collector size (d₅₀ = 1.36 cm) has a higher collector efficiency compared to the three smaller collector sizes (0.85 cm, 0.50 cm, and 0.10 cm) for particle size range above 0.1 μm. Below 0.1 μm particle size range, the smallest collector (0.10 cm) had more collision efficiency. One must remember that the overall removal of particles is also influenced by the collector size with the other equation parameters kept equal. Ultimately, the trapping efficiency results in Figure 4 were heavily influenced by collector size despite that collector efficiency was highest with the largest collector diameter (1.36 cm). The smallest collector size (0.10 cm) ended up removing more particles in both the sub-micron size and above 1-micron ranges. Comparison of the gravel size range showed that trapping efficiency is expected to be operating with incoming particles and aggregate size greater than 1 μm. For gravel diameter of 0.50 cm and 0.85 cm, it can reach 82% and 53% of removal for incoming particle and aggregate in the fine silt range and above (> 2 μm) (Figure 4).

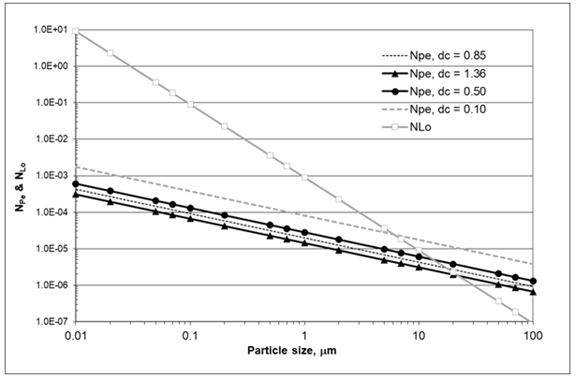
Figure 2 Dimensionless Numbers for Peclet (N_Pe) and London Van-der-Waals forces (N_Lo) for four collector sizes (dc) and over the range of particle sizes.

Figure 3 Dimensionless Numbers for Interception (N_R) and Gravitation (N_G) forces for four collector sizes (dc) and over the range of particle sizes.

Figure 4 Expected trapping efficiency for gravel diameter of 1.36 cm, 0.85 cm, 0.50 cm, and 0.10 cm using the Rajagopalan and Tien model.

Gravel materials used in this experiment were of alluvional type commonly found along riverbed. Most of the gravel mass (> 88%) was concentrated between 6.68 mm and 12.5 mm size with a d₅₀ of 8.50 mm based on a cumulative frequency curve of mass. Geological types of gravel found in the mix were limestone, shale, and granite with a proportion by mass of 53%, 26%, and 21%, respectively, associated with diameter greater than 6.68 mm. Shapes of gravel were checked against the Rittenhouse scale were oblong with sphericity equal to 0.84, 0.80, and 0.77 for limestone, shale, and granite. Sphericity for the gravel material means some rounded shape associated with limestone and shale; in contrast, granitic gravels had angles associated with them.¹⁴ Shape of particles can have a major effect on their attachment to collector’s surface¹⁵ for particle size between 200 nm and 1 mm under simulated conditions and high velocity. Limestone gravels were coated with a fine powder (<< 2.0 mm) very reactive to hydrochloric acid with an equivalent calcium carbonate of 62%.

The gravel matrix porosity were checked in the laboratory and yielded an average value of 0.37 based on three samples. The gravel had a density of 1.78 g/cm³.

Laser diffractometry falls into the category of light scattering techniques and is based on the principle that particles diffract light with a given angle proportional to their size.^16-19 The interaction of particle and incident light falling on it produces four related scattering phenomena: refraction, reflection, absorption, and diffraction of the incident light beam.

The laser diffractometer (LD), HORIBA LA-920,²⁰ was used for two types of reading regarding the gravel inlet samples: volume frequency and particle number frequency. The former reading was the first output from the apparatus while the latter reading is obtained from a built-in switch mode. The volume reading covers a large range of particle size from 0.389 μm to 300 μm and refers to the volume of particle (equivalent spherical diameter) read through the laser system. The particle number reading provides the number of particles for each size category. The size intervals for the two readings were similar and increased over the size range from clay to sand:

1. In the clay size particle (particle diameter < 2 μm), interval classes extended from 0.04 μm to 0.25 μm,
2. In the silt size particle (2 μm < particle diameter < 63 μm), interval classes increased from 0.29 μm to 7.5 μm; this category also includes small aggregates.
3. In the sand size particle (particle diameter > 63 μm), interval classes increased from 8.6 μm to 245 μm; this category also includes large aggregates.

For frequency below 0.1 %, the particle number frequency reading was dropped by the diffractometer. In general, this reading limit corresponded to particle size of a few microns (fine silt size). Any particle greater than the limit (within silt to sand size) did not have any particle number reading and a linear interpolation was used to obtain number for the remaining particle size based on the existing particle number. This size category was important in the determination of detachment from the gravel matrix.

In filtration practices, particle counting is the ultimate check for performance.^21-24 This approach can be improved by assigning deposition or detachment to size category such as clay, silt, and sand. In the next sections, both particle count and mass concentration were presented and compared for paired runoff samples (above and below) to assess effectiveness of filtration during a rainfall event. Total solid concentration was the only pollutant considered in this article. Pairing of samples from above and below location depended on synchronization of sampling starting time above and below the gravel inlet. For some of the events, two samples from above matched one sample from below or vice-versa; in some cases, above and below samples were simply synchronous. For every multiple sample matching and pairing, particle number of each sample were both reported and graphed. On the other hand, total solid masses were averaged for paired comparison and deposition assessment. The analysis was conducted for both intra-event and inter-event. Two methods were used to compare retention and detachment: particle counting method (PCM) and mass concentration method (MCM). PCM presents an extra advantage by assigning retention or detachment to particle size category such as clay, silt, or sand.

Particle counting method required several steps to complete the particle counting from the laser diffractometer reading: (1) direct reading of volume frequency from LD. Large particles and aggregates volume frequency values were large compared to those of small particles and aggregates, (2) conversion of volume frequency to particle count frequency. Small particle count frequency values were greater compared to those of large particles and aggregates. (3) particle count frequency dropped any frequency lower than 0.1%. (4) particle count frequency was converted to particle count assuming an equivalent sphere shape for given diameters and different densities for particle and aggregates sizes. Assumed density values were 2.65 for particle less than 2 μm in diameter, 1.80 for particle and small aggregates greater than 2 μm but smaller than 63 μm, and 1.60 for particle and large aggregates greater than 63 μm (Foster et al., 1985). (5) particle mass for each size category was determined based on spherical volume and density. The mass concentration of each sample helped complete the particle count of each size category (Table 2).

Filtration terms such as retention, trapping efficiency, and removal of particles have the same meaning and will be used interchangeably in the following exposition. Retention is also equivalent to the term “attachment” and is expressed as the complement to 1 to the mass concentration ratio of effluent to influent (1 – C/C_o, Co = influent concentration, and C effluent concentration) with unit in percent. This ratio could become negative when effluent concentration was higher than that of influent, which translates into detachment or re-entrainment of previously deposited particle. Detachment expresses either particles leaving the porous matrix by re-entrainment/ collision or particles that are not retained in the matrix and eventually decreases the filtration quality of the effluent. As for the particle size category, the terms silt and sand include small and large aggregates, respectively.

One of the major assumptions in this experiment was that concentration measured at the sampling point (10 meters away from the gravel mound) is applicable to the top of gravel trench. Also, the rainfall events described in the following paragraphs have been described earlier in a previous article.²⁵

Event of June 21, 2002

Samples obtained from this event covered three distinct periods: (1) first period, June 21 early morning to mid-afternoon; (2) second period, June 23 middle of the day to June 24 early morning; (3) third period, June 24 middle of the day and June 25 early morning (Figure 5). A large gap occurred between period 1 and 2 due to equipment failure (pressure transducer problem) and a simple linear interpolation was used to connect the concentration graphs.

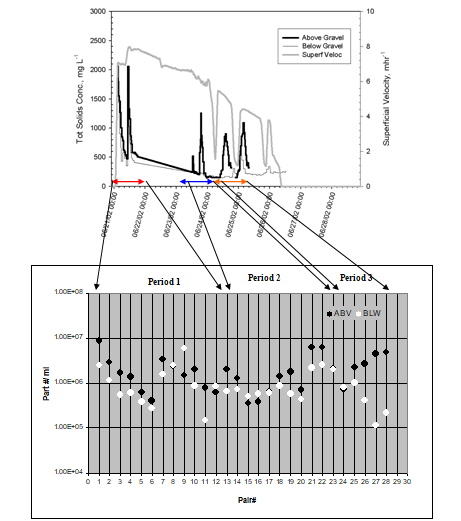
Figure 5 Total solids concentration and superficial velocity (top graph) with particle count graph (bottom graph) for June 21, 2002. The three periods are identified on both graphs. Filtration (attachment) occurs when black dots are above white dots; detachment is the opposite.

From the first period, there were two distinct concentration peaks (2064 mg L^-1 and 2058 mg L^-1) that corresponded to peak rainfall intensities. This series contained thirteen pairs of samples. The average removal (deposition) based on MCM is 28% with a range between 14% and 53%. PCM showed one detachment occurrence at the first sample pair in the sand size and several detachments following the second peak concentration (pair # 7), mostly in the clay and silt size particle (Table 3). More detachment was observed in samples following increase of superficial velocity from about 7 m hr^-1 to 8 m hr^-1. In the first period, the magnitude of retention seems to follow the concentration fluctuations with some delay regarding the peak values.

From the second period, there were two peaks of influent concentration not related to rainfall (first at 550 mg L^-1 and second at 1260 mg L^-1); these concentration increases coincided to field sampling twice on 6/23/02 at 11:00 and 21:00. The concentration increase is an artificial one (re-suspension of deposited sediment) and not based on natural input (rainfall) (Figure 5). On either side of the second concentration peak, retention became small (MCM) compared to the first period and showed some detachment (PCM) in the clay and silt size range. On average, the retention for the second period is 24% with a range between 78% for retention and –19% for detachment.

For the third period of sampling, two peaks of concentration followed small rainfall bursts with some delay. Retention by MCM are larger compared to that of the first period with a range from 24% to 80%. On average, filtration reached 37% for this period. Some detachment in the clay and silt range occurred before the peak concentration.

Overall, the June 21 event had a trapping efficiency of 32% by MCM. PCM has shown some detachment mostly in the clay and silt (and small aggregate) range; based on total particle and aggregate count, 6 out of 28 pairs had detachment mostly in the clay and silt (and small aggregate) sizes. Overall detachment based on PCM gave values of 5.14 x 10⁶ compared to 3.82 x 10⁷for attachment; these values suggest that more attachment occurred during this event (Table 3). From above the gravel, average particle size based on PCM was 0.84 μm, 0.90 μm, and 0.55 μm for the first, second, third period, respectively; for the same periods, average maximum particle and aggregate size reached 7.5 μm, 8.1 μm, and 4.1 μm, respectively (Figure 3). The re-suspension of sediment was probably the cause of maximum particle and aggregate size increase during the second period. From below the gravel, average and maximum particle (and aggregate) sizes were 0.92 μm and 8.0 μm, 0.67 μm and 5.2 μm, and 0.78 μm and 6.5μm for the first, second, and third period, respectively.

Event of August 3, 2002

Samples from this event were divided into two periods related to peak concentrations: (1) first period following the rainfall burst and (2) second period following re-suspension of the bottom sediment by sampling (Figure 6).

Figure 6 Total solids concentration and superficial velocity (top graph) with particle count graph (bottom graph) for August 3, 2002. The two periods are identified on both graphs. Filtration (attachment) occurs when black dots are above white dots; detachment is the opposite.

In contrast to June 21 event, trapping efficiency average based on MCM of this event was much lower with an average of 4.4%, of which 1.2% for the first period and 6.9% for the second period. Range of percentage trapping for the first period was between 86% and –26%; for the second period, trapping efficiency encompassed a range between 56% and –40%. Furthermore, only four pairs of sample out of eleven showed particle retention during the first period with three of them at the beginning and one pair toward the end of the period. Both periods showed some detachments based on MCM: in the first period, detachment occurred in 64% of samples while in the second period it decreased to 43% of samples. From above the gravel, 80% of samples had concentration less than 200 mg/L while below the gravel 100% of samples were less than 200 mg/L. PCM showed significant detachment in the clay size range with the same order of magnitude. Most of particle and aggregate losses (clay, silt, and sand) occurred within the first period. Based on PCM, detachment represented about one-fourth of attachment for this event. Detachment is greater compared to the June 21 event (Table 4).

Average particle and aggregate sizes were 1.8 μm and 3.4 μm for the first and the second periods, respectively, above the gravel; sizes were 1.7 μm and 3.6 μm below the gravel for the same periods (Figure 6). Average maximum sizes were 18 μm and 16 μm from above and below the gravel during the first period and 35 μm and 37 μm from above and below the gravel during the second period. These sizes are much larger than those from the first event on June 21.

Event of august 21, 2002

The samples collected for this event did not cover the whole event. There were 23 pairs of samples in total for which the average trapping was only 0.8%. Based on MCM, 43% of sample pairs had filtration against 57% that had detachment. Overall, detachment dominated this event with particles mostly in the clay and silt (and small aggregate) sizes that were re-entrained or not retained in the gravel matrix. Value of detachment based on PCM was 1.08 x 10⁷ compared to that of attachment at 4.62 x 10⁶ (Table 5). Samples with mass concentration below 200 mg/L were 87% and 96% from above gravel and below the gravel, respectively (Figure 7).

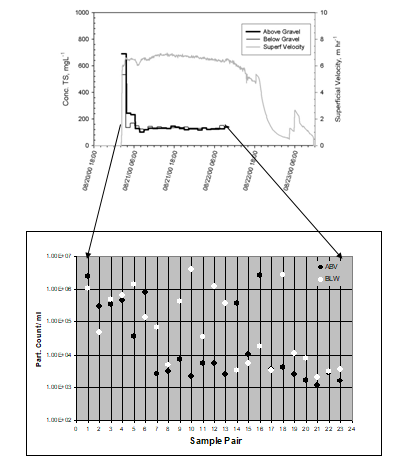
Figure 7 Total solids concentration and superficial velocity (top graph) with particle count graph (bottom graph) for August 21, 2002.

For average and maximum particle and aggregate size based on particle counting method, there were three distinct groups: (1) sample pairs from #1 to #6 had an average size of 0.86 μm and 0.91 μm above and below gravel, respectively; maximum sizes were 8.3 μm and 8.1 μm from the corresponding sampling locations; (2) sample pairs from #7 to #17 had an average 3.0 μm and 2.0 μm above and below gravel, respectively; maximum sizes were 29 μm and 20 μm from the corresponding sampling locations; (3) sample pairs 18-24 had an average 4.9 μm and 3.9 μm above and below gravel, respectively; maximum sizes were 49 μm and 40 μm from the corresponding sampling locations (Figure 7). There was a sensible increase in particle and aggregate sizes throughout the event. The average and maximum particle and aggregate sizes were larger than that of August 3 toward the end of the event.

Event of Oct 4, 2002

This event record was divided into three periods: (1) the first period covered the initial rainfall burst during which no surface ponding occurred therefore no “above gravel” sample was collected; (2) the second period covered the second rainfall burst and included 8 sample pairs; (3) the third period followed a peak concentration due to re-suspension of sediment during sampling with 9 pairs (Figure 8). Computation of trapping efficiency based on MCM covered the last two periods while that based on PCM had only the third period. The third period had its peak concentration due to re-suspension by sample collection in the field. Based on MCM, there were 18 pairs in total for this event on which attachment and detachment occurred each in 50% of the samples; the second and third period had an average trapping efficiency of 8.4% and 7.2%, respectively, with an overall average of 7.7%. Based on PCM, only the third period had record available for LD analysis and showed 78% of sample pairs with attachment and 22% with detachment. Most of the detachment occurred in the clay size particle (Table 6). Average particle and average aggregate size were 2.5 μm and 3.5 μm from above and below gravel while average maximum particle and maximum aggregate size was 25 μm and 34 μm from above and below gravel, respectively.

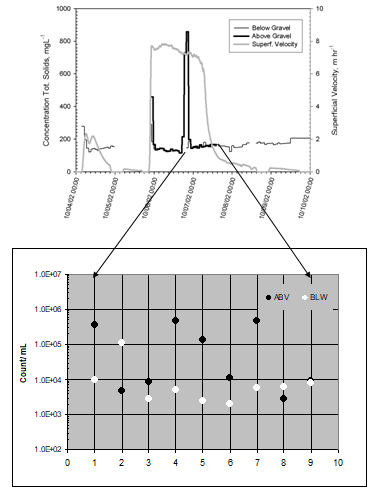
Figure 8 Total solids concentration and superficial velocity (top graph) with particle count graph (bottom graph) for October 4, 2002.

Two major methods were used to quantify retention or trapping efficiency for each event. MCM was based on mass concentration of paired samples from above and below and gave an average retention or detachment value for the entire event. PCM provided details of retention/detachment within particle (and aggregate) sizes. In general, there was agreement on the values of detachment between MCM and PCM. Small values of retention and negative values for detachment based on MCM corresponded to detachment values based on PCM. When detachment occurred mostly in the clay and silt (and small aggregate) sizes the impact on mass was limited and one can still see some attachment using MCM.

Values of particle and aggregate retention for the four rainfall events in 2002 were 32%, 4.4%, 0.79%, and 7.7% based on MCM on June 21, Aug 3, Aug 21, and October 4, respectively. Annual trapping efficiency was 11% by averaging these values. Statistical tests (paired t-test) showed that only the first value for retention (June 21) was significant (p < 0.001 at 10%). Probability associated with retention values tested with a paired t-test at 10% level for August 3, August 21, and October 4 were 0.192, 0.258, and 0.196, respectively. Majority of this retention occurred with the first event while the gravel matrix was still new and environmental conditions such as crop residue and canopy cover provided little protection. Mass concentrations were much larger in the initial event compared to the rest of the season. For the June 21 event, sample pairs with mass concentration less than 200 mg/L was 18% and 36% from above and below the gravel, respectively. Later in the season, canopy cover increased and decreased rainfall energy to transport soil particles into the pond and concentration. Samples from above and below gravel with mass concentration less than 200 mg/L reached 90% of total sample count within each event after June 2002. The value of 200 mg/L for mass concentration was chosen due to two reasons: (1) most of detachment occurred below that concentration; (2) this concentration constituted a borderline for the accuracy of the laser diffractometer (LD) readings. Above this concentration, LD readings gave a light transmission around 96%, which allowed an adequate reading in terms of accuracy for particle and aggregate sizes. Below 200 mg/L, reading gave light transmission close to 99% that gave some problems of accuracy.

Superficial velocity might have had some impacts on detachment. Sample concentrations from below the gravel inlet showed some increase in several instances when there was a large variation in superficial velocity: 1) three times with the June 21 event (6/25/02, 02:00; 6/25/02, 02:00; 6/25/02, 22:00) and 2) twice with the August 3 event (8/5/02, 00:00; 8/5/02, 10:00). These concentration changes may reflect disturbance and entrainment of previous deposits as flow velocity decreases or increases. For the other events, August 21 and October 4, effects of superficial velocity on concentrations were not as obvious as with the June event; however, a combination of low mass concentration and superficial velocity variability may have caused many detachments observed within sample pairs for those listed events. Such effects of velocity on effluent concentration were observed in laboratory experiments.^26,27 Filtration is a delicate balance between forces that initiate attachment and those that generate detachment. Usually shear forces by flowing fluid cause detachment or re-entrainment of deposited particles. In filtration practice, it is recommended to keep the superficial velocity as constant as possible to meet effluent quality. At the start of filtration runs in the early 1960s, a device called “slow-start module” will regulate the rate of flow increase from zero to full rate over a selected period between 15 and 60 minutes.²⁶ Rates of flow increase were kept very low to prevent breakthrough and to ensure effluent quality.

Maximum mass concentrations in samples were synchronous with large and small rainfall bursts and re-suspension due to sample collection. It was observed as well that maximum retention based on paired samples occurred at or close to maximum concentrations for each event. For the June 21 event, maximum trapping efficiency values occurred following rainfall bursts three times and following re- suspension one time. In between or following peak concentrations, samples concentrations declined fast usually close to or below 200 mg/L. Rainfall energy associated with bursts allowed some significant transport of sediment from higher grounds toward the gravel in the depression area. For the remaining events, maximum trapping efficiency occurred at initial rainfall bursts that coincided with maximum concentrations for “above gravel” samples.

Average particle and aggregate sizes based on article counting method were relatively small during the initial event in June ranging mostly in the sub-clay size (less than 1.0 μm): the first and second period had an average particle size of 0.84 μm and 0.89 μm, respectively. The third period has seen an average particle size of 0.55 μm. Average maximum particle and aggregate sizes for the three periods were 7.5 μm, 8.1 μm, and 4.1 μm, respectively. Such decrease in size over time may be due to settling of larger particle and aggregate sizes keeping in mind that water ponding for this event lasted for five days. By comparing above and below gravel particle and aggregate sizes for the June 21 event, average particle sizes are very close with 0.76 μm from above and 0.79 μm from below with a slight increase in size. Most of the detachment for the June event occurred within the clay particle size in terms of particle and aggregate numbers. The three remaining events have seen a net increase in particle and aggregate sizes: the August 3 event had an average size of 2.6 μm with its probable cause due to re-suspension of settled particles and aggregates by field sampling for “above gravel” samples. The October 4 event had the same increase in particle and aggregate sizes due to re-suspension of settled sediment. The August 21 event had a peculiar behavior with increasing particle and aggregate sizes within the event: three groups of sediment sizes were identified with averages of 0.86μm, 3.0μm, and 4.9μm, respectively.

Filtration methods allowed the assessment of gravel inlet trapping efficiency, which is based on mass concentration of “above” and “below” samples. Based on two methods of computing averages, trapping efficiency is given as a range between 11% and 22% rather than a unique number. Rajagopalan and Tien model was used to predict trapping efficiency for a range of particle sizes from 0.01 μm to 100 μm for a range of collector size including the gravel used in the field with d₅₀=0.85cm. In general, the model showed a relatively small trapping efficiency (<50%) due to collector (gravel) size for large aggregates and sand size category. Retention of clay and fine silt particle was even predicted to be much smaller (<20%) than those of large size particle and aggregate. The smaller the collector, the greater the filtration ability of the medium. However, mass concentration method showed that retention for some paired samples reached as high as 86% and most of it occurred with clay size particle. Such large trapping efficiency was reported by Gieseke³ in a gravel inlet experiment. The field experiment has shown that maximum filtration occurred with peak concentrations following rainfall bursts even for event occurring one year from the start of the experiment. Usually, concentration above 500 mg/L gave large values of trapping efficiency. Concentrations below 200 mg/L gave little retention or literally generated detachment.

Laser diffractometry (LD) methods has helped to quantify detachment based on particle count of paired samples from “above” and “below” the gravel inlet. Samples particle count was conducted using LD outputs and based on assumed aggregate density values as provided by Foster et al.²⁸ On the filtration cycle model and based on filtration practices, detachment occurs as the same time as attachment. It can start as early as the effective filtration period and increases toward the end of the filter cycle until the deterioration of the effluent quality. In the present experiment, detachment occurred with the first event and increased for the two following events (August 3 and 21). The latter date was all detachment with most in the clay size particle. Average particle and aggregate size within an event tended to decrease over time due to settling of larger particles; however, it was observed that artificial re-suspension of the deposited sediment in the basin increased significantly for average particle sizes for any given event.²⁹

We are indebted to the funding support from several organizations for this research project: Minnesota Department of Agriculture, Corn Grower Association, Minnesota Pollution Control Agency, and the Metropolitan Council. The grant numbers are as follows: 1/ USEPA Section 319 of the Clean Water Act 319, Grant # C9995006-00-0 CFMS #A18445 and, 2/ Minnesota Corn Research and Promotion Council, Grant # 01-08SP. We are also incredibly grateful to the field assistance of Mr. Edward Dorsey, Mr. Thor Sellie, and Mr. Andrew Scobbie.

None.

1. Herzig JP, LeClerc DM, LeGoff. Flow of Suspension through Porous Media. Industrial and Engineering Chemistry. 1970;62(5):8.
2. Remond S. DEM simulation of small particles clogging in the packing of large beads. PhysicaA 389. 2010:4485–4496.
3. Gieseke T. A comparison of sediment and phosphorus losses from rock inlets and open tile inlets in the Lower Minnesota River Basin. Master’s Degree Thesis. Mankato State University, Minnesota. 2000.
4. Oveson KL. Evaluation and Design of Rock Inlets as an Alternative Form of Surface Drainage. MS Thesis, University of Minnesota. 2001.
5. Hozalski R. Personal Communication. Civil Engineering Dept. University of Minnesota. 2002.
6. Amirtharajah. Some theoretical and conceptual views of filtration. Journal AWWA. 1988;80(12):36.
7. Jegatheesan V, Vigneswaran. Deep Bed Filtration: Mathematical Models and Observations. Critical Reviews in Environmental Science and Technology. 2005;35:515–569.
8. Tiantian Li, Yan Jin, Yuanfang Huang, et al. Observed Dependence of Colloid Detachment on the Concentration of Initially Attached Colloids and Collector Surface Heterogeneity in Porous Media. Environmental Science & Technology. 2017;51(5):2811–2820.
9. Keir G, Jegatheesan, Vigneswaran. Deep Bed Filtration: Modelling Theory and Practice. Water and Wastewater Treatment Technologies. UNESCO–EOLSS. 2009.
10. Logan BE, Jewett, Arnold, et al. Clarification of clean filtration models. Journ of Envir Eng. 1995;121(12):869–873.
11. Rajagopalan R, Tien C. Trajectory analysis of deep filtration with the sphere–in–cell porous media model. AIChE Journal. 1976;22(3):523–533.
12. Nelson KE, Ginn. Colloid Filtration Theory and the Happel Sphere–in–Cell Model Revisited with Direct Numerical Simulation of Colloids. Langmuir. 2005;21:2173–2184.
13. Ahmadi G. London van der Waals Force, Course Material: Mechanical Engineering 437/537. Clarkson University, South Carolina. 2004.
14. Yuichi Hayakawaa ,Takashi Oguchib. Evaluation of gravel sphericity and roundness based on surface–area measurement with a laser scanner. Computers & Geosciences. 2005;31(6):735–741.
15. Ke Li, Huilian Ma. Rotation and Retention Dynamics of Rod–Shaped Colloids with Surface Charge Heterogeneity in Sphere–in–Cell Porous Media Model. Langmuir. 2019;35(16):5471–5483.
16. Jillavenkatesa A, Dapkunas, Lin–Sien H Lum. Particle size characterization. Material Science and Engineering Laboratory. Special Publication 960–1. NIST Recommended Practice Guide. Department of Commerce, USA. 2001.
17. Konert M, Vandenberghe. Comparison of laser grain size analysis with pipette and sieve analysis: a solution for the underestimation of the clay fraction. Sedimentology. 1997;4:523–535.
18. Loizeau JL, Arbouille S, Santiago, et al. Evaluation of a wide range laser diffraction grain size analyzer for use with sediments. Sedimentology. 1994;41:353–361.
19. Beusenlick LG, Govers, Poesen, et al. Grain–size analysis by laser diffractometry: comparison with the sieve–pipette method. Catena. 1999;32:193–208.
20. Ravindra M, Srivastava. Effect of sequence of measurement on particle count and size measurements using a light blockage (HIAC) particle counter. Water Research. 1993;27(5):939–942.
21. HORIBA Instruments Inc. LA–920 instruction manual. 1st edition. Irvine Industrial Complex, Irvine, California. 1997.
22. Ndiongue S, Desjardins, Prévost. Relationships between total particle count, aerobic spore–forming bacteria and turbidity in direct filtration. Journal of Water Supply: Research and Technology–Aqua. 2000;49(2):75–88.
23. Hatukai S, Ben–Tzur, Rebhun. Particle counts and size distribution in system design for removal of turbidity by granular deep bed filtration. Water Sci Technol. 1997;36(4):225–230.
24. Broadwell M. A Practical guide to particle counting for drinking water treatment. Lewis Publishers. Boca Raton, FL. 2001.
25. Ranaivoson AZ, Moncrief. Gravel inlet hydrology and trends of contaminants and loading. Int J Hydro. 2019;3(5):376–388.
26. Cleasby JL, Williamson MM, Baumann. Effect of rate changes on filtered water quality. Journal of American Water Works Association. 1963;55:869–880.
27. Kau SM, Lawler. Dynamics of Deep–Bed Filtration: Velocity, Depth, and Media. Journal of Environmental Engineering. 1995;121(12).
28. Foster GR, Young RA, Neibling WH. Sediment composition for one–point source pollution analyses. Trans. Am Soc Ag Engrs. 1985;28:133–139.
29. Yao KM, Habibian, O’Melia. Water and wastewater filtration: concepts and application. Environmental Science and Technology. 1971;5(11):1105–1112.