eISSN: 2576-4500


Technical Paper Volume 1 Issue 3
School of Mechanical Engineering, Jimma University, Ethiopia
Correspondence: Rajesh Kumar, School of Mechanical Engineering, JIT, Jimma University, P.O. Box-378, Jimma, Ethiopia, Tel 251909462675
Received: May 11, 2017 | Published: October 9, 2017
Citation: Kumar R. Effects of elastic foundations on flexural response of shear deformable laminated plates subjected to transverse uniform lateral pressure uncertain system environment and hygrothermomechanical loading. Aeron Aero Open Access J. 2017;1(3):84-101. DOI: 10.15406/aaoaj.2017.01.00012
In this paper, the effect of elastic foundations on flexural response of shear deformable laminated plates subjected to transverse uniform lateral pressure under uncertain system environment and hygrothermomechanical loading using MATLAB [R2010a] code for micromechanical model approach is investigated. A C0 finite element method in conjunction with the first order perturbation technique extended by authors for plate subjected to lateral loading is employed to find out the second order response statistics (expected mean and coefficient of variations) of the transverse deflection of the plate. Plate material properties and elastic foundation parameters are taken as basic random variables. The plate is analysed for plate thickness ratios, aspect ratios, boundary conditions, lamina lay-up, fiber volume fractions, load deflections and environmental conditions. The performance of the stochastic laminated composite model is demonstrated through comparison of mean transverse central deflection by comparison with the results available in literatures and standard deviation results with independent Monte Carlo simulation before data generation.
Keywords: stochastic bending response, finite element method, uncertain system properties, elastic foundations
Aij, Bij, etc |
Laminate stiffnesses |
a, b |
Plate length and breadth |
h |
Thickness of the plate |
Ef, Em |
Elastic moduli of fiber and matrix, respectively. |
Gf, Gm |
Shear moduli of fiber and matrix, respectively. |
vf, vm |
Poisson’s ratio of fiber and matrix, respectively. |
Vf, Vm |
Volume fraction of fiber and matrix, respectively. |
αf, αm |
Coefficient of thermal expansion of fiber and matrix, respectively. |
bi |
Basic random material properties |
E11, E22 |
Longitudinal and Transverse elastic moduli |
G12, G13, G23 |
Shear moduli |
Kl, |
Linear bending stiffness matrix |
Kg |
Thermal geometric stiffness matrix |
D |
Elastic stiffness matrices |
Mαβ,mαβ |
Mass and inertia matrices |
ne, n |
Number of elements, number of layers in the laminated plate |
Nx, Ny, Nxy |
In-plane thermal buckling loads |
nn |
Number of nodes per element |
Ni |
Shape function of ith node |
ˉCpijkl |
Reduced elastic material constants |
f, {f}(e) |
Vector of unknown displacements, displacement vector of eth element |
u, v, w |
Displacements of a point on the mid plane of plate |
ˉu1, ˉu2, ˉu3, |
Displacement of a point (x, y, z) |
ˉσij,ˉεij |
Stress vector, Strain vector |
ψy, ψx |
Rotations of normal to mid plane about the x and y axis respectively |
θx, θy, θk |
Two slopes and angle of fiber orientation wrt x-axis for kth layer |
x, y, z |
Cartesian coordinates |
ρ, λ, Var(.) |
Mass density, eigenvalue, variance |
ω,ϖ |
Fundamental frequency and its dimensionless form |
RVs |
|
DT, DC, |
Difference in temperatures and moistures |
α1, α2, β1, β2 |
Thermal expansion and hygroscopic coefficients along x and y direction, respectively. |
Laminated composite plates are often subjected to combination of lateral pressure and hygrothermomechanical loading. The plates are more advantageous over plates made of conventional materials and they are more hygrothermally and mechanically stable than plates made of conventional metals. The capability to predict the structural response and enable a better understanding and characterization of the actual behavior of laminated composite plates resting on elastic foundations in terms of structural response when subjected to combined load is of prime interest for structural analysis. Composite structures have inherent dispersion in system properties due to lack of strict quality control and the characteristics of the large parameters involved with the manufacturing and fabrication process. The variation in the system properties of the composite materials necessitates the inclusion of randomness of system properties in the analysis; otherwise predicted response may differ significantly rendering the structures unsafe. For reliable and safe design, especially for sensitive engineering applications in thermal environments. Accurate prediction of system behavior of composite structures in the presence of uncertainties in the system properties favors a probabilistic analysis rather than analytical approach by modeling their properties as basic random variables.
Stochastic micromechanical modeling investigation yields more accurate system behavior and proved to be superior technique for design compared to stochastic macromechanical modeling investigations. Material properties, geometric properties, foundation stiffness parameters, fiber orientations, lamina lay-up sequence design and curing are of prime importance and these parameters must be considered in accurate prediction of uncertain system behavior of composites. Thus there is importance of uncertainties accountability in the responses. Laminated composite plates are widely used in aerospace, submarines, automotive industries, nuclear structures and in various structural components such as beams, thin and thick plates, shells, panels etc.
A considerable literature is available on the static response of geometrically linear and nonlinear composite laminated plates under various thermal, hygrothermal and mechanical loads or combination of both. Notably among them are Shen,1 Huang & Tauchert,2 Sen,3 Lin et al.4 Shen.5 Whitney et al. 6 studied the effect of environment on the elastic response of layered composite elates. Adam et al.7 Lee et al.8 Sai Ram and Sinha.9 Patel et al.10 Shen11,12 Non-linear bending of shear deformable laminated plates under lateral pressure and thermal loading and resting on elastic foundations and hygrothermal effects on the nonlinear bending of shear deformable laminated plates.
Salim et al.13 examined the effect of randomness in material properties (like elastic modulus Poisson’s ratios etc.,) on the response statistics of a composite plate subjected to static loading using classical plate theory (CLT) in conjunction with first order perturbation techniques (FOPT).14-17
A little literature is available on stochastic analysis for macromechanocal and micromechanical model investigation.18-30 Keeping in mind the above aspect, to the best of the authors’ knowledge, there is no literature covering effects of elastic foundations on flexural response of shear deformable laminated plates subjected to transverse uniform lateral pressure under uncertain system environment and hygrothermomechanical loading,
However, no work is available dealing with effects of elastic foundations on flexural response of shear deformable laminated plates subjected to transverse uniform lateral pressure uncertain system environment and hygrothermomechanical loading to the best of author’s knowledge.
In the present investigation, effects of elastic foundations on flexural response of shear deformable laminated plates subjected to transverse uniform lateral pressure uncertain system environment and hygrothermomechanical loading in the presence of small random variation in the system properties, the transverse shear strain using higher order shear deformation theory (HSDT) with von-Karman sense is studied by using stochastic analysis for micromechanical model. The C0 finite element method is employed to determine the second order statistics (mean and standard deviation) of flexural response parameter of laminated composite plates with uniform constant temperature (U.T). The numerical illustrations are concerned with flexural response behavior under different sets of thermo-material properties, stacking sequence, fiber volume fractions, plate thickness ratios, aspect ratios, different boundary conditions, and foundation stiffness, coefficient of hygroscopic expansions and coefficients of thermal expansions. It is observed that small amount of random variations in above mentioned parameters of the composite plate significantly affect the flexural response especially at micromechanical investigation.
Consider geometry of laminated composite rectangular plate of length a, width b, and thickness h, which consists of N-plies located in three dimensional Cartesian coordinate system (X, Y, Z) where X- and -Y plane passes through the middle of the plate thickness with its origin placed at the corner of the plate as shown in Figure 1. Let (ˉu, ˉv, ˉw) be the displacements parallel to the (X, Y, Z) axes, respectively. The thickness coordinate Z of the top and bottom surfaces of any kth layer are denoted by Z(k-1) and Z(k) respectively. The fiber of the kth layer is oriented with fiber angle θk to the X- axis. The plate is assumed to be subjected to uniformly distributed transverse static load is defined as q(x,y)=qo
The plate is assumed to attach to the foundation so that no separation takes place in the process of deformation.1,2 The interaction between the plate and the supporting foundation follows the two- parameter model (Pasternak-type) as:
P=K1w−K2∇2w
Where P is the foundation reaction per unit area, and ∇2=∂2/∂x2+∂2/∂y2 is Laplace differential operator and K1 and K2 are the Winkler and Pasternak foundation stiffness. This model is simply known as Winkler type when K2=0.1
In the present study, the assumed displacement field is based on the Reddy’s22,23 higher order shear deformation theory [1996], which requires C1 continuous element approximation. In order to avoid the usual difficulties associated with these elements the displacement model has been slightly modified to make the suitability for C0 continuous element [1997]. In modified form, the derivatives of out-of-plane displacement are themselves considered as separate degree of freedom (DOFs). Thus five DOFs with C1 continuity are transformed into seven DOFs with C0 due to conformity with the HSDT. In this process, the artificial constraints are imposed which should be enforced variationally through a penalty approach.
In the present study, the assumed displacement field is based on the Reddy’s22,23 higher order shear deformation theory, which requires C1 continuous element approximation. In order to avoid the usual difficulties associated with these elements the displacement model has been slightly modified to make the suitability for C0 continuous element in modified form, the derivatives of out-of-plane displacement are themselves considered as separate degree of freedom (DOFs). Thus five DOFs with C1 continuity are transformed into seven DOFs with C0 due to conformity with the HSDT. In this process, the artificial constraints are imposed which should be enforced variationally through a penalty approach. However, the literature26 demonstrates results using C0 can be obtained. The modified displacement field along the X, Y, and Z directions for an arbitrary composite laminated plate is now written as
ˉu=u+f1(z)ψx+f2(z)ϕx; ˉv=v+f1(z)ψy+f2(z)ϕy; ˉw=w; (1)
where ˉu ,ˉv and ˉw denote the displacements of a point along the (X, Y, Z) coordinates axes: u, v, and w are corresponding displacements of a point on the mid plane, ϕx=w,x andϕy=w,x and ψx , ψy are the rotations of normal to the mid plane about the y-axis and x-axis respectively. The function f1(z) and f2(z) can be written as
f1(z)=C1z−C2z3 ; f2(z)=−C4z3 withC1=1, C2=C4=4h2/3 .
The displacement vector for the modified C0 continuous model is denoted as
{Λ}=[uvwϕyϕxψyψx]T , (2)
where, comma (,) denotes partial differential.
For the structures considered here, the relevant strain vector consisting of strains in terms of mid-plane deformation, rotation of normal and higher order terms associated with the displacement for kth layer are written as
{ε}={εl}−{ˉεHT} (3)
where{εl} and{ˉεHT} are the linear and hygrothermal strain vector, respectively. Nonlinear analysis is given in.25
Using Eq. (3) the linear strain vector can be obtained using linear strain displacement relations which can be written as
{εl}={εLP0}+{zεLbεs}+{0z2ε*s}+{z3ε*0} (4)
The hygrothermal strain vector{ˉεt} is represented as
{ˉεHT}={ˉεxˉεyˉεxyˉεyzˉεzx}=ΔT{α1α2α1200}+ΔC{β1β2β1200} (5)
Stress–strain relation
The constitutive law of thermo-elasticity for the materials under considerations relates the stresses with strains in a plane stress state for the kth lamina oriented as an arbitrary angle with respect to reference axis for the orthotropic layers is given by.27
{σ}k=[ˉQ]k{ε}k
or
{σxσyσxyσyzσxz}k=[ˉQ11ˉQ12ˉQ1600ˉQ12ˉQ22ˉQ2600ˉQ16ˉQ26ˉQ6600000ˉQ44ˉQ45000ˉQ45ˉQ55]k{{εl}−{ˉεHT}} (6)
where, {ˉQij}k , {σ}k and {ε}k are transformed stiffness matrix, stress and strain vectors of the kth lamina, respectively,
Strain energy of the plate
The strain energy ΠSE of the laminated composite plates can be expressed as
ΠSE=12∫{ε}VT[σ]dV . (7)
Strain energy due to hygrothermal stresses
The strain energy (ΠTH) storage by hygrothermal load (uniform and transverse change in temperature and moisture across the thickness) is written as
ΠTH=12∫A[Nx(w,x)2+Ny(w,y)2+2Nxy(w,x)(w,y)] dA =12∫A{w,xw,y}T[NxNxyNxyNy]{w,xw,y}dA (8)
where, Nx, Ny and Nxy are pre-buckling thermal stresses.
Strain energy due to elastic foundations
The strain energy (Π3) due to elastic foundation having foundation layers can be written a
Πf=12∫A{K1(w,x)2+K2[(w,x)2+(w,x)2]} dA =12∫A{ww,xw,y}T[K1000K2000K2]{ww,xw,y}dA (9)
The potential energy due to work done by external mechanical loading of intensity q(x,y) is given by
W=−Wq=∫Aq(x,y) w dA (10)
where, q(x, y) is the intensity of distributed transverse static load which is defined as
q(x,y)=QE22h3b4 (11)
In the present study, a C0 nine-noded isoparametric finite element with 7 degree of freedoms (DOFs) per node as described earlier by {Δ} is employed. For this type of element, the displacement vector and the element geometry are expressed as
{Λ}=NN∑i=1φi{Λ}i; x=NN∑i=1φixi; and y=NN∑i=1φiyi (12)
where φi is the interpolation function for the ith node, {Λ}i is the vector of unknown displacements for the ith node, NN is the number of nodes per element and xi and yi are cartesian coordinate of the ith node.
ΠHT=NE∑e=1[12{Λ(e)}T[K(e)]{Λ}(e)−{Λ(e)}T[F(e)HT]]=12{q}T[K]{q}−{q}T[FHT] (13)
Work done due to external transverse load
Using finite element model (Eq. (10), Equation (9) may be written as
W=NE∑e=1W{e} where W{e}=−∫A(e){Λ}(e)T{PM}(e) dA =−{q}(e)T{PM}(e) (14)
Governing equations
The governing equation for the bending analysis can be derived using Variational principle, which is generalization of the principle of virtual displacement.23 For the bending analysis, the minimization of first variation of total potential energy Π (ΠSE+ ΠHT+ Πf− W) with respect to displacement vector is given by
δ(ΠSE+ ΠHT+ Πf− W) = 0 (15)
[K]{W}={FHT}, (16)
with {FHT}={PM}+{PHT}
where [K], {W}, {PM} and {PHT} are global linear stiffness matrix, response vector, mechanical and hygrothermal force vector, respectively.
The stiffness matrixes [K], displacement vector {W} and force vector [FHT] are random in nature, being dependent on the system properties. Therefore the eigenvalues and eigenvectors also become random. In deterministic environment, the solution of Eq. (16) can be obtained using conventional method.
In the present study, our aim is to find the second order statistics of WRi when the second order statistics of primary RVs bRi are known. Any random variable can be expressed as the sum of its mean and the zero mean random variable which is expressed .The expression only up to the first-order terms and neglecting the second- and higher-order terms are given as random variable RVR =mean (RVs)+ zero-mean random variable (RVR)
The operating random variables in the present case are defined as:
bRi=bdi+bri; KRsij=Kdsij+Krsij; WRi=Wdi+Wri
We can express the above relations in the form:
bRi=bdi+∈bri; KRsij=Kdsij+∈Krsij; WRi=Wdi+∈Wri
where ∈ is a scaling parameter, and is small in magnitude. We consider a class of problems where the zero-mean random variation is very small as compared to the mean part of random variables. i.e., RVd>>∈RVr . Using the Taylor series expansion and neglecting the second and higher-order terms since first order approximation is sufficient to yield results with desired accuracy with low variability which is the cases in most of the sensitive application.
The governing equation (16) can be written in the most general form as:24,28-30
[KR]{WR}={FRHT}={FR} (17)
Zeroth order perturbation equation (∈0):[Kd]{Wd}={Fd} (18)
First order perturbation equation (∈1): [Kd]{Wr}+[Kr]{Wd}={Fr} (19)
W=Wd+{∂Wd∂brl}brl and var(W)=E[∑l∂Wd∂blRblr]2 (20)
Where E [ ] and var (.) are the expectation and variance respectively. The variance can further be written as.28
var (W)=N∑lN∑ldiag[∂Wd∂blR(∂Wd∂blR)T]E(blr,blr) (21)
var(W)=(∂Wd∂blR)[σb][ρ][σb](∂Wd∂blR)T (22)
Eq. (22) expresses the covariance of the deflection in terms of standard deviations (SD) of random variables bi (i=1, 2,…, R) and correlation coefficients.
A nine noded Lagrange isoparamatric element, with 63 DOFs per element for the present HSDT model has been used for discretizing the laminate. Based on convergence study conducted a (8 × 8) mesh has been used throughout the study. In the all problem considered, the individual layers are taken be equal thickness.
The results are presented taking COV of the system property equal to 0.10.31 However, the scattering of system can be taken by allowing the COV to vary from 0 to 20% and the presented results would be sufficient to extrapolate the results keeping in mind the limitation of FOPT. The basic random system variables such as E1, E2, G12, G13, G23, υ12, α1, α2, k1, k2 and Q are sequenced and defined as
b1=E11, b2=E22, b3=G12, b4=G13, b5=G23, b6 = α1, b7 = α2, b8 = β2, b9 = k1, b10 = k2,b11 = Q
The following dimensionless linear transverse mean central deflection has been used in this study.
W0l=Wl/h , k1=K1b4/Ed22h3 ; k2=K2b2/Ed22h3 ,
in which Wi, K1 and K1 dimensional mean transverse central deflection, dimensionless Winkler and Pasternak foundation stiffness parameters respectively.
In the present study,
various combination of boundary edge support conditions namely, simply supported (S1 and S2), clamped and combination of clamped and simply supported have been used and shown in.Figure 2
The plate geometry used is characterized by aspect ratios (a/b) = 1and 2, side to thickness ratios (a/h) = 20, 30, 40, 50, 60, 80 and 100. The following material properties are used for computation Shen:12
Validation study for mean transverse central deflection
Table 1 compares the hygrothermal effects on the linear and non-linear bending behavior of a (±450)2T laminated square plate, dimensionless load deflection (Q) where (Q= q b4/E22h4=100, 150, 200), fiber volume fraction (Vf =0.6), plate thickness ratio (a/h =10), simple support SSSS (S2) boundary conditions and under environmental conditions. It is noticed that present [HSDT] result for mean hygrothermal deflection are in good agreement with the deterministic results of.12 Figure 3 compares the hygrothermal effects on the linear and non-linear bending behavior of a (±450)2T laminated square plate, dimensionless load deflection (Q) where (Q= q b4/E22h4=100, 150, 200), fiber volume fraction (Vf =0.6), plate thickness ratio (a/h =10), simple support SSSS (S2) boundary conditions and under environmental conditions. It is noticed that present [HSDT] result for mean hygrothermal deflection are in good agreement with the deterministic results of.12
(Q) |
Non-Dimensional Hygrothermal Bending Load |
|||||
|---|---|---|---|---|---|---|
Shen 12 |
Present[HSDT] |
Shen 12 |
Present[ HSDT] |
|||
ΔT=00C, ΔC=0% |
ΔT=00C, ΔC=0% |
ΔT=300, ΔC=3% |
ΔT=300, ΔC=3% |
|||
Non-linear |
Linear |
Non-linear |
Non-linear |
Linear |
Non-linear |
|
100 |
0.6887 |
1.328 |
0.6952 |
0.6261 |
0.9377 |
0.6222 |
150 |
0.8857 |
1.5801 |
0.883 |
0.7126 |
1.1067 |
0.7264 |
200 |
0.9909 |
1.7325 |
0.9989 |
0.777 |
1.1837 |
0.768 |
Table 1 Comparison of Hygrothermal Effects on the Linear and Non-linear Bending Behavior of a (±450)2T Laminated Square Plate, Load Deflection (Q) where Q= q b4/E22h4, Fiber Volume Fraction (Vf) =0.6, Plate Thickness Ratio (a/h) =10, Simple Support SSSS (S2) Boundary Conditions under Environmental Conditions


Figure 3 Validation study of dimensionless transverse central deflection of angle-ply square laminated composite plate subjected to linearly varying temperature.
Validation study for random transverse central deflection
Validation study for random hygrothermal central deflection of material properties (E22), plate thickness ratio (a/h) =30, aspect ratio (a/b) =1, rise in temperature(ΔT=2000C) , rise in moisture concentration(ΔC=2%) , simple support SSSS (S2), fiber volume fraction (Vf=0.6) , dimensionless load deflection (Q =100), angle ply antisymmetric (±450)2T laminated composite plate resting on Winkler (k1=100, k2=00) and Pasternak( k1=100, k2=10) elastic foundations is shown in Figure 4. It is seen that present FOPT results are satisfactory with the MCS results.
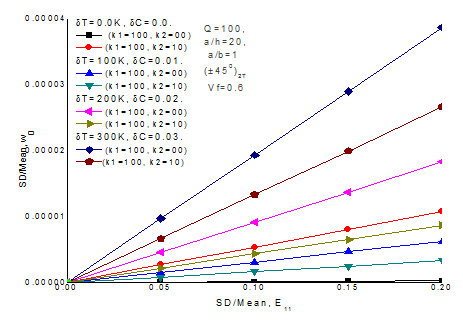
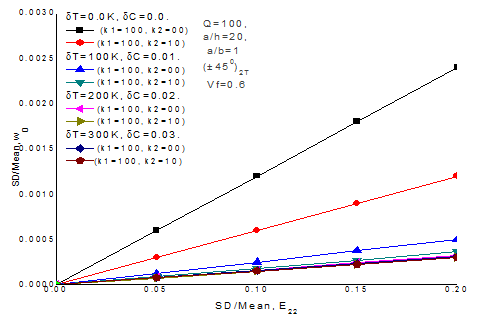
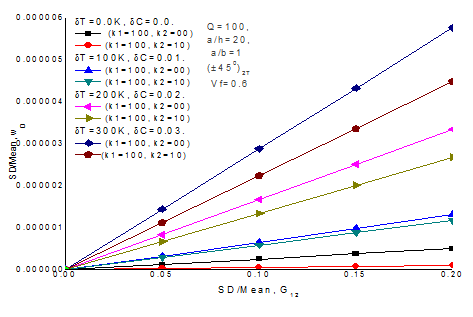
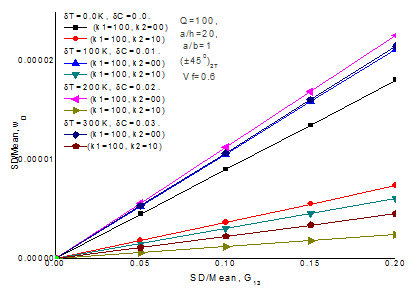
Figure 4 Validation of present SFEM results with independent MCS results of laminate composite square plates resting on Winkler and Pasternak elastic foundations, subjected hygrothermal lateral loading having SSSS (S2) support condition for only one system properties E22.
In (Table 2a) (Table 2b), it is observed that as increases the lateral pressure, the mean transverse central deflection increases and corresponding coefficient of variation decreases. It is noticed that expected mean transverse central deflection (W0l) value of individual random variables of hygrothermal deflection decreases for Winkler elastic foundation and it further decreases for Pasternak elastic foundation whereas COV of hygrothermal deflection increases on rise in temperature and moisture concentration as shown in Figure 5.
(bi) |
(k1=100, k2=00) |
|||
|---|---|---|---|---|
ΔT= 00C, ΔC=0.00 |
ΔT= 1000C, ΔC=0.01 |
ΔT= 2000C, ΔC=0.02 |
ΔT=3000C, ΔC=0.03 | |
COV, Wl, |
COV, Wl, |
COV, Wl, |
COV, Wl, |
|
E11(i=1) |
(0.0351) 2.14e-07 |
(0.0312) 6.15e-06 |
(0.0273) 1.83e-05 |
(0.0234) 3.87e-05 |
E22(i=2) |
0.0024 |
5.00E-04 |
3.27E-04 |
3.11E-04 |
G12(i=3) |
5.06E-07 |
1.31E-06 |
3.35E-06 |
5.76E-06 |
G13(i=4) |
1.81E-05 |
2.12E-05 |
2.26E-05 |
2.15E-05 |
G23(i=5) |
9.08E-06 |
1.06E-05 |
1.13E-05 |
1.07E-05 |
V12(i=6) |
7.19E-05 |
7.19E-06 |
4.77E-06 |
7.14E-06 |
α11(i=7) |
1.53E-11 |
8.77E-11 |
1.58E-10 |
1.86E-10 |
α22(i=8) |
4.05E-12 |
2.10E-10 |
6.75E-10 |
1.40E-09 |
β2(i=9) |
7.42E-10 |
4.11E-08 |
1.31E-07 |
2.73E-07 |
k1 (i=10) |
6.76E-06 |
7.00E-06 |
7.32E-06 |
7.77E-06 |
k2(i=11) |
0 |
0 |
0 |
0 |
Q(i=12) |
2.52E-04 |
2.61E-04 |
2.73E-04 |
2.88E-04 |
Table 2(A) Effects of the Variation of Individual Random System Properties bi, [{(i =1 to 12), = 0.10] on the Dimensionless Expected Mean (W0l) and Coefficient of Variation (Wl) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) Elastic Foundations, Subjected to Uniform Constant Temperature and Moisture (U.T), in-plane Bi-axial Compression, Plate Thickness Ratio (a/h=20), with Simple Support S2 Boundary Conditions. The Dimensionless Mean Hygrothermal Deflections are given in Brackets. Load Deflection Q=100, Fiber Volume Fraction (Vf=0.6)
(bi) |
(k1=100,k2=10) |
|||
|---|---|---|---|---|
ΔT= 00C, ΔC=0.00 |
ΔT= 1000C, ΔC=0.01 |
ΔT= 2000C, ΔC=0.02 |
ΔT=3000C, ΔC=0.03 |
|
COV, Wl, |
COV, Wl, |
COV, Wl, |
SD/Mean, Wl, |
|
E11(i=1) |
(0.0330) 1.07e-05 |
(0.0291)3.26e-06 |
(0.0253) 8.63e-06 |
COV, Wl, |
E22(i=2) |
0.0012 |
3.63E-04 |
2.98E-04 |
3.01E-04 |
G12(i=3) |
1.03E-07 |
1.18E-06 |
2.68E-06 |
4.48E-06 |
G13(i=4) |
7.42E-06 |
6.09E-06 |
2.43E-06 |
4.57E-06 |
G23(i=5) |
3.71E-06 |
3.04E-06 |
1.21E-06 |
2.28E-06 |
V12(i=6) |
0.0017 |
1.08E-04 |
1.20E-05 |
2.51E-07 |
α11(i=7) |
2.03E-10 |
3.10E-10 |
4.21E-10 |
5.30E-10 |
α22(i=8) |
2.17E-09 |
1.81E-09 |
1.39E-09 |
8.93E-10 |
β2(i=9) |
4.16E-07 |
3.47E-07 |
5.82E-06 |
1.67E-07 |
k1 (i=10) |
5.63E-06 |
5.70E-06 |
5.82E-06 |
5.98E-06 |
k2(i=11) |
5.29E-06 |
5.59E-06 |
5.79E-06 |
5.85E-06 |
Q(i=12) |
2.52E-04 |
2.64E-04 |
2.78E-04 |
2.93E-04 |
Table 2(B) Effects of the Variation of Individual Random System Properties bi, [{(i =1 to 12), = 0.10] on the Dimensionless Expected Mean (W0l) and Coefficient of Variation (Wl) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Pasternak (k1=100, k2=10) Elastic Foundations, Subjected to Uniform Constant Temperature and Moisture (U.T), in-plane Bi-axial Compression, Plate Thickness Ratio (a/h=20), with Simple Support S2 Boundary Conditions. The Dimensionless Mean Hygrothermal Deflections are given in Brackets. Load Deflection Q=100, Fiber Volume Fraction (Vf=0.6)
For the same lateral pressure and temperature distribution, it is seen that the COV of transverse central deflection becomes more important as the plate thickness decreases i.e., a/h increases (Table 3). It is seen that on variations of thickness ratio the mean (W0l) hygrothermal deflection increases whereas COV of hygrothermal deflection decreases with different combinations of input random variables when laminated composite plates resting on Winkler and for Pasternak elastic foundations hygrothermal deflection decreases further with different combinations of input random variables in Figure 6.
a/h |
(k1=100, k2=00) |
(k1=100, k2=10) |
||
|---|---|---|---|---|
Mean, W0l |
Mean, W0l |
|||
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
|
5 |
0.0126 |
0.0097 |
0.0304 |
0.0261 |
10 |
0.0278 |
0.0228 |
0.0314 |
0.0268 |
30 |
0.0377 |
0.0285 |
0.0338 |
0.0229 |
50 |
0.0411 |
0.0332 |
0.0351 |
0.0227 |
100 |
0.0449 |
0.0256 |
0.0364 |
0.0175 |
Table 3 Effects of Plate Thickness Ratios (a/h) with Random Input Variables bi, [{(i =1 to 9), (7..9), (10,11) and (12)} = 0.10] on the Dimensionless Expected Mean (W0l) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) Elastic Foundations, Subjected to Uniform Constant Temperature (U.T), and In-plane Bi-axial Compression with Simple Support S2 Boundary Conditions. Load Deflection Q=100, Fiber Volume Fraction (Vf=0.6)
In Table 4, it is noticed that on increase of aspect ratio the mean (W0l) hygrothermal central deflection value increases for Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) elastic foundations whereas COV of hygrothermal deflection decreases for all different combinations of input random variables, it is more dominant for Pasternak elastic foundations as shown in Figure 7.
a/b |
(k1=100, k2=00) |
(k1=100, k2=10) |
||
|---|---|---|---|---|
|
Mean, W0l |
Mean, W0l |
|||
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
|
0.5 |
0.0027 |
0.0024 |
0.0024 |
0.0019 |
1 |
0.0395 |
0.0308 |
0.0345 |
0.0223 |
1.5 |
0.1947 |
0.1314 |
0.1562 |
0.1049 |
2 |
0.5555 |
0.3814 |
0.4088 |
0.29 |
Table 4 Effects of Aspect Ratios (a/b) with Random Input Variables bi, [{(i =1 to 9), (7..9), (10,11) and (12)} = 0.10] on the Dimensionless Expected Mean (W0l) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) Elastic Foundations, Plate Thickness Ratios (a/h=40). Subjected to Uniform Constant Temperature (U.T), and In-plane Bi-axial Compression with Simple Support S2 Boundary Conditions. Load Deflection Q=100, Fiber Volume Fraction (Vf=0.6)
In Table 5 it is noticed that combined simple support and clamped support CSCS have significance effects on mean (W0l) hygrothermal deflection with different combinations of input random variables under environmental conditions. However the COV of hygrothermal deflection also varies accordingly under given environmental conditions and different combinations of input random variables for square composite plates resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) elastic foundations, mean and COV are significant forΔT =2000C, ΔC=0.02 Pasternak(k1=100,k2=10) elastic foundations as shown in Figure 8.
BCs |
(k1=100, k2=00) |
(k1=100, k2=10) |
||
|---|---|---|---|---|
Mean, W0l |
Mean, W0l |
|||
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
|
SSSS (S1) |
0.0426 |
0.0346 |
0.0358 |
0.0232 |
SSSS (S2) |
0.0426 |
0.0345 |
0.0357 |
0.0231 |
CCCC |
0.0423 |
0.0411 |
0.0353 |
0.0358 |
CSCS |
0.0408 |
0.0686 |
0.0391 |
0.0777 |
Table 5 Effects of Support Conditions with Random Input Variables bi, [{(i =1 to 9), (7..9), (10,11) and (12)} = 0.10] on the Dimensionless Expected Mean (W0l) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) Elastic Foundations, Plate Thickness Ratios (a/h=60). Subjected to Uniform Constant Temperature (U.T), and In-plane Bi-axial Compression with Simple Support S2 Boundary Conditions. Load Deflection Q=100, Fiber Volume Fraction (Vf=0.6)
In Table 6, it is observed that on change of lamina layup the mean (W0l) of hygrothermal central deflection decreases significantly for cross ply symmetric plate. The COV of hygrothermal central deflection also decreases for different combinations of input random variables. It is significant to note for cross ply symmetric plates with ΔT =2000C, ΔC=0.02 and Pasternak elastic foundations as shown in Figure 9.
Lay-up |
(k1=100, k2=00) |
(k1=100, k2=10) |
||
|---|---|---|---|---|
Mean, W0l |
Mean, W0l |
|||
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
|
(±450)2T |
(k1=100, k2=00) |
(k1=100, k2=10) |
(k1=100, k2=00) |
(k1=100, k2=10) |
(±450)S |
0.0335 |
0.0268 |
0.0302 |
0.0239 |
[00/900]2T |
0.0226 |
0.0178 |
0.0202 |
0.0156 |
[00/900] S |
0.0107 |
0.0088 |
0.0101 |
0.0082 |
Table 6 Effects of Lay-Up with Random Input Variables bi, [{(i =1 to 9), (7..9), (10,11) and (12)} = 0.10] on the Dimensionless Expected Mean (W0l) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) Elastic Foundations, Plate Thickness Ratios (a/h=50). Subjected to Uniform Constant Temperature (U.T), and In-plane Bi-axial Compression with Simple Support S2 Boundary Conditions. Load Deflection Q=100, Fiber Volume Fraction (Vf=0.6)
In Table 7, it is seen that on increasing load deflection the mean (W0l) hygrothermal central deflection increases in given environmental conditions and different combinations of input random variables .The central deflection value and COV of hygrothermal central deflection decreases in similar conditions and important to note for plates with ΔT =2000C, ΔC=0.02 and Pasternak elastic foundations as shown in Figure 10.
(Q) |
(k1=100, k2=00) |
(k1=100, k2=10) |
||
|---|---|---|---|---|
Mean, W0l |
Mean, W0l |
|||
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
|
100 |
0.0377 |
0.0285 |
0.0338 |
0.0229 |
150 |
0.0563 |
0.0476 |
0.0501 |
0.0392 |
200 |
0.0698 |
0.063 |
0.0616 |
0.0523 |
Table 7 Effects of Load Deflections (Q) with Random Input Variables bi, [{(i =1 to 9), (7..9), (10,11) and (12)} = 0.10] on the Dimensionless Expected Mean (W0l) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) Elastic Foundations, Plate Thickness Ratios (a/h=30). Subjected to Uniform Constant Temperature (U.T), and In-plane Bi-axial Compression with Simple Support S2 Boundary Conditions. Fiber Volume Fraction (Vf=0.6)
In Table 8, it is noticed that on varying fiber matrix volume fraction the mean (W0l) hygrothermal central deflection increases in given environmental conditions and different combinations of input random variables whereas the value of COV of hygrothermal central deflection also varies in similar conditions. It is to be noted for Pasternak elastic foundations and plates with ΔT =2000C, ΔC=0.02 as shown in Figure 11.
(Vf) |
(k1=100, k2=00) |
(k1=100, k2=10) |
||
|---|---|---|---|---|
Mean, W0l |
Mean, W0l |
|||
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
ΔT= 00C, ΔC=0.00 |
ΔT= 2000C, ΔC=0.02 |
|
0.50 |
0.0381 |
0.0289 |
0.0332 |
0.0209 |
0.55 |
0.0385 |
0.0296 |
0.0336 |
0.0213 |
0.60 |
0.0395 |
0.0308 |
0.0345 |
0.0223 |
0.65 |
0.0413 |
0.0327 |
0.0361 |
0.024 |
0.70 |
0.044 |
0.0355 |
0.0385 |
0.0265 |
Table 8 Effects of Fibre Volume Fractions (Vf) with Random Input Variables bi, [{(i =1 to 9), (7..9), (10,11) and (12)} = 0.10] on the Dimensionless Expected Mean (W0l) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) Elastic Foundations, Plate Thickness Ratios (a/h=40). Subjected to Uniform Constant Temperature (U.T), and In-plane Bi-axial Compression with Simple Support S2 Boundary Conditions. Load Deflection (Q)=100
In Table 9, it is noticed that on increasing temperature and moisture the mean (W0l) hygrothermal central deflection decreases in given environmental conditions and different combinations of input random variables whereas the value of COV of hygrothermal central deflection increases in similar conditions as noticed for plates with ΔT =2000C, ΔC=0.02 and Pasternak (k1=100,k2=10) elastic foundations as shown in Figure 12.
Environmental conditions |
(k1=100, k2=00) |
(k1=100, k2=10) |
|---|---|---|
W0l |
W0l |
|
ΔT= 00C, ΔC=0.0 |
0.0533 |
0.0488 |
ΔT= 1000C, ΔC=0.01 |
0.0486 |
0.0438 |
ΔT= 2000C, ΔC=0.02 |
0.0436 |
0.0388 |
ΔT= 3000C, ΔC=0.03 |
0.0384 |
0.0338 |
ΔT= 4000C, ΔC=0.04 |
0.033 |
0.0288 |
ΔT= 5000C, ΔC=0.05 |
0.0276 |
0.0238 |
Table 9 Effects of Temperature and Moisture Rise (ΔT, ΔC) with Random Input Variables bi, [{(i =1 to 9), (7..9), (10,11) and (12)} = 0.10] on the Dimensionless Expected Mean (W0l) of Hygrothermomechanically Induced Central Deflection of Angle Ply (±450)2T Square Laminated Composite Plates Resting on Winkler (k1=100,k2=00) and Pasternak(k1=100,k2=10) Elastic Foundations, Plate Thickness Ratios (a/h=20). Subjected to Uniform Constant Temperature (U.T), and In-plane Bi-axial Compression with Simple Support S2 Boundary Conditions. Load Deflection (Q)=150, Fiber Volume Fraction (Vf=0.6)
A C0 SFEM probabilistic procedure is adopted to compute the second order statistics of transverse central deflection of geometrically linear laminated composite plate in the framework of HSDT with randomness in material properties, coefficients of thermal expansion and coefficients of hygroscopic expansion, elastic foundation parameters and lateral loading.
Among the different system properties studied, the elastic moduli, elastic foundation parameters, lateral loading and environmental conditions have dominant effect on the COV of the transverse deflection when compared to other system properties subjected to uniform and linearly varying temperature distribution. In order to assess the effects of temperature and moisture on the bending behavior of shear deformable laminated plates, a theoretical analysis is developed based on a micro mechanical model.
The COV of the hygrothermomechanically induced transverse central deflection of the plate increases as distribution in lateral pressure increases, this bring out importance of considering hygrothermomechanical loading along with lateral pressure from design point of view specially in aerospace and other sensitive application where reliability of the components is important. Tight controls of these properties are therefore required for high reliability of the plate design. The flexural response of the laminated composite plate deteriorates considerably with the increase in temperature and moisture concentration and this hygrothermal environment becomes more detrimental as the working temperature reaches higher temperature.
(Aij,Bij,Dij,Eij,Fij,Hij)=∫h/2−h/2Qij(1,z,z2,z3,z4,z6)dz
; (i,j=1,2,6)
(Aij,Dij,Fij)=∫h/2−h/2Qij(1,z2,z4)dz
; (i,j=4,5)
[Kb]=n∑i=1∫A(e)[Bb(e)]T[Db][Bb(e)]dA
;
[Ks]=n∑i=1∫A(e)[Bs(e)]T[Ds][Bs(e)]dA
[KG]e=∫A[BNL]T{φ}dA=∫A[G]T[φ][G]dA
[Kf]=12∫A[Bf]T[Df][Bf]dA
{q}=NE∑e=1{Λ}(e)
[FT]=n∑i=1∫A(e)[[B1i(e)]T[NT]+[Bb1i(e)]T[MT]+[Bb2i(e)]T[PT]]dA
.
where
[Db]=[φi,x000000φi,y0000000φi,x000000φi,y0000000φi,x000000φi,y0000000C1φi,x0000000C1φi,y00000C1φi,yC1φi,x00000−C2φi,x0−C2φi,x00000−C2φi,y0−C2φi,y000−C2φi,y−C2φi,x−C2φi,y−C2φi,xφi,x]{q} ,
[Ds]=[00φi,x100000φi,x0100000−30−300000−30−3]{q}
[Bgi]=[00φi,x000000φi,y0000]
, [N0]=[NxNxyNxyNy]
,
ˉCijkl=[ˉQ11ˉQ12ˉQ1600ˉQ12ˉQ22ˉQ2600ˉQ16ˉQ26ˉQ6600000ˉQ44ˉQ45000ˉQ45ˉQ55]
ˉQ12= ˉQ21cos4α+2(Q12+2Q66)cos2αsin2α+Q22sin4αˉQ12= ˉQ21=(Q11+Q22−4Q66)cos2αsin2α+Q12(cos4α+sin4α)ˉQ16=(Q11−Q12−2Q66)sinαcos3α+(Q12−Q22−2Q66)sin3αcosαˉQ22=Q11sin4α+2(Q12+2Q66)cos2αsin2α+Q22cos4αˉQ26=(Q11−Q12−2Q66)sin3αcosα+(Q12−Q22−2Q66)sinαcos3αˉQ26=(Q11+Q22−2Q12−2Q66)cos2αsin2α+Q66(cos4α+sin4α)ˉQ44=Q44cos2α+Q55sin2αˉQ45=(Q55−Q44)sinαcosα−Q54ˉQ55=Q55cos2α+Q44sin2α
Where
Q11=E11(1−v12v21) ,Q12=v12E22(1−v12v21) ,=v21E11(1−v12v21) =Q21,Q22=E22(1−v12v21) , Q66=G12, Q44=G13, Q55=G12, v21=v12E22E11
None.
Author declares that there is no conflict of interest.

©2017 Kumar. This is an open access article distributed under the terms of the, which permits unrestricted use, distribution, and build upon your work non-commercially.