The behavior of laminar jet propane diffusion flames in the presence of moderate-strength permanent magnets has been investigated and the results of this experimental study are presented. While there are many ways of impacting combustion behavior, the impact of magnetic fields and their gradients has, to date, been underexplored. It has been previously recognized that the gradient magnetic field can influence laminar diffusion flames because of paramagnetic and diamagnetic components of the reaction zone of the flame. Using a magnet assembly of moderate-strength neodymium iron boron magnets mounted on an iron yoke, a non-uniform magnetic field was applied to the laminar jet flame. Propane/air flames with different flow velocities were produced from a 0.81mm burner port and the reaction zone was subjected to a gradient magnetic field. The experimental results show that the reaction zone exhibited a significant increase in local temperature under the influence of a decreasing magnetic gradient field. Compared with the case of the zero-magnetic field applied, the flame temperature increased by an average of 40K. In an increasing magnetic gradient field, the local temperature is expected to decrease, but it was found to increase slightly relative to the zero-magnetic field case. It was still found to be lower than the decreasing magnetic gradient field condition. It appears that the impact of the magnetic field is not strongly dependent on the burner tip position in the magnetic field gradient.

The energy associated with the magnetic influence on flame behavior is generally several orders of magnitude smaller than the kinetic energy of molecules at room temperature. Nevertheless, recent studies have shown that an inhomogeneous magnetic field provides a means to control combustion behavior. Gaseous combustion comprises of not only a chemical reaction but also of physical processes of heat transfer and mass diffusion. These processes can be manipulated using magnetic forces applied on paramagnetic gas flows to the flame. The motivation for this study is to look at the effects produced by a magnetic field of moderate-strength. The benefits of using permanent magnets include negating the need for external energy sources to produce high magnetic field strengths, as well as relatively less expensive. Magnetic fields are known to affect flame behavior and gas flows because of the paramagnetic and diamagnetic nature of the constituent gases. Paramagnetism is a form of magnetism whereby certain materials are weakly attracted by an externally applied magnetic field, and form internal, induced magnetic fields in the direction of the applied magnetic field. Paramagnetic materials include aluminum, oxygen, titanium, and iron oxide. In contrast with this behavior, diamagnetic materials are repelled by magnetic fields and form induced magnetic fields in the direction opposite to that of the applied magnetic field. Nitrogen, CO₂, and most hydrocarbon fuels are examples of diamagnetic materials and experience a weak repulsion to the applied magnetic field.

The magnetic susceptibility, the ratio of the magnetization to the magnetic field strength,¹ is the parameter that characterizes this behavior. All materials, to some degree, display diamagnetic behavior. For materials whose atoms possess permanent dipole moments, the associated paramagnetic forces are typically orders of magnitude larger and the diamagnetic behavior for these materials is thus negligible.² The magnetic susceptibility for a diamagnetic material is not a strong function of temperature and is negative in sign while a paramagnetic material has a positive magnetic susceptibility. A diffusion flame is used as a test subject to study the effect of the magnetic field since it simulates combustion characteristics found in various industrial applications. A diffusion flame is a flame in which fuel and oxidizer come together in a reaction zone through molecular and turbulent diffusion. The fuel may be in the form of a condensed medium (either solid or liquid) or in the gaseous form of a gaseous fuel jet, and the oxidizer may be a flowing gas stream or the quiescent atmosphere. It is the diffusion rate of the fresh mixture into the flame zone which sustains combustion and hence the name.

In diffusion flames, hydrocarbon fuels, nitrogen, carbon dioxide are diamagnetic; oxygen is the principal paramagnetic gas. As the paramagnetic susceptibility of oxygen is orders of magnitude larger, the diamagnetic behavior is considered as negligible. A gas containing more O₂, such as air, tends to move towards the stronger magnetic field and a gas with less O₂ such as fuel or combustion gas tends to move towards the weaker magnetic field. Based on this, it may be possible to utilize a magnetic field to control the flow field of the combustion region to improve combustion characteristics. A key parameter to characterize the laminar diffusion flame behavior is the flame height under the influence of the magnetic field.³ The flame height (Lf) is defined as the vertical distance between the burner surface and the point along the flame axis where the fuel is consumed in stoichiometric proportions.⁴ At present, magnetic control of combustion and gas flow with low-cost permanent magnets is a relatively new scope of research and further experimental study is required to establish the mechanism for this interaction. The specific objective of the study is to design an experimental set-up to measure a local representative temperature. In addition, the influence of magnetic forces to promote combustion compared to the normal buoyancy forces induced by gravity in diffusion flames is studied.

A large assembly of research has recently been carried out to study the effects of magnetic field on the behavior of flames to understand its mechanism. The effect was first recognized in 1847 when Michael Faraday⁵ held a flame of a wax taper with the magnetic field and observed its tendency to move into an equatorial position. He also observed that the flames were more luminous when placed with the magnetic field. He theorized that the changes were due to the presence of “magnetic” and “diamagnetic” gases in the flames. Over the past 30 years, there has been a renewed interest in the impact of magnetic field on combustion behavior. Hayashi⁶ investigated magnetic field effects on the emission intensities of intermediate species in premixed flames. He found that the magnetic field is seen to increase the combustion efficiency through the increase in the population of OH^* radicals. He thought that this change in the combustion process is due to the magnetically induced change of singlet-triplet conversion rate.⁷ Aoki⁸ rejected this theory for the gaseous combustion process since, in a gaseous phase, the lifetimes of a radical pair disappear before inverting the spins of excited states by S-T conversions.

Ueno S & Harada K⁹ investigated this behavior on candle flames. They noted that the presence of an increasing magnetic field gradient caused the flame to deflect in the direction of decreasing magnetic field strength. They came up with two reasons for this behavior; first, the charged particles in flame plasma made a current loop that tended to reduce the external magnetic field. Thus, flames escape from the magnetic field of higher intensity. Second, paramagnetic O₂ gases could be gathered by a magnetic field gradient and create a magnetically induced pressure to press back other diamagnetic gases and particles. To clarify this behavior, Ueno S & Harada K^10,11 expanded their research to study flames and jets of gas flow. Since the gas flow was also impacted by the magnetic field, the first hypothesis of charged particle theory was rejected. He also concluded that the presence of a strong magnetic gradient did not concentrate oxygen but aligned or trapped the molecules to make a “wall of oxygen” under the influence of magnetic gradients like an “air curtain” that pressed back flames and other gases. In 1990, Ueno S & Iwasaka M¹¹ studied the properties of air curtain and observed that the flow of CO₂ gas wasn’t blocked by the magnetic field in the N₂ atmosphere, which emphasizes the importance of O₂ as a paramagnetic gas in the formation of a magnetic curtain.

Aoki⁸ was the first one to investigate the effect of an inhomogeneous magnetic field under increasing, decreasing and uniform magnetic field gradients. In case of an upward decreasing field generated by electromagnets, he found that the presence of a magnetic field caused an increase in the flame temperature, emission intensities of radicals OH^*, CH₈ and C₂^* transitions and a bluing tendency of the flames. The author used a simplified diffusion model to explain the increase in flame temperature and flame dimensions in a diffusion flame; that they were mainly because of the effect of magnetic force. This magnetic force acting on the paramagnetic species changes the gas flow which affects the mixing and diffusion process conditions. Therefore, it is the changes in the actual physical processes that change the combustion state and not the chemical reaction itself.

On the other hand, the upward-increasing magnetic fields¹² increased the flame dimensions; they decreased radical emissions, temperatures and the bluing tendency. These phenomena were contrary to those observed in previous studies. He also investigated butane combustion under a uniform magnetic field encircled by a magnetic field.¹³ He observed no effect on combustion state, except for some changes that can be ascribed to the magnetic gradient produced by pole fringes. This exists for most of the magnets and is unavoidable. He showed that the velocities of electrons and ions produced by the combustion reaction are very low in the diffusive flame where the flow is dominated by buoyant force. Hence, the effect of Lorentz forces on the deformation of the flame was insufficient to cause any appreciable changes in flame within the magnetic field. Yamada¹⁴ supported this, as the amount of ionic species is negligibly small, and the influence of the Lorentz force can be ignored in ordinary flames. This explanation of magnetic force theory was also supported by Wakayama¹⁵ when she conducted experiments on O₂, N₂, a mixture of the two and air. The behavior of gas flow can be explained by the difference between the magnetic force acting on the gas group and the air surrounding it.

$F=(\chi -{\chi }_0)H\frac{\partial H}{\partial R}$

She explained that the air was attracted to the magnetic field which displaced the nitrogen gas from a magnetic field of relatively high intensity. This explanation also applies to the escape of flames from a high magnetic field gradient or magnetic quenching of flames observed by Ueno¹¹. Thus, the study demonstrates that it is the magnetic susceptibility of gas groups which exerts a net magnetic force that blocks the gas flow. Later, Wakayama^16,17 studied premixed, partially-premixed and diffusion methane flames within the magnetic field gradients. The results of these experiments brought to light a distinct thought process for the interaction of flames with magnetic fields. It was observed that a decreasing magnetic field along the flame caused its shape to be more elongated and slender, while an increasing magnetic field produced shorter and thicker flames. It was also observed that the temperature and luminosity of the flame increased with increasing gradient strength. These observations were attributed to the paramagnetic nature of oxygen and the diamagnetic properties of the products of combustion. She suggested two reasons for the magnetic promotion of combustion in diffusion flames. First, when fuel gas flows in the direction of decreasing field strength, the paramagnetic nature of oxygen increases the diffusion rate of air to the reaction zone and diamagnetic reaction products reject out efficiently. Accordingly, the burning velocity increases with increasing strength. Secondly, magnetic convection of air is also magnetically promoted because the gradient of temperatures causes the gradient of magnetic susceptibilities. The latter effect is less than the former one, comparing the difference of the magnetic susceptibilities in both cases.

However, no significant changes were observed for partially premixed flames and no effects on premixed flames, in either increasing or decreasing magnetic fields. Also, when the flames were moved to a homogenous field, the flames did not appear to be affected. Again in 2000, Mizutani¹⁸ found that premixed flames were hardly affected by a uniform magnetic field as intense as 5T. He also concluded that the direct effects of the magnetic field on the chemical kinetics of flame propagations are negligible. This suggested the convection of oxygen into the regions of increasing strength was the most dominant factor in affecting the combustion behavior. Hence, a diffusion flame is best suited to study the effect of a non-uniform magnetic field, as this combustion reaction occurs in the interface where the oxidizer and air react in stoichiometric proportions.

Diffusion flames are mainly governed by physical processes of mixing and diffusion of fuel gas and oxidizer. The rate of combustion is altered by magnetic field modifying the transport phenomena of oxidizer (Figure 1) to the reaction zones of such flames. Wakayama & Sugie¹⁹ suggested two kinds of air flow that promoted combustion in methane diffusion flames within 2cm gap of electromagnets. Air was magnetically attracted along the steepest gradient (solid lines) and supplied to the flame front. This makes flame front velocity three orders larger than the diffusion rate by natural physical processes. Furthermore, magnetic convective air flow occurred along the y-axis (dotted lines) feeding oxidizer to the leading edge of the flame because magnetic susceptibility of air is proportional to. Accordingly, the direction of magnetic force in decreasing magnetic field facilitates this combined movement of involved species to promote combustion in diffusion flames.

Yamada et al.,¹⁴ performed a numerical analysis of OH radical distributions in H₂/O₂ diffusion flames. It was observed that the magnetic gradient induced changes in the repartition of OH density in the flame. The effect is due to the enhancement of the entrainment gas flow around the flame base. This increases the mass density and then the magnetic susceptibility of oxygen in the peripheral region of the flame. To explore the possibility of combustion control by magnetic force, they²⁰ performed experiments using a co-axial type burner set between permanent magnets. Using spectroscopic techniques, they measured radial migration of OH towards the central axis of the flame as predicted numerically. Numerical simulations based on gas dynamics and magnetism showed that the magnetic force acts on the mean velocity of the gas mixture and not on the diffusion velocity of OH radicals. Hence, the magnetic effect is essentially due to magnetic force acting on O₂ and not directly on OH itself.

Baker et al.,²¹ studied the characteristics of slotted laminar jet diffusion flames in the presence of upward decreasing magnetic field produced by permanent magnets. The magnetic field decreased the flame height, increased the intensity of the flame, reduced the flow rate for which visible soot inception occurred, and increased the flow rate below which the flame extinguished. They provided a power-law fit between the experimental data and dimensionless parameters, which shows an inverse relation between magnetic body force and flame height. Khaldi²² investigated the phenomenon of thermos-magnetic convection due to the dependence of the O₂ magnetic susceptibility on temperature. They redefined the Rayleigh number as the results showed that heat transfer driven by magneto-gravity buoyancy is like that driven by gravity alone. Gillon²³ did extensive research on lift-off height of methane co-flow diffusion flames. Flame lift-off is referred to as the phenomenon in which the fuel mass flow rate exceeds a critical value and the flame and burner become separated. When the mass flow rate increases further, the lift-off height increases until the flame becomes flat and then blows out. The flame is said to be stabilized where the flame propagation speed with respect to the fuel/oxidizer matches the local flow velocity. Under the influence of an upward decreasing magnetic field, Gilard²⁴ observed a reduction in lift-off height. Magnetic force acting downwards on paramagnetic oxygen molecules decreases the air velocity locally. This modification of the external air mixing layer enhances the radial diffusion of methane and drops the stoichiometric line moving the flame front to a lower position. Kumar²⁵ & Aggarwal et al.,²⁶ studied the effect of magnetic field on the temperature profile of butane diffusion flame. The maximum flame temperature increased under an upward-decreasing magnetic gradient and decreased under an upward-increasing magnetic gradient, while a negligible effect on temperature was noted in a uniform magnetic field.

The experimental device is installed at the Reacting Flow and Turbulent Jets laboratory in the MAE department of NC State University. The components are designed and fabricated in a way to produce a laminar propane/air diffusion flame which is convenient for accurate temperature measurements. The experiments were carried out in the presence of a magnetic gradient field created by a permanent magnet placed around the burner system and the results are compared to a case of zero-magnetic field created using false magnets. All the components were made using non-magnetic materials to avoid any unwarranted attractions by the application of the magnetic field on the setup used. A picture of the experimental setup is shown in Figure 2 with the pertinent elements identified. The experimental setup is placed where the surrounding air remains relatively static, is mainly composed of the propane-air diffusion burner, yoke with the magnets, flow meter along with provisions for local temperature measurements.

The burner system is required to produce laminar flow conditions for the fuel gas issuing into the ambient air and to maintain a stable flame environment for temperature measurement required for this system. The fuel flow rate was regulated using a metering valve to ensure accurate results reproducible over multiple tests under similar conditions. A schematic representation of the burner is shown in Figure 3. The burner consists of a cylindrical base set on a stand through which a series of PVC fittings have been configured.

The burner setup consists of a fuel supply system and the diffusion burner. Propane gas was fed into the inner tube of 0.81mm inner diameter is selected for this investigation. Due to a burner length of 125mm, the gas flow is assumed to be fully developed at the exit of the burner, with a Poiseuille-type velocity distribution. The main purpose of the fuel supply system is to supply the combustion gases to the burner in controlled proportions. This supply system consists of compressed gas cylinders, flow meters, valves, and tubing, and can supply the fuel gases, oxidizer and others such as purge gases, both to a premixed and a diffusion burner configuration, present in the lab. The line supplying gases issues 99.0% pure CP grade propane (C₃H₈) was supplied from the cylinder to the diffusion burner. It flows through a Yor-Lok precision flow adjustment needle valve to govern the passage of the fuel gases and was monitored using volumetric flow meter (OMEGA, FMA-A2309) having a range of 0-10SLPM. The readings were estimated to be accurate within 5%. This range is sufficient for experiments to be performed over a wide range of jet velocities. Propane is injected at ambient temperature (i.e. 292.7K).

In this investigation, N52 grade permanent magnets (DX8C-N52) having a diameter of 37.6mm and a depth of 19.05mm is used to generate the gradient magnetic field. Two magnets, each of which could produce a pull force of 52.3Kg towards a steel plate are used in the current investigation. Placing two of these magnets in an iron yoke completes a path for the magnetic flux which enables a magnetic field whose strength is stronger than the individual strength of the magnets, to be formed between the poles of the magnet. Plain Iron Yoke design was selected based on a prior study done by Vikram²⁷ in the lab. This design is selected for its combination of a sufficiently high field and a reasonably big air gap and is fabricated. The yoke with the magnets is shown in Figure 4.

The magnetic field induction was measured along the vertical z-axis position using a Gauss meter with a transverse probe where the uncertainty of measurement was 1% and is shown in Figure 5. The influence of the magnetic field along the flame length is studied and hence the field distribution in the vertical direction is emphasized. Figure 5 also shows the specific locations of study employed in this experiment. The burner nose is positioned to be in two vertical positions A and B so that the flame experiences an increasing gradient magnetic field when the burner is set at position A and a decreasing gradient magnetic field when it is set at position B. The measurements of these cases were compared to a case of the zero-magnetic field.

Temperature measurements

The most critical element to this study is to identify the effect of non-uniform magnetic fields on the combustion processes occurring in the diffusion flame. The local temperature was monitored with a high-temperature Type K thermocouple probe, enclosed in a protection tube. Type K thermocouple has been chosen since it can accommodate temperatures up to 1523K. The temperature is measured at the highest point on flame tip along the z-axis for a given configuration. The thermocouple translation mechanism facilitates this movement for the different axial positions of flame tip for a given flow velocity as shown in Figure 1. The thermocouple is connected to the digital temperature reader (CL3515R), which is a portable thermometer with a 4-digit LCD. It is designed to be used with an external K Type thermocouple as temperature sensors. It has an accuracy of ± (0.05% rdg+0.5 K) -223 to 1645K. It includes DAQ (Data Acquisition) software to record the measurements and export the data in excel format.

Temperature correction for radiation losses

In a high-temperature environment, such as the hot-flame gases in the current burner arrangement, one major source of uncertainty in the measurement of local temperature is the correction for radiation. The temperature read by the thermocouple is less than the true value due to radiation heat transfer between the thermocouple surface and the surroundings. Under the assumption of steady-state temperature, an energy balance around the thermocouple bead may be written as the sum of conductive, convective, radiative and catalytic heat gain/loss:

$m_b{c}_p,b\frac{dT}{dt}=0={\dot{q}}_{concd}+{\dot{q}}_{conv}+{\dot{q}}_{rad}+{\dot{q}}_{cat}$

where m_b and c_p, b are the bead mass and the bead specific heat, respectively. Catalytic effects may be neglected a priori due to the choice of a K-type chromel/alumel thermocouple, whose materials can be considered non-reactive under the present conditions. Neglecting the conduction through thermocouple wires, the convective heat transfer between the thermocouple and the flame gases should be equal to net radiative heat exchange between the thermocouple bead and its ambient surroundings. Thus, the radiation correction has been considered. If T_m, the thermocouple temperature is the same through the thermocouple probe and that steady condition prevails, the derivation for the temperature correction is as shown below:

${\dot{q}}_{conv}=h_g{A}_{sph}(T_f-T_m)$

${\dot{q}}_{rad}=\epsilon A_{sph}\sigma (T_m{}^4-T_{\infty }{}^4)$

Where; T_f is the corrected local temperature (K), T_m is the measured temperature (K), by equating the above two equations the corrected temperatures were calculated by using the relation:

$T_f=T_m+\frac{\sigma \epsilon }{h_g}(T_m{}^4-T_{\infty }{}^4)$

The value of the convective heat transfer coefficient hg is approximated using the Nusselt number definition for a sphere immersed in a fluid²⁸ and given by the equations:

$h_g=\frac{k_g}{d}\left[2+0.6{\mathrm{Pr}}^{\frac{1}{3}}\left[\frac{ud}{v}\right]\right]$

This equation for h_g is valid for the case of forced convection from a sphere for Reynolds number in the range 1-70,000.²⁸

A set of experiments were carried out to determine the temperature distribution as a function of propane flow velocity within the flame. A propane/air diffusion flame was established for various fuel flow rates and the measured local temperatures were corrected for radiation losses. The measurements were carried out for three different cases; increasing magnetic field gradient, decreasing magnetic field gradient and when no magnetic field was applied. The temperatures were measured at the flame tip for each of the above cases. This represents the peak local temperature value along the central axis of the flame length. The thermocouple was positioned along the flames’ central axis and gradually positioned at the flame tip on the z-axis. For a circular port flame, the flame length does not depend on diameter but, rather, on the initial volumetric flow rate²⁹ so, the propane flow rate is varied to produce a flame whose height is within the influence of the magnetic field. This limited the maximum flow velocity for the experiments to 0.051m/s. The local temperature test values represent the average of ten measurements at each flow velocity with an estimated experimental error of ± (0.05% rdg+0.5 K). Further, the measured thermocouple temperature is corrected for radiation losses.

Case A: Zero-magnetic field

The variation in representative local temperature as a function of flow velocity is measured at the flame tip for the case of the zero-magnetic field. This is performed using an aluminum yoke instead of the magnetic yoke. Using a yoke to hold the magnets in place gives rise to two different impacts–a dynamic impact on the flow field and a magnetic impact. In this investigation, we consider only the magnetic impact on the flow field. To extract only the impact of the magnetic force on flames from the buoyancy force generated due to the presence of the yoke, a yoke made of aluminum possessing the exact geometry of the magnetic yoke is fabricated and all the baseline investigations are done in the presence of the aluminum yoke. The results are collected for flow velocities varying from 0.009 m/s to 0.051m/s. Figure 6 shows that local temperatures are underestimated by approximately 43.5 K due to radiation losses from the thermocouple tip (Table 1).

Case B: Increasing magnetic gradient field

The variation in highest local temperature with flow velocities in the presence of a gradient magnetic field whose strength was increasing from the burner tip is studied and is shown in Figure 7. It is observed that the local temperatures are underestimated by approximately 52K due to radiation losses from the thermocouple tip (Table 2).

Case C: Decreasing magnetic gradient field

The variation in local temperature in the presence of a gradient magnetic field whose strength was decreasing from the burner tip is studied and is shown in Figure 8. It is observed that the local temperatures are underestimated by approximately 52K due to radiation losses from the thermocouple tip (Table 3)

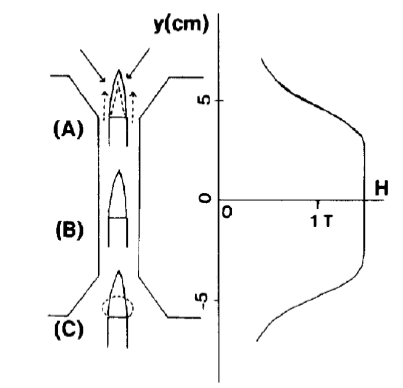
Figure 1 Direction of entrainment of air to flame under decreasing magnetic field (A).¹²

Figure 2 Experimental set-up.

Figure 3 Burner system.

Figure 4 Magnet assembly.

Figure 5 Actual magnetic field strength variation.

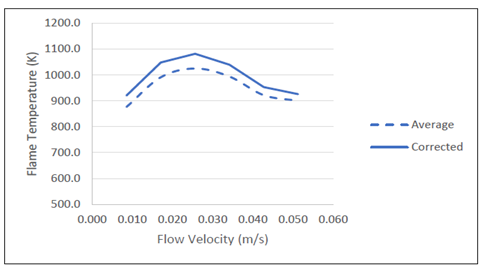
Figure 6 Variation of measured and corrected local temperature with flow velocity for zero-magnetic field.

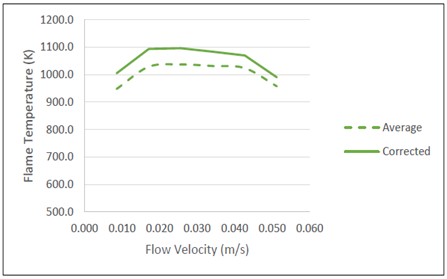
Figure 7 Variation of measured and corrected local temperature with flow velocity under increasing magnetic field gradient.

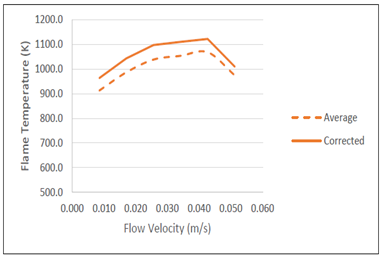
Figure 8 Variation of measured and corrected local temperature with flow velocity under decreasing magnetic field gradient.

The values of the local temperatures under the influence of an increasing and decreasing gradient magnetic field and no applied magnetic field for the 0.81mm burner can be found in Table 4.

Figure 9 shows the impact of the gradient magnetic field on local temperature as a function of flow velocity. We can see that the trend of local temperature with the variation of fuel flow velocity can be roughly divided into three characteristic regions.

1. Region I: This region is distinguished by an increasing behavior in the local temperature at the flame tip when the propane flow velocity is increased in all the three cases. It is noted that in this region the local temperature peaks for case B when the flame is under increasing magnetic gradient field.
2. Region II: This region represents a change in the trend of local temperature vs. propane flow velocity for each case. For the last two cases when a gradient magnetic field is applied, the rate of temperature rise slows down to almost approaching a flat line. However, for case A: zero-magnetic field, the temperature starts to drop sharply after peaking at 1082.1K for 0.26m/s.
3. Region III: This region expresses similar behavior for all the three cases, where the local temperature starts decreasing with any more increase in the propane flow velocity. It is in this region we observe the highest increase in the local temperature of all the three cases reaching up to 1122.3 K at 0.043 m/s, which is +40 K increase compared to the peak temperature in case A: zero-magnetic field. The local temperature behaves in a manner that is most favorable for the combustion of propane/air diffusion flame in case C when the flame is inside a decreasing magnetic gradient field.

It is significant that the application of the decreasing magnetic gradient field is accompanied by an increase in local characteristic temperature. In case C, by subjecting the flame to a decreasing magnetic gradient field, oxygen from the surrounding gas is drawn towards the flame via the magnetic force exerted. Thus, better utilization of the oxidizer implies better combustion. This aid enhanced burning conditions by replenishing reactants and removing hot product gases from the flame zone and thus leading to higher temperatures. A reverse effect is expected in the case B when the increasing magnetic gradient field is applied as the magnetic forces are in the direction that quenches the combustion processes in a diffusion flame. Although the peak temperature in case B was found to be lower than that compared to case C, the local temperatures measured are still higher than the corresponding values for case A: zero-magnetic field. This behavior was not consistent with a previously published theory for the magnetic promotion of combustion.²⁵ However, a similar anomaly was observed by Gillon³⁰ where it was observed that the magnetic gradient field.

This study examined the behavior of propane jet diffusion flames exposed to a low-strength gradient magnetic field. Based upon the results of this investigation, the following conclusions have been drawn:

1. The experimental data can be cast into three regions and is found to exhibit similar behavior within the regions for all the three conditions examined. When the propane flow velocity is low (region I), the local temperature shows an increasing behavior. As the velocity is increased (region II), the rise of local temperature slows down until it peaks and starts to decrease when the velocity is further increased (region III).
2. When the laminar propane/air diffusion flame corresponding to flow rates 0.009 m/s–0.051 m/s were studied, a non-uniform decreasing magnetic field was observed to have the most significant impact on combustion processes in the flame. For this condition, compared to flames with the zero-magnetic field, the local representative temperature increased by an average of 40K. Here, the magnetic force enhanced the supply of paramagnetic oxygen to the bottom and efficiently pushed the diamagnetic gases out the reaction zone. This behavior was consistent with previously published results.
3. In the case of increasing magnetic field, reverse effects were expected but the local temperature showed a slight increase of 15K relative to the case with the zero-magnetic field. It was still lower compared to that of a decreasing magnetic gradient field. This anomaly in flame behavior indicates a more complex interaction with the magnetic field or it could be an artifact of the small investigation area of the magnet assembly. When the burner tip is positioned for this case, there is still a possibility of decreasing magnetic gradient acting on the flame length from the upper half of the magnet assembly.

Future Work
There is scope for much future work that can be conducted further in this area of research to fully understand the complex nature of the interactions between magnetism and combustion:

1. To study the effect of different strengths of the magnetic field to identify the transition in flame behavior on the application of the varying external field. This can be done by employing compact permanent magnets of higher field strength compared to the one used in the current investigation to see a pronounced effect of the magnetic field.
2. The current investigation area was limited to the size of the magnet assembly employed. This can be expanded to extend the experiments as the current investigation was limited by the maximum flow velocity required to maintain the flame top inside the magnet assembly.
3. The study of flame-field interaction was altered by the intrusion of the thermocouple tip that can manipulate the temperature at the flame tip location. Although the radiation losses were considered and corrected temperatures were calculated, to improve the accuracy of the investigation, it would be helpful to use a non-contact type temperature measurement system.
4. To establish its commercial and industrial application, further design improvements can be performed. To ensure the temperature measurements are accurate and the magnetic field are unaltered by the heating of the magnets, an active cooling arrangement for the magnets and thermocouple will ensure the continuous working of the setup.
5. The current investigation can be taken further to study the behavior of flame in each of the three regions individually with respect to different base parameters like burner tip diameter, maximum field strength, co-flow air velocity and using different fuels etc.
6. It would be helpful to investigate the effect of the flow rate on the soot produced by the diffusion flame under the effect of magnetic gradient flame to gauge whether the concentration of soot will be altered. Thus, enhancing the combustion processes to burn the fuel supplied more completely relative to when there is no applied magnetic field.

Figure 9 Comparison of corrected local temperature for three different cases with flow velocity.

None.

The author declares that there is no conflict of interest.

1. Rosenwieg RE. Ferrohydrodynamics. New York: Cambridge University Press; 1985.
2. Boudreaux EA, Mulay LN. Theory and Applications of Molecular Paramagnetism. New York: Wiley; 1976.
3. Legros G, Gomez T, Fessard M, et al. Magnetically Induced Flame Flickering. Proc Combust Inst. 2011;33(1):1095–1103.
4. Fujita O, Ito K, Chida T, et al. Determination of Magnetic Field Effects on a Jet Diffusion Flame in a Microgravity Environment. Symposium International on Combustion. 1998;27(2):2573–2578.
5. Hayashi H. The external magnetic field effect on the emission intensity of the A 2Σ+ → X2Π (0-0) transition of the OH radical in flames. Chemical Physics Letters. 1982;87(2):113–116.
6. Hayashi H, Sakaguchi Y, Nagakura S. Chem. Letters (1980) 1149; Y. Sakaguchi and S. Nagakura, A. Minoh and H. Hayashi, Chem Phys Letters. 1981;82:213.
7. Ueno S, Esaki H, Harada K. Combustion Processes in Strong DC Magnetic Fields. IEEE Transactions on Magnetics. 1985;21(5):2077–2079.
8. Aoki T. Radicals' Emissions and Butane Diffusion Flames Exposed to Upward-Decreasing Magnetic. Japanese Journal of Applied Physics. 1989;28(5):776–785.
9. Ueno S, Harada K. Effects of Magnetic Fields on Flames and Gas Flow. IEEE Transactions on Magnetics. 1987;23(5):2752–2754.
10. Ueno S. Quenching of Flames by Magnetic Fields. Journal of Applied Physics. 1989;65(3):1243–1245.
11. Ueno S, Iwasaka M. Properties of Magnetic Curtain Produced by Magnetic fields. Journal of Applied Physics. 1990;67(9):5901–5903.
12. Aoki T. Radical Emissions and Anomalous Reverse Flames Appearing in Upward–Increasing Magnetic Fields. Japanese Journal of Applied Physics. 1990;29(1):181–190.
13. Aoki T. Radical Emissions and Butane Diffusion Flames Exposed to Uniform Magnetic Fields Encircled by Magnetic Gradient Fields. Japanese Journal of Applied Physics. 1990;29(5):952–957.
14. Yamada E, Shinoda M, Yamashita H, et al. Numerical analysis of a hydrogen-oxygen diffusion flame in a vertical or horizontal gradient of the magnetic field. Combustion Science and Technology. 2002;174(9):149–164.
15. Wakayama NI. Behavior of Gas Flow under Gradient Magnetic Fields. Journal of Applied Physics. 1991;69(4):2734–2736.
16. Wakayama NI. Effect of a Gradient Magnetic Field on the Combustion of Methane in Air. Chemical Physics Letters. 1992;188(3-4):279–281.
17. Wakayama NI. Magnetic Promotion of Combustion in Diffusion Flames. Combustion and Flame. 1993;93(3):207–214.
18. Mizuatani Y, Fuchihata M, Ohkura Y. Premixed Laminar Flames in a Uniform Magnetic Field. Combustion and Flame. 2001;125:1071–1073.
19. Wakayama NI, Masaaki S. Magnetic Promotion of Combustion in Diffusion Flames. Physica B: Condensed Matter. 1996;216(3-4):403–405.
20. Yamada E, Shinoda M, Yamashita H, et al. Magnetic Effect on OH radical distributions in a Hydrogen-Oxygen Diffusion Flame. International Joint Power Generation Conference; 2001. 139–144 p.
21. Baker J, Calvert ME. A Study of the Slotted Laminar Jet Diffusion Flames in the Presence of Non-Uniform Magnetic Fields. Combustion and Flame. 2003;133(3):345–357.
22. Khaldi F, Noudem J, Gillon P. On the similarity between gravity and magneto-gravity convection within a non-electroconducting fluid in a differentially heated rectangular cavity. International Journal of Heat and Mass Transfer. 2005;48(7):1350–1360.
23. Gillon P, Blanchard JN, Gilard V. Magnetic field influence on coflow laminar diffusion flames. Russ J Phys Chem B. 2010;4(2):279–285.
24. Gilard V, Gillon P, Blanchard CJN. Effects of Magnetic Field on the Stabilization of a Lifted Diffusion Flame. In Proceedings of the European Combustion Meeting; 2009. 5 p.
25. Kumar M, Agarwal S, Kumar V, et al. Experimental investigation on butane diffusion flames under the influence of magnetic field by using digital speckle pattern Interferometry. Appl Opt. 2015;54(9):2450–2460.
26. Shilpi Agarwal, Manoj Kumar, Chandra Shakher. Experimental investigation of the effect of magnetic field on temperature and temperature profile of diffusion flame using circular grating Talbot interferometer. Optics and Lasers in Engineering. 2015;68:214–221.
27. Vikram Ramnath. Investigation of the Impact of Low Strength Gradient Magnetic Fields on Jet Diffusion Flames. Theses and Dissertations, NC State University Repository; 2017.
28. Incropera FP, Dewitt DP. Introduction to Heat Transfer. 3rd ed. New York: John Wiley and Sons; 1996. 478–479 p.
29. Turns S. An Introduction to Combustion: Concepts and Applications. 2nd ed. Mc-Graw Hill; 2000.
30. Gillon P, Blanchard JN, Gilard V. Methane/Air-Lifted Flames in Magnetic Gradients. Combustion Science and Technology. 2010;182(11-12): 1805–1819.