MOJ
eISSN: 2574-9722
Submit manuscript...
Opinion Volume 1 Issue 1
Pulfrich effect for vision therapy
Qamil Kllogjeri,1
Verify Captcha
Regret for the inconvenience: we are taking measures to prevent fraudulent form submissions by extractors and page crawlers. Please type the correct Captcha word to see email ID.
Pellumb Kllogjeri2
Verify Captcha
Regret for the inconvenience: we are taking measures to prevent fraudulent form submissions by extractors and page crawlers. Please type the correct Captcha word to see email ID.
1Department of IT Engineer, At Streym Ltd, UK
2Department of Mathematics, University of Elbasan, Albania
Correspondence: Pellumb Kllogjeri, Department of Mathematics, University of Elbasan, Albania
Received: May 09, 2017 | Published: May 12, 2017
Citation: Kllogjeri Q, Kllogjeri P. Pulfrich effect for vision therapy. MOJ Biol Med. 2017;1(1):22–23. DOI: 10.15406/mojbm.2017.01.00006
Abstract
Our work is part of the world-wide and ongoing efforts of GeoGebra and other technology communities to increase the pace of change in development of new tools to facilitate and faster the doing of mathematics, the teaching and learning of mathematics, and applications of software.1 The article is result of the research done with regard to the applications of mathematics in special directions such as: solving problems of physics, biology and astronomy by using technology as a bridge. With GeoGebra can be performed lot of simulations of physical phenomena from Mechanics discipline and solved computational problems.2 Our main focus is the vision problems derived from the weakening of the eye muscles, although they are very strong. Our opinion is that it is possible to recover or strengthen the eye muscles by the use of computer-based exercises.
Keywords: vision therapy, simulation of physical phenomenon, geogebra software, virtual environment
Introduction
Vision therapy is a physical therapy, a non-surgical treatment for many common visual problems such as lazy eye, crossed eyes, double vision, convergence insufficiency and reading and learning disabilities. It works on the eyes and visual system. In vision therapy can be used prisms, eye patches, filtered lenses etc. In the present time are used computerized systems to conduct therapy sessions. There are progressive programs of vision exercises or procedures that help to fit the visual needs of the patient. The computer-based exercises for impairing the vision can be conducted at home or in office, in short sessions. Our paper is concentrated in the vision problems derived from the weakening of the eye muscles, although they are very strong. Our opinion is that it is possible to recover or strengthen the eye muscles by the use of computer-based exercises. One special and very comfortable mean is GeoGebra software.1 The use of GeoGebra and other programs relates to the massive and global use of computers and cell phones irrespective of the age. Consequently, the need for muscle re- education to restore vision function is grown dramatically. The computer-based exercises help to improve fundamental visual skills and abilities, improve visual comfort, ease, and efficiency. There are a number of studies using computer games to aid in the rehabilitation of individuals with both neuromuscular and musculoskeletal disorders with the majority focusing on upper limb rehabilitation.3–5
We address here the use of Pulfrich Effect as a game for home or office-based vision therapy is targeting a common sensory and neuromuscular binocular eye movement disorder known as convergence insufficiency. This treatment relies on exercises that require repeated convergence of the eyes by following the movement of the pendulum. We are of the opinion that the use of an interactive virtual medium, provided by GeoGebra software, will make therapy more fun and encourage the patients to persist in completing their prescribed therapy with successful outcomes.
Visualization of pulfrich phenomenon with geogebra
The Pulfrich phenomenon or effect is a psychophysical perception related to the apparent spatial disparity between the two eyes when the sight of one of the eyes is purposely passed through a filter. The effect was observed in an experiment with a swinging pendulum that was first performed in 1922 by a German physicist called Carl Pulfrich.6 The classical demonstration of the Pulfrich Effect is as following: The pendulum is swung back and forth and its movement is observed with both eyes. Care must be shown that the movements of the pendulum stay in the same plane parallel to the viewer’s forehead, which is making the observation. The meaning of this is that the swinging be in the plane which is vertical to the observer’ sight rays (the trace of the pendulum is a circular part lied in this plane), in other words: not in a way that the pendulum shifts near and far of this plane (in this case its trace is a circle that cuts the plane). When viewed normally with both eyes, the pendulum just seems to swing back and forth. When a filter is placed in front of one eye, the pendulum suddenly seems to be swinging in an ellipse parallel to the floor. In other words, the pendulum should appear to be tracing an oval path. This is an optical illusion. Dimming the light equally to both eyes does not cause the illusion in (Figure 1).
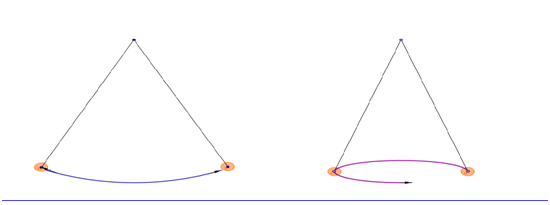
Figure 1 The real path of the pendulum is in a plane (left). The Pulfrich pendulum appears to move in an elliptical path, toward and away from the observer (right).
The Pulfrich Effect, which happens when a filter is placed in front of one eye, is an optical illusion in which an object which is moving in a plane parallel to the viewer's forehead seems to move out of the plane mentioned above. It looks as if the pendulum approaches (or recedes from) the viewer. This illusion is stereoscopic: it occurs only when the moving object is being viewed with both eyes and a filter is placed in front of one eye.6 Pulfrich was never able to observe the phenomenon because he was blind in one eye. He explained the phenomenon without observing it. For this reason, this phenomenon bears his name.
Explanation of pulfrich phenomenon
The placement of the filter in front of one eye (usually it is a neutral density filter) is "causing a time delay for the neural signal in the eye".7 Therefore, the perceived position of the swinging pendulum in the filtered eye falls” slightly behind that in the other eye for a given moment, creating this way a spatial offset between what is perceived in the two eyes".7 In the case, when the right eye is covered by the filter: when the pendulum swings from left to right, the "spatial offset corresponds to a binocular disparity that shifts the perceived depth of the pendulum towards the observer " and, when the pendulum swings from right to left, the "spatial offset corresponds to a binocular disparity that shifts the perceived depth of the pendulum away from the observer ".7 As said earlier, the target which is oscillating at eye level in a front parallel plane appears to rotate out of its oscillating plane when a coloured filter is placed in front of one of the observer's eyes. Pulfrich (following a suggestion by Fertsch) accounted for the depth displacements in terms of a difference in the hypothesized visual latent periods of the two eyes. The visual latent period of each eye was assumed to be a reciprocal function of the level of retinal illumination. Thus, the eye covered with the filter presumably "signals" a position of the oscillating target that lags behind the position that was signalled by the uncovered eye. Lit, Alfred and others have studied the effects of conditions of illumination of visual latency, measured by several different monocular and binocular experimental procedures, in which the magnitudes of the far and near displacements of the Pulfrich stereo-phenomenon were measured at various levels of illumination.8 The size of these shifts depends upon how fast the object is moving. A swinging pendulum moves at its fastest in the middle of the swing (the minimum point of the circular trace), and comes to a momentary stop at the ends, where it changes direction. Consequently, when viewing a pendulum with one eye dimmed, the apparent distance shift will be greatest in mid-swing and zero at the ends of the swing. The result is that the pendulum seems to follow an elliptical path. The applet of demonstration of the Pulfrich effect is created using GeoGebra software, and it can be downloaded from the wiki. Visiting the link, http://www.geogebratube.org/student/m30375, you can watch the two phases of the pendulum swinging, even with repetitions. The path is almost elliptical.9,10
Acknowledgements
None.
Conflict of interest
The author declares no conflict of interest.
References
- Hohenwarter Judith, Hohenwarter Markus. Introduction to Geo Gebra. 2008. p. 3–20.
- Hohenwarter M, Preiner J. Dynamic mathematics with Geo Gebra. The journal of online mathematics and its applications 7; 2007.
- Leder R, Murillo N, Ibarra C, et al. Computer game motivating rehabilitation with objective measures of improvement in motor function, in 2001 Proceedings of the 23rd Annual engineering in medicine and biology society international conference; Turkey: IEEE; 2001. p. 1388–1390.
- Elk M, Driessen B. Active rehabilitation of cva patients in IEEE international conference on systems; Man and Cybernetics, Netherlands: IEEE; 2004. p. 2449–2453.
- Betker A, Szturm T, Moussavi Z. Development of an interactive motivating tool for rehabilitation movements,” in Proceedings of the 2005 IEEE engineering in medicine and biology 27th annual conference; China: IEEE; 2005. p. 6893–6896.
- Morgan MJ, Thompson P. Apparent motion and the Pulfrich effect. Perception. 1975;4(1):3–18.
- Anzai A, Ohzawa I, Freeman RD. Joint-encoding of motion and depth by visual cortical neurons: neuronal basis of the Pulfrich effect. Nat Neurosci. 2001;4(5):513–518.
- Lit Alfred. The magnitude of the Pulfrich stereophenomenon as a function of target velocity. Journal of Experimental Psychology. 1960;59(3):165–175.
- http://pulfrich.siu.edu/Pulfrich_Pages/explains/expl_txt/explaint.html
- http://www.geogebratube.org/student/m30375
©2017 Kllogjeri, et al. This is an open access article distributed under the terms of the, which permits unrestricted use, distribution, and build upon your work non-commercially.