Shape is a fundamental morphological descriptor, one method of its estimation being from digitally processed images. Elliptic Fourier method is an outline-based morphometrics that has some advantages: it does not require either landmarks or previous knowledge about the shape variation of the objects under study; it can visualize the contour of information and reconstruct the original shape; it can be mathematically normalized to size, rotation and starting point of the contour trace; and it can be conducted automatically using computer software. Hence, the variation could be decomposed into several mutually independent quantitative characteristics. In this manner, unacceptable errors based only on human visual judgment of shape, which is frequently deceptive and misled by size factors, can be effectively eliminated. The present study evaluated the shape of equine distal sesamoids (“navicular bone”) in 28 feet belonging to “Cavall Pirinenc Català” by means of Elliptic Fourier methods. No allometric relationship (low coefficient of regression) was demonstrated between area and form of the bone. At the same time, different morphological patterns appeared. Shape differences were centred on the proximal ridge, which moved from a crescentic, oval lucency, slightly oval, to a flat contour, and no concave ridge was detected. So breed does not seem to be very prone to suffer from navicular diseases, as some authors have stated that there is an apparent predisposition according to shape of the proximal articular border. These results provide a baseline to further examine shape quantification in sesamoid morphology.

Keywords: cavall pirinenc català, image analysis, navicular bone, outline fitting functions

PCA, principal component analysis; EFA, elliptical fourier analysis; FDs, fourier descriptors; CPC, catalan pyrenean horse; NPMANOVA, non-parametric-multivariate-analysis-of-variance

Horses have a distal sesamoid bone, which is located within the hoof and lies between the middle phalanx and distal phalanx. This sesamoid is known as the “navicular bone”. The navicular bone has the physical shape of a small canoe, which led to the name "navicular" bone, the prefix "navicu" meaning "small boat" in Latin. The bone is elongated transversely and articulates with both the distal and middle phalanx, lying palmar to the distal interphalangeal joint. The palmar flexor surface of the bone is characterized by a prominent sagittal ridge, which is covered by fibrocartilage and provides a smooth surface for the deep digital flexor tendon to glide during weight bearing. Its distal border contains a small articular facet of hyaline cartilage for articulation with the distal phalanx.

The region that the distal sesamoid bone occupies is an important structure in relation to equine lameness, responsible for as much as one third of all cases of lameness, called “navicular syndrome”, which affects the front feet in particular.¹ Newer imaging techniques have shown that damage to the soft tissues in the region may be a significant contributor to lameness, so variations in “normal” anatomy exist and some authors describe important differences in its proximal border, the site of origin of the collateral sesamoidean ligament (“suspensory ligament”). This is why good image assessment of the distal sesamoid is quite important.

The shape of the proximal articular border of the navicular bone outline on its dorso-palmar view is classified 1-4 according to Dik and van den Broek:² 1=concave; 2=undulating; 3=straight; 4=convex.³ Dik & van den Broek’s² findings indicate an apparent shape predisposition to radiological changes associated with navicular disease, shape 1 showing the highest prevalence, therefore representing the poorest shape conformation, and shape 4 reflecting the least susceptible shape. Therefore, the clinical analysis of distal sesamoid must consider a complete quantification of the form, but accurate, quantitative measurements of its form are not easy to assess and a few papers include it, such as those by Salinas et al.,⁴ and less information exists regarding the manner in which this form changes as the horse ages.

The shape of the distal sesamoid design is an irregular curved structure, so the use of the conventional metrical approach, consisting of distances, angles and ratios, is difficult to apply effectively. Using the geometric morphometric approach, shape variability can be studied as a geometric property without any effect of size. An approach for analysing the closed outlines of an object is Elliptical Fourier Analysis (EFA), developed by Kuhl and Giardina,⁵ which transforms the data from the spatial domain into the frequency domain, allowing the detection of small variations in shape and the evaluation of an object’s shape independently of the size. The technique is ideally suited for characterizing the shape of complex morphologies.⁵

EFA is based on the production of Fourier functions (harmonics) to analyse the outlines of an object. Its function is defined as a parametric formulation in the form:  allowing the separation of the boundary contour into separate x- and y- components. These parametric functions consist of a pair of equations (x and y) derived as functions of a third variable (t), where n equals the harmonic number. Fourier Descriptors (FDs) allow the analysis of contours that cannot be simply represented as single-valued functions (a requirement with conventional Fourier analysis). Another attractive property of FDs is that they not only allow the precise reconstruction of the biological outline, but also permit the re-creation, at any time, of the form from the coefficients obtained, in the absence of the original specimen. FDs have been successfully applied to a number of morphological structures.⁶

Therefore, our objective was to describe a quantitative measurement of the dorsal surface of the equine navicular bone using Elliptical Fourier Analysis (EFA). Although, as said previously, there were some former studies, this is the first successful research to quantitatively evaluate this distal bone shape.

Specimens

Twenty-eight dissected distal sesamoids (forefeet) belonging to “Cavall Pirinenc Català” of unknown sex were studied. Animals were sacrificed in a commercial abattoir for commercial purposes not related to the object of this study. They were <24months and presented no apparent lameness on entering the slaughterhouse.

The “Cavall Pirinenc Català”/Catalan Pyrenean horse (CPC) is a breed raised for meat production in the harsh environment of the North-eastern part of the Pyrenees along the Spanish-French border.⁷ It is a compact, broad-built, predominantly chestnut horse with rather short limbs.⁸ Genetic analysis suggests that this small population of CPC horses (<4,600)⁹ is closely related to the Breton and Comtois breeds.¹⁰ The CPC horses are reared outdoors throughout the year and do not receive any additional food besides some low-quality straw in winter. When young horses are selected for slaughter, they are gathered in large paddocks and receive additional feeding with hay and concentrates during the last months before slaughter. Animals of this breed range all year round over pastures and do not receive any hoof care, trimming, or shoeing. Therefore, their hooves must be considered naturally shaped but, remarkably, hoof wall problems are quite rarely encountered in this breed.¹¹

Extraction of the outlines and FDs

Each distal sesamoid was obtained by dissection, cleaned and dried, and then hand-drawn on its palmar surface in a standard orientation procedure, so the shape corresponded to the view of the bone when dorso-palmar radiographic projections are performed (proximal edge up, distal edge down). Then, drawings were scanned and introduced as a BMP image. Finally, geometric information of the shape was transcribed using the SHAPE® software package,¹² which recorded the scanned images and generated the elliptical Fourier description for each sample. Briefly, the procedure was as follows: each colour image was converted into a binary image (i.e. transformed into white for the bone outline and black for the background, in pixels), so the outlines of each continuous contour (interface between the black and the white pixels) were automatically traced and digitalized; each outline was transcribed in chain-code; the area enclosed in each outline was also automatically calculated. An average of 1,530 points were positioned along the outline of each bone. The size and orientation of each contour were standardized by the first harmonic ellipsis method and outlines reduced to the coefficients of the EFA a_n, b_n, c_n and d_n of 20 harmonics [(4x20)]. Each harmonic corresponded to the four coefficients defining the ellipse in the xy-plane. The final shape was approximated by 20 harmonics, which corresponded to 80 Fourier coefficients, whereupon the coefficients effectively became shape variables. These coefficients are mathematical descriptors of forms,⁵ so they were statistically analysed. For more detailed information on this software, we refer readers to the help file of this program.

Multivariate analysis

Data were screened for outliers (specimens with relatively unusual morphologies compared to other specimens) using univariate and multivariate exploratory statistical techniques (box. plot for size, PCA for shape, and cluster analysis for both). Outliers could appear as having large sizes relative to the mean (size box-plot), lied outside 95% confidence interval (PCA shape scatter plot), or as isolated specimens in cluster diagram (size+shape phenogram).

As SHAPE® adjusted for size and orientation, the first harmonic (H₁) does not contain morphologic information,¹³ so only seventy-six [(4x20)-4] (H₂ to H₂₀) standardized Fourier descriptors were finally used for the outline analysis. A regression was conducted, using area (log transformed) as independent, and coefficients as dependent variables. As regression has a number limit for dependent variables, only seven first harmonics (H₂ to H₈) were considered. The K-means clustering, a non-hierarchical clustering method, was undertaken, selecting “a priori” four clusters (as stated before, Dik and van den Broek² recognized four groups according to navicular shape).

In practice, one uses a number of harmonics less than infinity for the summation. Therefore, one tries a range of harmonics and settles on a manageable number that adequately represents the original curve, so additional harmonics do not increase the accuracy of the reconstruction. This is why, subsequently, a Principal Component Analysis (PCA), performed on the covariance matrix, was carried out, allowing the maximization of harmonics’ variance which permitted the optimal rigid axes rotation to be found, axes being the PC. Geometric interpretation for each PC was assessed from the reconstructed contours using inverse Fourier transforms. Finally, a NPMANOVA (Non-Parametric-Multivariate-Analysis-Of-Variance) was used to assess morphological differences between detected groups, using Euclidean distances and only effective PCs. All statistic treatments were performed with the PAST® package.¹⁴ The significance level was established at 5%.

Outliers

The original data set was not reduced as no potential outliers appeared.

 Regression on area

A low coefficient of regression of area vs shape appeared (R²=0.02, Wilk’s λ=0.083), so no allometric change of sesamoid pattern could be supposed along growth of animals.

Quantitative analysis of the elliptical fourier descriptors

The number of effective PCs was nine, which explained 94.5% of the total variance observed Table 1. Summary statistics¹⁵ of the elliptical Fourier descriptors of the first nine harmonics for the size-normalized sesamoid outlines studied are listed in Table 2. Differences were centred on the proximal ridge, which moved from a crescentic (n=4), oval lucency (n=4), slightly oval (n=7), to a flat proximal contour (n=10) (Figure 1). NPMANOVA showed significant differences between all these groups (p≤0.0035).

Cluster 1 Straight proximal contour.

Cluster 2 Crescentic.

Cluster 3 Oval lucency.

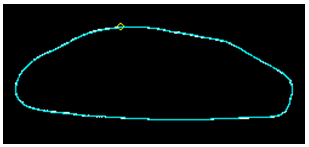
Cluster 4 Slightly oval.

Figure 1 Patterns for the four groups obtained in distal sesamoids (forefeet) belonging to “Cavall Pirinenc Catala” (dorsopalmar views). Differences are centred on the proximal ridge, which moves from straight proximal contour (cluster 1), crescentic (cluster 2), oval lucency (cluster 3), to slightly oval (cluster 4). Significant differences appeared between them (p≤0.0035).

The outlines employed in the present study turned out to be an example of data that are very well-suited for a particular analysis. Moreover, the retained variables defined the shape independently of the size. In this case, the morphologic variations of navicular bone were well quantified by geometric variables obtained from 2D image analysis of their outlines.

There are clear differences between breeds in the prevalence of radiographic changes that fit in with the syndrome of navicular disease:³ in a study on normal Dutch Warmbloods,² the average prevalence’s for 3 and 4 grades were 16%, for shape 1-those of highest prevalence-, was 36%, and for 4-that of lowest prevalence- was 9%. In cadaver forefeet of Finnhorses, a breed where navicular disease is a rare diagnosis, shape 1 prevalence was 11% and shape 4 was 38%.¹⁶ Moreover, in horses, there is a hereditary element in navicular bone shape.²

On the dorso-palmar view, the proximal contour of the flexor cortex of the navicular bone in CPC ranges from crescentic-shape 4- to straight proximal border-shapes 2 and 3-, with no concave ridge-shape 1- detected. Therefore, if a shape-dependent distribution of the biomechanical forces exerted on the bone exists,² the conformational determinants of susceptibility for the development of navicular disease in CPC could then be determined. Moreover, in view of the results obtained in this study, this breed does not seem to be very prone to suffer from navicular diseases.

Being the navicular bone a 3D object, it is obvious that 2D pictures will inevitably imply a loss of information and a degree of inaccuracy. Mathematically this is because 2D and 3D shapes will be far apart in the space because of the third Z dimension (“thickness”), which is in fact present only in the real 3D information. But the outline of the navicular bone introduce very small two to three dimensional approximation, as long as the points used to measure form are on the same plane (e.g. proximal and distal edges, like a margin of a bivalve shell). If this difference will be a loss of information it may not represent a problematic source of inaccuracy. So, 2D data accurately capture the relative shape differences among individuals. Moreover, the dorso-palmar radiographs of the bone, on which clinical diagnosis rely, will capture the same specific 2D aspect of variation, and for both image sources loss of information will affect in a similar fashion.

The limit to sample size has been set by the time and effort available for data collection, but also by the availability of specimens in abattoir. We must recognize that in this study sample size was too small for a robust assessment of a morphological distinctiveness of the equine navicular bone in the breed. This is why it is fundamental to understand that our study should be seen as preliminary and in need of confirmation using a larger sample. Evidently, more research including more animals with a wider age spectrum should also be undertaken.

The authors thank the abattoir MAFRISEU SA in La Seu d’Urgell (Catalonia, Spain) for providing the samples. The study conception, samples preparation and data acquisition were performed by Lozano, and statistical analysis by Parés-Casanova. Both authors contributed equally to data interpretation, writing, and revision of the manuscript and to the final approval of the version submitted.

Author declares that there is no conflict of interest.

1. Wintzer HJ. Maladies du cheval. Maloine. Paris; 1991.
2. Dik KJ, van den Broek J. Role of navicular bone shape in the pathogenesis of navicular disease: a radiological study. Equine Vet J. 1995;27(5):390‒393.
3. Dik KJ, Van den Belt AJM, Broek VD. Relationships of age and shape of the navicular bone to the development of navicular disease: a radiological study. Equine Vet J. 2001;33(2):172‒175.
4. Salinas P, Figueroa S, Carrasco C, et al. Morphometry, Planimetry and Stereology in the Distal Sesamoid Bone in Equine Foot with and without Navicular Syndrome. Int J Morphol. 2014;32(1):357‒363.
5. Kuhl FP, Giardina CR. Elliptic Fourier features of a closed contour. Comput Graph Image Process. 1982;18(3):236‒258.
6. Younker JL, Ehrlich R. Fourier biometrics: harmonic amplitude as multivariate shape descriptors. Syst Zool. 1977;26(3):336‒342.
7. Fernandez M, Gomez M, Delgado JV, et al. Guia de Campo de las Razas Autoctonas Espanolas. Madrid, Spain: Serga; 2009.
8. Pares PM, Pares R. Algunas características faneropticas del caballo Breton Ceretano. Av Alim Mejora Anim. 1997;37(4‒5):53‒57.
9. Infante JN. Caracterizacion estructural de las explotaciones equinas de carne del Pirineo Catalán. Caracterizacion Morfologica de la Raza: Caballo Pirenaico Catalan. Barcelona, Spain: Tesina de Investigacion. Univ. Autonoma de Barcelona. 2008.
10. Infante JN, Ferrando A, Pares PM, et al. Estructura genetica poblacional en la raza equina Cavall Pirinenc Català (CPC). Su relacion con otras razas carnicas espanolas y la influencia de razas pesadas francesas. Gijon, Asturias, Espana: Libro de Comunicaciones VII Congreso Iberico sobre los Recursos Geneticos Animales. 2010;66.
11. Pares PM. A nonlinear model for estimating hoof surface area in unshod meat-type horses. J Equine Vet Sci. 2011;31(7):379‒382.
12. Iwata H, Ukai Y. Shape: A computer program package for quantitative evaluation of biological shapes based on elliptic Fourier descriptor. J Hered. 2002;93(5):384‒385.
13. Crampton JS. Elliptic Fourier shape analysis of fossil bivalves: some practical considerations. Lethaia. 1995;28(2):179‒186.
14. Hammer O, Harper DAT, Ryan PD. PAST: Paleontological Statistics Software Package for Education and Data Analysis. Pal Electron. 2001;4(1):4‒9.
15. Davis JC. Statistics and Data Analysis in Geology. New York, USA: John Wiley & Sons; 2002.
16. Ruohoniemi M, Ryhanen V, Tutamo RM. Radiographic appearance of the navicular bone and distal interphalangeal joint and their relationship with ossification of the collateral cartilages of the distal phalanx in Finnhorse cadaver forefeet. Vet Radiol Ultrasound. 1998;39(2):125‒112.