Four methods are currently used for well test data interpretation in hydrocarbon-bearing formations which are conventional straight line, type curve-matching, non-linear regression (computer assisted) and TDS technique. The first is a very important method but has two main drawbacks: difficult to define a given region or flow regime and requires one plot for each flow regime. The second one is basically a trial-and-error method, and it is tedious and risky. It requires hundreds of type curves. The third one requires expensive computer software and involves none uniqueness of the solution; it is also the most used and misused worldwide. And the last one is very versatile, practical and self-verifiable. This short paper deals with the importance and application of this last technique which is not very much used in the hydrocarbon industry, maybe, due to a conservative tendency or ignorance of it. More than 200 hundred publications and a couple of books have been published on this issue. Impact of such publications is very low, though.

Keywords: well test, pressure derivative, TDS technique, permeability

k, permeability (md); h, reservoir thickness (ft); hp, length of perforations (ft); n, flow behavior index (power-law parameter); P, pressure; psi, pressure in laplace space; r, radius (ft); rw, wellbore radius (ft), t, time (hr); ℓ, laplace parameter suffices; D, dimensionless; v, vertical

TDS technique¹ was introduced in 1993. It is a modern tool to analyze pressure tests using characteristic points and lines found in a pressure change and pressure derivative log-log plot that allows the direct obtaining of the parameters of the well and the reservoir without using adjustment by type-curve matching using direct analytic expressions, instead. It is used by all commercial software without recognizing the appropriate name of the technique. For instance, Spivey and Lee² call it “Manual Log-Log Analysis” without given the appropriate references and they recommend the technique.

TDS technique is a very practical and accurate analytic tool. It is applied to many several reservoir conditions and well configurations. Because of the power of this technique, it has been calls it “a panacea” in a book.³ In fact, there are several cases where conventional analysis may either fail or apply -even not possible- with very difficult. For a wider approach to this method the reader may refer to the just-mentioned book³ and/or the work by Escobar, Jongkittnarukorn and Hernandez⁴ which contains a very wide state-of-the art of this technique along with its applications. Applications of TDS are not only extended to shale reservoirs and but also to pressure transient analysis and transient rate analysis, which the results are very much accurate and its use is quite easy.

TDS technique has many advantages. It has been applied to a great number of reservoir and well configuration cases providing equations and procedures for reservoir description. It can be successfully applied to cases in which pressure tests are too short or incomplete. There are cases where flow regimes can be artificially and accurately created. For instance, if permeability is previously known, the radial flow regime can be artificially found and drawn from a test on a hydraulically- fractured well when only linear or bilinear flow are observed. It can also be accurately applied to non-Newtonian fluid, fractal reservoirs, reservoirs with threshold pressure gradient, injection wells and a variety of conditions that takes too much space to be mentioned here. Then, Ref. 4 and 5 should be consulted. In spite of its power and versality, the use of TDS technique is not very popular which is demonstrated by the low impact of the publications on that topic.

Only two cases will be commented on TDS technique along this short note. One is regarding a work for well test interpretation of transient pressure tests in vertical wells under spherical power-law flow conditions.⁵ For pseudoplastic spherical/hemispherical flow, the slope of the pressure derivative is no longer -½, besides it changes with the value of flow behavior index, n, which indicates that the interpretation of pressure data for the dealt systems using traditional methods should not be accurate and may be difficult to accurately be applied.

Ci-qun presented the Laplace solutions for the case of infinite reservoir and constant-rate production. For n < 0.5 this is:

$\overline{P_D}\left(\ell \right)=K_{\frac{1-2n}{4-2n}}\left(\frac{\sqrt{\ell }}{2-n}\right)/K_{\frac{3}{4-2n}}\left(\frac{\sqrt{\ell }}{2-n}\right){\ell }^{\frac{3}{2}}$   (1)

When n = 0.5 Equation 6 reduces to the radial flow case. It means that the pressure behavior of Non-Newtonian fluid in a spherical flow for n = 0.5 is the same as that of Newtonian fluid in radial flow.

$\overline{P_D}\left(\ell \right)=K_0\left(\frac{2\sqrt{\ell }}{3}\right)/K_1\left(\frac{2\sqrt{\ell }}{3}\right){\ell }^{\frac{3}{2}}$   (2)

For 0.5 < n ≤ 1:

$\overline{P_D}\left(\ell \right)=\frac{K_{n-0.5}\left(\sqrt{\ell }\right)}{{\ell }^{3/2}{K}_{n+0.5}\left(\sqrt{\ell }\right)}$   (3)

When n = 1, Equation 6 reduces to the spherical flow case of Newtonian fluids.

The mathematical model provided by Equations (1) through (3) was used to build Figure 1. Figure 1 contains a dimensionless pressure and pressure derivative versus dimensionless time log-log plot of. As observed in such plot, the radial flow regime slope is dominated by the characteristics of the fluid. A pressure test of this nature can be interpreted by either conventional straight-line analysis and TDS Technique but providing better and verifiable results with the last one. To have access to the step-by-step interpretation techniques the reader must be addressed to Ref. 2 in which reference all the involved parameters are provided and detailed examples are given.

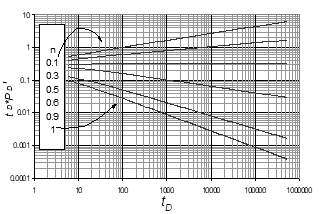
Figure 1 Behavior dimensionless pressure derivative for a non-Newtonian fluid in the spherical.³

On the other hand, the mathematical model used to generate Figure 2 was provided by Ichacra.⁶ This model provides a very particular situation and interpretation technique reported in.⁷ Spherical stabilization takes place when a partially completed well is perforated near a constant-pressure boundary, meaning that either there is a gas cap or a bottom aquifer overlying or underlying, respectively, the oil reservoir. This flow regime has a characteristic slope of -3/2 on the pressure derivative versus time log-log plot as seen in the just mentioned Figure.

This flow regime is only seen when the formation’s thickness is greater than 50 ft as depicted in Figure 2, in spite that a vertical/horizontal permeability ratio is small. Notice for the case of a smaller permeability ratio, last curve in the right, the spherical stabilization is seen for a reservoir thickness of 200 ft. Penetration ratios higher than 40 % prevent this flow regime of being observed. Also, for low permeability contrast, the radial flow can be slightly seen because the constant-pressure boundary effect is retarded. The complete steady-state period is fully developed once the transient wave has reached the no-flow pressure boundary; the thicker the reservoir the later the maximum point is seen. This maximum corresponds to both the presence of the no-flow boundary and the penetration ratio.

Figure 2 Effect of wellbore storage on the spherical stabilization flow regime.⁷

From the characteristic points found on the pressure derivative curve presented in Figure 3, the interpretation technique was developed⁷. A point on the spherical stabilization flow regime which exhibits a slope of -3/2 on the pressure derivative plot is read. An equation for determination of the vertical permeability was then provided in.² The maximum point observed just before the development of the steady-state period is unique. This allows for the estimation of the radial permeability. Once the permeability is obtained, the value of the pressure derivative is drawn -horizontal line- on the plot – artificially but logically created- which interception point with the -3/2-slope line leads to the estimation of a new value of vertical permeability. This assures the self-verifiability of the TDS technique.

Figure 3 Unified behavior of the dimensionless pressure derivative versus time log-log plot.⁷

For developing both conventional analysis -very limited for this case providing incomplete results- and TDS Technique, a unified pressure derivative curve for different values of reservoir thickness, thickness penetration ratio and vertical/horizontal permeability ratio. All the curves⁷ fall into one when the dimensionless time being multiplied by the permeability ratio and the pressure derivative also multiplied by the penetration ratio to the power -3/2. From this plot, Escobar, Ghisays-Ruiz, and Srivastav⁷ developed the analytic equations for reservoir characterization using both conventional analysis and TDS Technique. The first one presents some limitations in the estimation of reservoir parameters. Ref. 2 also presented examples to demonstrate the accuracy of the equations and the practicality of the TDS Technique.⁸

A very brief comment is given on the TDS technique with the purpose of call the reader’s attention and motivation so that the use of this powerful methodology can be spread out since it has been shown to be a practical, accurate and easy to use for oil or gas well test interpretation. TDS technique is the best accurate option when short test are into consideration and can be used to artificially create non-existing flow regimes. Also, TDS Technique has covered several scenarios but its use is not well spread, which is seen by the impact of the publications on this field. However, we have made an educated assumption that TDS Technique is widely used by most popular commercial software since they use straight lines and one of the most popular commercial software uses intersection points. TDS Technique is also the only alternative to interpret pressure tests in some complex systems such as non-Newtonian fluids, fractal reservoirs, short tests, among others which do not have mathematical models already included in most commercial well test interpretation software.

None.

There are no conflicts of interest.

None.

1. Tiab D. analysis of pressure and pressure derivative without type-curve matching: 1- skin and wellbore storage. Journal of Petroleum Science and Engineering. 1993;12:171–181.
2. Spivey JP, Lee J. Applied well test interpretation. SPE Textbook series. Society of Petroleum Engineers. 2013;13:385.
3. Escobar FH. Novel, Integrated and revolutionary well test interpretation and analysis. IntechOpen. 2019.
4. Escobar FH, Jongkittnarukorn K, Hernandez CM. The power of TDS technique for well test interpretation: a short review. Journal of Petroleum Exploration and Production Technology. 2018;9:731–752.
5. Escobar FH, Martinez JA, Bonilla LF. Transient pressure analysis for vertical wells with spherical power-law flow. CT&F. 2012;5(1):19–35.
6. Ichara MJ. Pressure behavior in vertically fractured wells subject to limited entry and bottom water drive. Society of Petroleum Engineers. 1981.
7. Escobar FH, Ghisays Ruiz A, Srivastav P. Characterization of the spherical stabilization flow regime by transient pressure analysis. Journal of Engineering and Applied Sciences. 2015;10(14):5815–5822.
8. Ci qun L. Transient spherical flow of non-newtonian power-law fluids in porous media. Applied Mathematics and Mechanics. 1988;9(6):521–525.