International Journal of
eISSN: 2475-5559


Research Article Volume 1 Issue 4
1Department of Mechanical Engineering, The Petroleum Institute, United Arab Emirates
1Department of Mechanical Engineering, The Petroleum Institute, United Arab Emirates
Correspondence: Nabil Kharoua, Department of Mechanical Engineering, The Petroleum Institute, United Arab Emirates, Tel 97126075416, Fax 97126075220
Received: October 27, 2016 | Published: December 30, 2016
Citation: Kharoua N, Khezzar L. Effects of Geometry, Temperature, and Inlet Conditions on the Flow Split in Spheroids Manifold. Int J Petrochem Sci Eng 2016;1(4):106-113. DOI: 10.15406/ipcse.2016.01.00022
A Computational Fluid Dynamics CFD study was conducted on the mal-distribution problem occurring within the pipe network upstream of pairs of spheroids gravity separators used in the oil industry. The series of simulations were conducted using the Euler-Euler multiphase and the k-ε turbulence models. The cases studied reflect different scenarios of oil production capacity in addition to the effect of seasonal variations of temperature increasing from 10°C during winter to 24°C during summer. The inlet conditions were varied so that they permitted to elucidate the effects of the flow rates and temperatures at the inlet of the piping network on the multiphase flow behavior and hence the mal-distribution within the pairs of spheroids considered. Two manifold configurations were taken from real installations in the oil industry. They contain T-junctions with different orientations. Averages of 2.2 million computational cells were generated for each case studied.
The main source of mal-distribution was found to be the sequence and cascading of the existing T-junctions inside the pipe network that are known to act like phase separators. A mal-distribution between the risers of each spheroid was, also, noticed. The relatively large number of T-junctions used, as well as the structure of the downstream piping network employed, led to complex multiphase flow behavior. The mal-distribution, generated by different flow scenarios, were less than 12 for the manifold referred to as Configuration 1. Configuration 2 caused more noticeable mal-distribution reaching up to 40%. The symmetry of the piping networks and the arrangement of the T-junctions were found to be a key parameter among the causes of the mal-distribution.
Keywords: spheroids, T-junction, flow mal-distribution, multiphase flow, CFD
CD Drag coefficient
d Droplet diameter
D Pipe diameter
f Drag function
gi Acceleration of gravity in the i direction
Gk Turbulent kinetic energy production term
Kpq Interphase exchange coefficient
k Turbulent kinetic energy
p Pressure
Re Reynolds number
S1, S2, S4, S5 Spheroids
N1, N2, N3, N4 Risers
Ui, Uj xi, xj mean velocity component
xi, xj Cartesian coordinates
α Volume fraction
ε Turbulent kinetic energy Dissipation rate
μ Dynamic laminar viscosity
μt Dynamic turbulent viscosity
ρ Density
σk, σε Prandtl coefficient associated with k and ε respectively
ˉˉτq
Stress-strain tensor
τp Particle relaxation time
Phase separation is a key operation in the oil industry. Crude oil usually contains oil, water, gas, and some other minor components such as solids and salt. Oil and gas should be as pure as possible to match the international standards for hydrocarbons commercialization.1 The separation process is done by means of large settling devices among which batteries of spheroids are placed downstream of low pressure LP separators. The piping network connecting the spheroids to the LP separators causes a mal-distribution of the crude within each pair of spheroids leading to an overloading of one of the spheroids and an under-loading of the other. The finite volume technique within the framework of the Reynolds averaged Navier-Stokes (RANS) equations and the k-e turbulence model with an Eulerian-Eulerian multiphase model was used to capture the complex behavior of the gas, oil, and water constituting the crude oil. A geometry reflecting the piping network causing the mal-distribution problem was built and decomposed in finite volumes to solve the set of equations governing the multiphase flow.
The potential sources of unequal split of the mixture are Tee-junctions inside the piping networks. Multiphase flow distribution inside T-junctions is challenging to predict and has been the subject of several previous experimental studies. It is noteworthy to mention that almost all of the existing literature is related to the nuclear and power industry. Pao et al.2 stated that the work of Orange3 was the first study on irregular distribution of phases at the pipe junctions. There is consensus within the research community that the main parameters affecting the flow split inside a T-junction are: the geometry (dimensions and orientation of the side arm), inertia differences of the phases, gravity effects, and the flow pattern upstream of the T-junction.4–6
It should be mentioned that the majority of the previous studies considered laboratory test cases of T-junctions without complex pipe networks downstream as encountered in industry and which might represent an additional important source of resistance to the flow changing remarkably the flow split trend inside the junction. Azzopardi & Whalley4 after studying the effect of the different parameters affecting the flow split inside T-junctions, recommended that two-phase flows should not be passed through T-junctions and manifolds unless a very severe mal-distribution of phases at outlet is tolerated. Azzopardi7 mentioned the importance of the rest of the system downstream of the T-junction on the multiphase flow split behavior. Hart et al.8 have explained that the liquid route preference is dictated by the balance between forces due to pressure drop (driving force) and due to axial momentum. The research study stated, hence, that for high flow rates, the liquid would prefer the straight trajectory inside the T while, for low flow rates, it would penetrate more easily in the T branch. Recently, research studies explored the possibility to use T-junctions in serial as efficient pre-separation tools.9 The idea was to use a horizontal pipe connected to a first vertically upward side arm followed by a vertically downward side arm. This has permitted to reach gas-rich product stream containing less than 10 % by volume liquid over a wide range of inlet conditions. This emphasizes the effects that T-junctions can have on upstream approaching multiphase flow with dramatic consequences on flow distributions.
In the present CFD study the Eulerian-Eulerian multiphase model in conjunction with the k-ε turbulence model were used to simulate the multiphase flow behavior inside the pipe network upstream of pairs of spheroids. Several geometrical combinations with different inlet conditions were investigated. The cases studied reflect different scenarios of oil production capacity in addition to the effect of seasonal variations of temperature increasing from 10°C during winter to 24°C during summer. The inlet conditions were varied so that they permitted to elucidate the effects of the flow rates and temperatures at the inlet of the piping network on the multiphase flow behavior and hence the mal-distribution within the pairs of spheroids considered. The CFD approach adopted is presented in the next section including the geometry of the installations, the mathematical models, and the boundary conditions. Subsequently, the results of the simulation cases are illustrated and discussed followed by the conclusions summarized in the last section.
This section describes the methodology of the present numerical simulation work. The geometrical configurations, the governing equations, the boundary conditions are presented in detail.
Manifold configuration
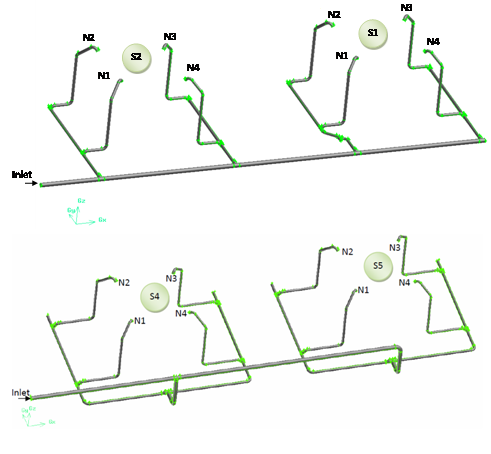
A sketch of a pair of spheroids is presented in Figure 1. The upstream manifold constitutes a complex configuration due to the use of fittings especially T-junctions.
The geometry of the pipe network was built in multi-blocks and meshed with a hybrid (98 % hexahedral and 2 % tetrahedral) grid. An average of 2.2 million cells was generated for each case studied. This corresponds to the upper limit of the computational resources available. The two different configurations considered in the present work are shown in Figure 2 and an example of the mesh used is illustrated in Figure 3.
Eulerian-Eulerian multiphase model
The discretize form of the continuity and momentum equations, for each phase q, are solved to obtain the individual flow fields and volume fractions of each phase. The multiphase continuity equation is
∂∂xi(αqρqUi,q)=0 …….. (1)
With the condition that ∑nk=1αk=1
The momentum equations for each phase are defined as
∂p∂xi+∂∂xj(ˉˉτq)+αqρqgi+n∑p=1→Rpq ………. (2)
Where the stress-strain for the qth phase is modeled as
ˉˉτq=αqμq(∂∂xjUi,q+∂∂xiUj,q)+αq23μqδij∂∂xkUk,q …… (3)
And the interaction force model between phases →Rpq is
n∑p=1→Rpq=n∑p=1Kpq(→Up−→Uq) …… (4)
Kpq Is the Interphase exchange coefficient and is equal to
Kpq=αqαpρpfτp …….(5)
The particulate relaxation time is defined as
τp=ρqd2p18μq …… (6)
Where f is the drag function
f=CDRe24 ……. (7)
And CD is the drag coefficient which according to10 is
24(1+0.15Re0.687)Re Re≤10000.44 Re>1000 ………. (8)
The relative Reynolds number for primary phase q and secondary phase p is
Re=ρq|→Up−→Uq|dpμq ………….. (9)
Additional forces, such as lift and virtual mass, were neglected. The lift force is due mainly to velocity gradients of the primary phase and is exerted on large secondary-phase liquid droplets. It is not appropriate for closely packed droplets as it is the case for the multiphase flow studied and is insignificant compared to the drag force. Regarding the virtual mass force, it should be added when the density of the secondary phase (oil and water in our case) is much smaller than that of the primary phase (gas in our case).
The relative Re for secondary phase’s p and r is
Re=ρrp|→Ur−→Up|drpμrq …….. (10)
Since the flow is multiphase, the turbulence model should take in consideration the phases contributions and interactions. The turbulence model chosen considers the mixture as a single fluid which characteristic turbulent variables are injected into the individual phase momentum equations. The differential equations for the prediction of the turbulent kinetic energy k and its dissipation rate ε have the form
∂∂xj(ρmUm,jk)=∂∂xj(μt,mσk∂∂xjk)+Gk,m+ρmε …….. (11)
∂∂xj(ρmUm,jε)=∂∂xj(μt,mσε∂∂xjε)+εk(C1εGk,m−C2ερmε) ……… (12)
Where the mixture density is defined as
ρm=N∑i=1αiρi ………… (13)
The mixture velocity as
Um=∑Ni=1αiρiUi∑Ni=1αiρi ……… (14)
And the mixture viscosity as
μt,m=ρmCμk2ε ……………. (15)
The production term of k is
Gk,m=μt,m(∂∂xjUi,q+∂∂xiUj,q)∂∂xjUi,q …………….. (16)
C1ε=1.44, C2ε=1.92, Cμ=0.09, σk=1, σε=1.3
The relatively fine mesh and the set of individual phase equations necessitated to run the simulation in parallel mode using 8 processors for each case. The study employed the commercial code Fluent 12.1.
The Phase-Coupled SIMPLE algorithm was used for pressure-velocity coupling.10 The convection terms of the momentum, k, and ε equations were discretize using the second order upwind scheme while the QUICK scheme is employed for the volume fraction equation.
Boundary conditions
Individual mass flow rates of the phases constituting the crude mixtures were imposed at the inlets with Reynolds numbers, based on the inlet velocity and the pipe diameter D=0.743m, in the range 106-3.7x106 while a pressure condition was prescribed at the outlets. A no-slip condition with a standard wall function11 was employed at the wall boundaries. Boundary conditions and fluid properties of the reference winter, summer, and modified production scenario cases are detailed in Tables 1-6 for both configurations.
Inlet Flow Rate (Kg/S) |
Inlet Flow Rate (M3/S) |
Volume Fraction (%) |
Outlet Pressure (Pa) |
Density (Kg/M3) |
Viscosity (Kg/Ms) |
Fluid Temp(˚C) |
|
Oil |
391.20 |
0.47 |
10.11 |
15175 |
831.4 |
3.51E-04 |
12.38 |
Water |
10.66 |
0.01 |
0.23 |
1017 |
1.22E-04 |
||
Gas |
8.73 |
4.17 |
89.67 |
2.092 |
8.37E-06 |
Table 1 Boundary conditions-configuration 1-winter case.
|
Inlet Flow Rate (Kg/S) |
Inlet Flow |
Volume Fraction (%) |
Outlet Pressure (Pa) |
Density (Kg/M3) |
Viscosity (Kg/Ms) |
Fluid Temp(˚C) |
Oil |
386 |
0.468 |
6.67 |
15175 |
825.12 |
2.87E-04 |
24.82 |
Water |
10.55 |
0.010 |
0.15 |
1007.5 |
8.94 E-05 |
||
Gas |
14.06 |
6.533 |
93.18 |
2.15 |
8.42E-06 |
Table 2 Boundary conditions-configuration 1-summer case.
Inlet Flow Rate (Kg/S) |
Inlet Flow Rate (M3/S) |
Volume Fraction (%) |
Outlet |
Density (Kg/M3) |
Viscosity (Kg/Ms) |
Fluid Temp. (˚C) |
|
Oil |
449.58 |
0.54 |
9.2 |
15175 |
825.12 |
2.87E-04 |
24.82 |
Gas |
11.46 |
5.33 |
90.8 |
2.15 |
8.42E-06 |
Table 3 Boundary conditions-configuration 1-modified production scenario case.
|
Inlet Flow |
Inlet Flow Rate (M3/S) |
Volume Fraction (%) |
Outlet Pressure (Pa) |
Density (Kg/ M3) |
Viscosity (Kg/Ms) |
Fluid Temp. (˚C) |
Oil |
579.03 |
0.71 |
11.31 |
13775 |
816.98 |
3.72E-03 |
10.67 |
Water |
54.67 |
0.05 |
0.86 |
1017.99 |
1.27 E-03 |
||
Gas |
11.18 |
5.50 |
87.83 |
2.03 |
8.73E-06 |
Table 4: Boundary conditions-configuration 2-winter case.
|
Inlet Flow Rate (Kg/S) |
Inlet Flow Rate (M3/S) |
Volume Fraction (%) |
Outlet Pressure (Pa) |
Density (Kg/M3) |
Viscosity (Kg/Ms) |
Fluid |
Oil |
571.42 |
0.71 |
7.22 |
13775 |
809.24 |
2.93E-04 |
24.74 |
Water |
54.49 |
0.05 |
0.55 |
1007.52 |
8.96E-05 |
||
Gas |
19 |
9.02 |
92.22 |
2.1 |
8.73E-06 |
Table 5 Boundary conditions-configuration 2-summer case.
|
Inlet Flow Rate (Kg/S) |
Inlet Flow Rate (M3/S) |
Volume Fraction (%) |
Outlet Pressure (Pa) |
Density (Kg/M3) |
Viscosity (Kg/Ms) |
Fluid |
Oil |
244.96 |
0.30 |
7.32 |
13775 |
809.24 |
2.93E-04 |
24.74 |
Gas |
8.03 |
3.82 |
92.72 |
2.1 |
8.73E-06 |
Table 6 Boundary conditions-configuration 2-modified production scenario case.
The modified production scenario case data correspond to the temperature of the summer season. The same fluid composition of the winter case was used at higher temperatures of about 24.82˚C to test the temperature effects expected during the summer season with the same production capacity, these cases with higher temperatures will be referred to in the present work as summer case.
Tables 7 & 8 summarize the differences between the input data for the cases studied taking as reference the winter case.
Flow Rate |
Winter Case (Kg/S) |
Modified Production Scenario Case (Kg/S) |
Change In % |
Summer Case (Kg/S) |
Change In % |
Oil |
391.20 |
449.6 |
14.92 |
385.99 |
-1.33 |
Water |
10.66 |
28.7 |
169.20 |
10.55 |
-1.05 |
Gas |
8.73 |
11.5 |
31.21 |
14.06 |
60.94 |
Table 7 Comparison of the inlet conditions of configuration 1.
Flow Rate |
Winter Case (Kg/S) |
Modified Production Scenario Case (Kg/S) |
Change In % |
Summer Case (Kg/S) |
Change In % |
Oil |
579.03 |
244.96 |
-57.69 |
571.42 |
-1.31 |
Water |
54.67 |
15.64 |
-71.40 |
54.49 |
-0.32 |
Gas |
11.18 |
8.03 |
-28.12 |
18.97 |
69.67 |
Table 8 Comparison of the inlet conditions of configuration 2.
Results and discussion must illustrate and interpret the results of the study.
The mal-distribution is illustrated through a mass balance count between the inlet and the outlets of each case. Furthermore, a mass balance for the risers of each spheroid is also considered. An investigation of the possible reasons of the multiphase flow split behavior inside the T-junctions is conducted based on details of the internal flow structure. Simulations using only two phases Tables 3, Table 6 have shown that the small amount of water can be omitted without any noticeable effect on the final solution of the simulation. The winter production conditions are taken as a reference case.
Effects of geometry (winter case)
Configuration 1 is characterized by reduced horizontal side arms of the T-junctions connected to the header. The remaining T-junctions feeding the risers have an upward inclination of 45˚C.
Smoglie & Reimann12 have described the phenomena occurring during the passage of a stratified flow through a horizontal T-junction and have determined what they called the beginning of gas and liquid entrainment corresponding to certain flow conditions. In addition, Azzopardi & Smith13 concluded that reduced T-junctions can cause much more pronounced phase redistribution as it is the case for the present work.
Relying on the existing literature, although limited for laboratory scale, explanations of the multiphase flow behavior inside both configurations are presented in this section considering the winter case boundary conditions.
Configuration 1 Figure 4 presents almost 10% (gas) mal-distribution between S1 and S2. However a considerable mal-distribution between the risers is observed. A discrepancy of about 5 % in mass balance was observed for the liquid phases.
Configuration 2 Figure 5 generates a mal-distribution reaching about 20% between S4 and S5. Contrary to Configuration 1, the distribution within the risers of each spheroid is quasi-homogenous.
Figure 6 shows the oil volume fraction distribution inside the whole domain in addition to the four T-junctions connected to the header for configuration 1. The oil-liquid stratification is clearly seen at the last junction however it is not evident at the first one. The risers, containing less fluid (N2 and N3) for both spheroids, are characterized by an accumulation of the liquid phase increasing, thus, the resistance effect and pushing the fluids towards the other risers. At the first junction, where no evident stratification is seen, the oil seems to be still dispersed inside the side arm while at the last junction, stratification is noticed inside the side arm. The velocity field, illustrated in Figure 7, shows clearly that any accumulation of liquid seen in Figure 6 is related to a decay of the velocity leading to a more noticeable gravitational effect compared to the inertial momentum.
Contours of the oil volume fraction distribution, inside Configuration 2 Figure 8, show a blockage effect due to the recirculation zone generated in the branch Figure 9.
This configuration contains a vertically downward side arm connected to the header constituting the T-junction where the main flow split occurs contrary to Configuration 1 where four T-junctions with horizontal branches are employed. This makes the first T-junction the most important location of flow split and, thus, the main source of mal-distribution.
Effects of temperature
It can be seen from Figure 10 (Configuration 1) that no significant effect of the temperature on the mal-distribution problem is observed. Nonetheless, the behavior of the fluids inside the risers has changed.
The trend has completely inverted in the case of S1. In this case only the gas mass flow rate has changed. Again the riser N2 of spheroid S2 inside which almost all fluids are quasi-stagnant correspond to an accumulation of liquid Figure 11 and a decay of the velocity field Figure 12.
For configuration 2 Figure 13 the mal-distribution trend is similar to that of the winter case. Similarly to Configuration 1, the increase of the gas mass flow rate has delayed, somehow, the stratification of the oil phase inside inlet pipe Figure 14. It was seen (not showed herein) that an appreciable pressure increase occurs in the header just beyond the first T-junction while it decreases remarkably inside the branch. The pressure difference between the run and the outlets of S5 is much higher than that between the branch and the outlets of S4 which led to higher velocities inside S5’s manifold compared to those inside S4’s manifold Figure 15.
Effects of inlet conditions
The inlet conditions of the modified production scenario case are higher for Configuration 1, in terms of mass flow rates, than those of the winter case Table 7. It was observed that the increase of the gas flow rate only, from winter to summer due to the temperature increase, doesn’t affect the trend of the mal-distribution. However, the liquid inlet flow rate has also increased for this case and this is most probably the reason why the mal-distribution trend has inverted making the amount of liquid and gas taken by S1 higher than that taken by S2 due to the increased axial momentum generated by higher velocities inside the header Figure 16. An accumulation of quasi-stagnant oil inside the risers N2 and N3 of S2 was observed corresponding to deceleration of the oil phase and a pressure increase inside the headers.
For Configuration 2 Figure 17, the same trend, compared with the winter case, was obtained but with a more noticeable mal-distribution reaching 40 %. The amount of fluids leaving the piping network via the risers N1, N2, and N3, of S4, was limited. In fact, there was an accumulation of oil in those risers. This production scenario is 32 % lower than the reference case. Hence, the lower corresponding flow rates at the inlet of the piping network for this case have caused a different multiphase flow behavior inside the risers Table 8.
Tables 9 summarize the main differences, in terms of percentage of liquid accumulated inside each spheroid.
Case |
First Spheroid S2 (%) |
Second Spheroid S1 (%) |
Description |
Mal-Distribution (%) |
Winter |
53 |
47 |
- Reference case |
6 |
Summer |
49 |
51 |
- Higher gas inflow |
2 |
Modified production scenario |
46 |
54 |
- Higher inflow |
8 |
Table 9 Configuration 1: summary of the results.
The authors of the present work are grateful to the Petroleum Institute of Abu Dhabi for providing High Performance Computing facilities.
The author declares no conflict of interest.

©2016 Kharoua, et al. This is an open access article distributed under the terms of the, which permits unrestricted use, distribution, and build upon your work non-commercially.