Descriptions of observations of the movement of ball lightning down from the cloud and up to the cloud are given. Based on this, it was concluded that it has a positive charge. The case was considered when ball lightning resisted the action of the wind due to attraction to the cloud. Examples of ball lightning “hovering” at a certain height above the ground are described. To explain this, a hypothesis was proposed about formation in the space between ball lightning and the conductor a cloud of clusters having a charge of the same sign as the charge of ball lightning. This idea was tested experimentally. It is shown that the process of formation of a “cloud” of charges near ball lightning can be the reason for the existence of groups of ball lightning associated with each other. Examples of descriptions of ball lightning movement near a flying aircraft and inside its cabin are given. An explanation of these events is given.

Keywords: ball lightning, motion, charge, levitation over conductors, groups of ball lightning

The generally accepted opinion about the nature of the movement of ball lightning, which can be found in descriptions of its observations, is the statement that its movement does not obey any laws. The observers say that it can move both up and down, chaotically changing the direction of movement.¹⁻⁵ However, a same strangeness in its behavior was noticed. It is said that sometimes ball lightning can move against the wind. To explain this, a hypothesis was proposed according to which ball lightning is an object that has the ability to move in the air without friction.⁶ There are two documentary evidences that allow us to get an idea of the nature of ball lightning movement. The first of them is a photograph of the trail of ball lightning, taken in 1960 in Sochi (Russia).⁷ (Figure 1)

Figure 1 Photograph of falling ball lightning, taken in Sochi in 1960.⁷

​The second is a video about ball lightning observation in the Moscow region Mitino in 2015.^8,9 (Figure 2) The “Sochi’s” photograph shows the trail of vertically falling ball lightning, which finally discharged at the top of a pole with a street lamp. The thickness of the trace is the same along the entire trajectory of the ball. This means that the ball lightning moved at a constant speed. While descending, it made at least three stops, which manifested itself in the form of loops in its wake.¹⁰ If we assume that ball lightning has a certain mass, then its movement could be explained by falling into the Earth's gravitational field. However, the reason for the appearance of loops on its trail remains unexplained. They could appear if ball lightning had an electric charge, which was affected by the oscillating electric field of a linear lightning discharge. Let us assume that the electric field of the atmosphere, acting on the charge of ball lightning, forced it to move downward. The place where the “Sochi’s” ball lightning descended was 1-2 km away from the place where the linear lightning discharge is visible in the photograph. Typically, a thundercloud is an electric dipole several kilometers long. The upper part of the cloud is positively charged.¹¹ Far from the cloud, electric field lines run to the ground from the top of the cloud. Thus, it can be assumed that the electric field vector at the location of the ball lightning movement was directed downward. Since the ball lightning moved in the direction of this vector, we can conclude that it had a positive charge. An analysis of the behavior of ball lightning in Mitino⁹ made it possible to understand the reason for its ability to resist wind pressure. The ball lightning was filmed simultaneously by the three operators, this made it possible to determine its location using triangulation (Figure 2).

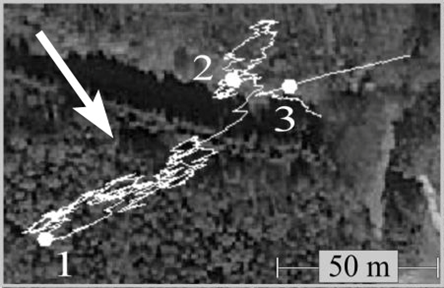
Figure 2 Horizontal projection of the trajectory of ball lightning, captured in the “trap”.⁹

In this figure the wind direction is shown by the arrow. The presence of a trail gap between areas 1 and 2 is explained by the fact that during this period of time the shooting was carried out only by one operator. Point 3 is the place where ball lightning began to rise to the cloud.

When a thundercloud approached Mitino, accompanied by a strong wind, ball lightning moved for about 80 seconds at a height 40-50 m in a limited area of ​​space 12 m wide and 100 m long. After that, it rose to the approaching cloud and began to move horizontally at the speed of the wind. At this time, the force acting on the ball lightning charge was directed upward, against gravity. Since the charge of the lower part of the thundercloud is negative, it follows that the charge of the ball lightning was positive. So, the assumption that ball lightning has a charge allows us to find an explanation of the reasons that determine its movement away from the surface of the earth. However, it follows from this that ball lightning that finds itself close to the earth (and, in general, near any conductor) must inevitably be attracted to it. This occurs due to the fact that in the electric field created by the positive charge of ball lightning, negative charges will accumulate in the conductor near it, and positive charges will be pushed out to the periphery. As a result, a force will arise that attracts ball lightning to the conductor. This force can lead to ball lightning falling to the ground and even to the formation of deep holes in the soil.¹² But more often than not, the behavior of ball lightning near a conductive surface turns out to be completely different. When falling, it stops, hangs at some distance from the ground and begins to move horizontally, repeating the details of the relief (rising above the hills and falling in the hollows). A case of ball lightning moving over a meadow overgrown with tall grass is described.¹³ The ball lightning flew horizontally at a distance 15-20 cm from the grass. The grass bent to the ground in front of the flying ball lightning. After this, the grass rose and stretched in the direction behind the passed ball lightning. It seemed that a “pit” of pressed grass was moving across the meadow. This behavior of ball lightning can be explained by the fact that when charged ball lightning approaches a conductor, a certain force begins to act between them, repelling it from the conductor. In articles^14,15 it was suggested that the cause of this force may be high-frequency radio emission generated by ball lightning. Due to this radiation, eddy electric currents are generated in nearby conductors. The interaction of the alternating magnetic fields of these currents with the alternating magnetic field of the generator located inside the ball lightning should lead to the appearance of a force that repels the ball lightning from the conductor. To test this idea, we measured the magnitude of the force repelling a coil of three turns of a copper tube with a diameter of 3 mm, powered by an alternating current with a frequency 440 kHz, from a copper plate.¹⁶ Experiments have shown that with increasing distance l from the lower edge of the coil to the plate, the repulsion force changes in inverse proportion to the value ${\left(l+a\right)}^7$ , where $a=4.6$ cm is the internal diameter of the coil. With a generator power of 4.6 kW, the repulsive force of the coil could be represented by the formula $F=3.675·{10}^7/{\left(l+a\right)}^{7}mN.$

At $l=1cm,F=213mN$ , and when the coil was removed to a distance equal to its diameter (5.2 cm), the repulsion force decreased by 50 times - to 4.2 mN. This means that a cause of ball lightning levitation at heights equal to several of its diameters cannot be the force caused by the interaction of eddy currents. This forces us to look for other ways to explain this phenomenon.

The reason of ball lightning levitation

Ball lightning constantly loses its charge. The charge carriers that “leaked” through the shell fly away from the ball lightning along the lines of the electric field it creates. Moving in the electric field of ball lightning, this charge transmits to it a mechanical impulse directed towards the center of the ball. If ball lightning is located at a large distance from conducting bodies, the electric field near its surface is uniform (the density of field lines emerging from its surface is the same everywhere). Therefore, the vector sum of impulses transferred to various parts of its surface will be equal to zero. However, if there is a conductor near the charged sphere, then due to the induction of charges of the opposite sign in it, the uniform distribution of field lines on the surface of the sphere will be disrupted. It may happen that almost all field lines will emanate from the part of the surface of the sphere facing the conductor. In this case, the force arising from the repulsion of charges will act only on half of the surface of the sphere, facing the conductor. Let's consider what effect the ions located on the outer surface of the shell render on ball lightning. They have the same charge sign as the charge of ball lightning. Therefore, they are repelled from it in the direction toward the conductor. Water molecules “stick” to these ions, and charged clusters are formed. Clusters, moving with friction against the air, can form in the space between ball lightning and the conductor some kind of “cushion” of charges of the same sign as the charge of ball lightning. Let us assume that at a distance $l=1$  m from the center of ball lightning, a “cloud” of clusters with a total charge q has formed between it and the conductor. Let the charge of this ball lightning be $Q={10}^{-3}C$  and the ball is located at a distance $L=3$  m from the surface of the earth. Let us take the characteristic time of charge drainage (approximately equal to the lifetime of ball lightning) to be $\tau =100s$ . Hence the average value of the current flowing from the surface of ball lightning is equal to $I=Q/\tau ={10}^{-5}A$ . Charge Q is attracted to its “image” in the ground with a force $F_{mr}=Q^2/4\pi {\epsilon }_0{\left(2L\right)}^2$ and is repelled from the “cloud” of charges with force $F_{rep}=Qq/4\pi {\epsilon }_0{l}^2$ . Here ${\epsilon }_0={\mathrm{8.854.10}}^{-12}F·m$  is the dielectric constant. Forces of attraction and repulsion will become equal at $q=Q{l}^2/4L^2$ . Substituting $Q={10}^{-3}C,l=1m$  and $L=3m$ , we find $q=2.8·{10}^{-5}C$ . This charge can be accumulated in the “cloud” in time $t=q/I=2.8s.$

To estimate the magnitude of the force repelling ball lightning from the “cloud” of charges, we carried out experiments to measure the force acting on the plates of a flat electric capacitor in the presence of a corona discharge between them.¹⁷ A capacitor was used, consisting of two disks with a diameter 12 cm. The upper (moving) disk had 90 steel needles protruding above the surface of the plate to a height 5 mm. The side of the plate with the needles was facing inside of the capacitor. To eliminate the effect of ionic wind, 88 holes with a diameter 5 mm were drilled in the plate.

Figure 3 shows the dependence of the force of attraction of the plates on the square of the electrical voltage between them. It can be seen that when the voltage rises from zero to the value $U_{cd}$ , at which a corona discharge appears at the ends of the needles, the force of attraction of the plates changes in proportion to the square of the voltage, $F_{at}~U^2$ . When a corona discharge appeared, the attractive force $F_{cd}$  became less than $F_{at}$  and their difference $\Delta F=F_{at}–F_{cd}$  increased with increasing voltage as $\Delta F=z·U^2.AtU<30kV$  and the distance between the plates $l<7$ cm, the value $z{}_{exp}=1.64·10^{-12}N/V^2$ . Let us assume that at large values of the distance L of the ball lightning from the ground and at large values of the potential U on the surface of the ball lightning, the coefficient z does not become less than ${10}^{-12}N/V^2$ .

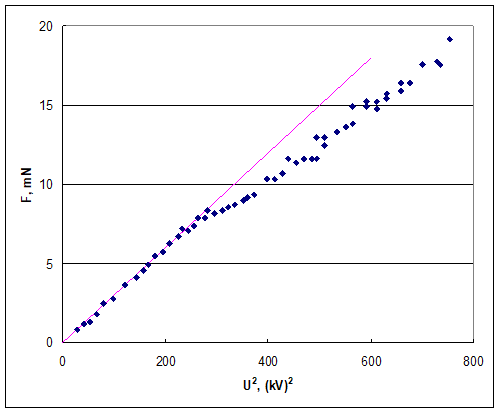
Figure 3 Dependence of F, the attractive force of a plate with 90 needles, in which 88 holes are drilled, on the square of the voltage U². The distance between the plates is 4 cm.

Let us return to the ball lightning discussed above with a radius $R={10}^{-1}$ m with a charge $Q={10}^{-3}C$ , flying above the ground at a height of $L=3$ m. The potential on the surface of this ball lightning is $U=Q/4\pi {\epsilon }_{0}R={10}^{8}V$ . From here we find the lifting force $\Delta F=z·U^2={10}^{4}N$ . The force of attraction of charge Q to the ground is $F_{mr}=Q^2/4\pi {\epsilon }_0{\left(2L\right)}^2={10}^{3}N$ . As we see, according to our rough estimate, the force of ball lightning repulsion from the ground can exceed the force of its “mirror” attraction, and also can compensate the force of its gravity. Ball lightning will levitate at a certain distance L from the ground if, as this distance decreases, the force of its repulsion from the conductor increases. The reason for this increase may be the redistribution of field lines emanating from the surface of ball lightning - from spherically symmetrical to concentrated in the space between the charge and the conductor. Therefore, we can assume that the factor z will depend on the distance L. To a first approximation, the repulsive force can be represented by the formula $F_{rep}=A/L^n$ , where $n<2$ . The total force of repulsion of ball lightning from the ground is $F_{tot}=A/L^n–B/L^2–M_{bl}·g$ . (Here M_bl is the mass of ball lightning, and g is the acceleration of free fall). Under equilibrium conditions, $F_{tot}=0$ . The above-described observation of the movement of ball lightning over a meadow confirms the validity of the described mechanism of its levitation. The charges flowing from the ball lightning “sit” on the tops of the blades of grass and are repelled from them. The grass bends down. After this, the charges flow from the grass into the air and only the force caused by the polarization of the stems in the electric field of ball lightning remains. The stems rise and trail behind it.

​Guiding of ball lightning

Another pattern can be traced in the nature of ball lightning movement. Once near an extended conductor, ball lightning sometimes moves along it. A review¹⁸ reported that in 16% of observations, ball lightning flew over wires or other metal objects. According to,¹⁹ ball lightning moved along wires in 20% of cases. Typically, “guiding” occurs without direct contact with the conductor; ball lightning moves, remaining at a noticeable distance from it, sometimes up to 3 meters.³ The ball lightning that I saw as a child flew over the center of a line of eight telephone wires at a distance of 30-40 cm from the plane in which the wires were located. A detailed description of the movement of ball lightning along wires can be found in the book.⁵ “When lightning struck a power line support, a bright ball with a diameter 25-30 cm was formed at its top. The ball came off the support and began to move along the protective wire. This wire began to glow white and red. It glowed in the area from the ball to the top of the support. Having traveled 1-1.5 meters, the ball stopped, and then jumped onto the lower wire and, without changing color or brightness, rolled some distance along this wire and exploded. At the same time, small balls flew from the ball in different directions, fell to the ground and disappeared. However, the main ball did not disappear: it became smaller and changed color. After the explosion, the ball jumped onto the wire running below. It rolled along the wire for some distance, stopped again, somehow jumped over the wire, several more spark balls separated from it, and it fell onto the lower wire. Rolling along the wire again, the ball fell to the ground and disappeared. Falling from wire to wire, the ball did not move strictly vertically, but in an arc. The ball traveled half the distance between the supports in 2-3 minutes, its speed was about 1 m/s.” Dmitriev²⁰ witnessed the formation of ball lightning on the Onega River. Ball lightning traveled a path of about 90 meters over a bunch of seven rafts located in a circular arc with a radius of about 60 m. The width of the raft was about two meters.

This style of ball lightning behavior can be explained by the presence of an electric charge. When ball lightning approaches a long wire, its “polarization” occurs: charges of the opposite sign of the ball lightning charge are collected in the near part of the wire, and charges of the same sign are pushed to the periphery of the wire.²¹ (Figure 4)

Figure 4 Ball lightning over a wire. Side view.

The charges located on the wire near the ball lightning attract it to the wire, and the charges displaced to the periphery partially weaken the force of attraction. However, complete compensation of the attractive force does not occur, and in the absence of other forces, the ball lightning must “land” on the conductor. This can be prevented by the process of charge draining from the surface of ball lightning. Moving in the direction of the conductor, this charge creates a “cushion” of charges similar to the charge of ball lightning. At a certain distance between ball lightning and the wire, the force of attraction may be equal to the force of its repulsion from this “cushion”. The flow of ball lightning charge onto a wire can lead to an increase in its potential, and a corona discharge can occur on its surface. Apparently, this can explain the glow of the wire in the observation described above.⁵ “Explosions” of ball lightning with the formation of sparks can be considered as partial electrical discharges with the ejection of elements of its core. The mechanical recoil pulse from this discharge can tear the ball lightning away from the wire and cause it to either jump to the underlying wire or fall to the ground. The fact that ball lightning always moved from top to bottom means that it has a certain mass. The movement of ball lightning along a wire can be explained by the action of an electric field, the vector of which is directed along the wire. The reasons for the appearance of this field can be both the electric fields of thunderclouds and the drop in the potential of electric lines from the place of generation to the place of energy consumption. The curvilinear movement during the fall of ball lightning can also be explained by the action of this electric field. Let us pay attention to another feature of the movement of ball lightning over a flat extended system of conductors (rafts in Dmitriev’s case or parallel wires in my observation). Ball lightning “prefers” to move along the axis of this “ribbon” of conductors. The reason for this can be understood by looking at Figure 5.

The ball lightning, located above the outermost conductor of the strip (Figure 5a), will be subject to an attractive force from other conductors, which will force it to take an average equilibrium position (Figure 5b).

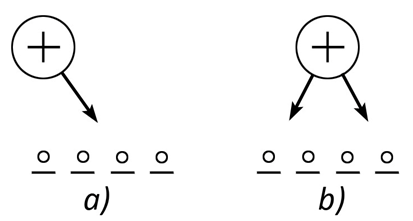
Figure 5 Ball lightning over a group of wires. View along the wires. a) Ball lightning deviated from the middle of the group. b) Ball lightning in an equilibrium position.

Groups of ball lightning

The literature describes many cases of observation of groups of ball lightning associated with each other. For example, the review²² tells how, before a thunderstorm on one hot day in August 1984, a woman suddenly saw a fireball with a diameter of about 3 cm, flying at a height of 2.5-3 m. “After some time, at approximately the same height as the first ball, two ball lightning the size of a tennis ball appeared. They flew at a distance 10 cm from each other in the same direction where the first ball flew... A few seconds later, three fire balls appeared at the same height. They flew at a distance 30 cm from each other, forming an equilateral triangle. Two of them were at a distance 15-20 cm from each other, and the third was 30 cm away from them.” Stakhanov³ cites a case when in the summer of 1955 two white balls with a diameter 10 and 15 cm flew into the kitchen of citizen Gerasimova through a chimney. “The balls flew one after another at a speed 2-3 m/s at a height 30-40 centimeters from the floor... Having flown up to the grounding wire, the balls stretched out, turning into ellipsoids. Having touched the wire, they silently disappeared into the hole in the floor into which the grounding went.” In the summer of 1976, “during a strong thunderstorm, ball lightnings flew out of a thick black cloud. They moved randomly one after another horizontally over a segment of about 100 meters long. The distance between them was from two meters to several centimeters. Sometimes they flew in groups of several. The size of the ball lightning was approximately 15 cm, they moved at a height of about 2 m at the speed of a running person”.⁵ At the end of August 1970, near the city Shuya, during rain with lightning and thunder, a witness observed five fireballs flying over the roofs of village houses. Two of them were larger than a soccer ball, and the other three were smaller. The order of their arrangement resembled a bunch of grapes.⁵ In July 1978 in Pskov, “during a severe thunderstorm, ball lightning, consisting of four balls, flew into the room on the first floor through the window: in front - a large one with a diameter of 10-15 cm, and behind it, in a chain at a distance of about 10 cm from each other, three more balls with a diameter 3 cm. The balls flew to the electric switches and went into them”.⁵ In the summer of 1980, “a witness, who was on the fourth floor, saw two “soap bubbles” with a diameter of about 15 cm at the level of the first floor. They were spinning in the air and very slowly rising up, moving away from the wall of the house. It was as if they were connected by a thread”.²³ “On March 8, 1999, at 10 p.m., on Khoroshevskoe Highway near Moscow, a yellow object of three large luminous balls flying from north to south was observed. The object made chaotic movements and swaying. One got the impression that the balls, located on the same line, converged and diverged from each other“.²³ Figure 6 shows a group of three objects that appeared in the Moscow sky on April 25, 2009.

Figure 6 Photograph of three luminous objects located at the vertices of a triangle. UFO in Moscow 04/25/2009 / UFO in Moscow: http://www.youtube/com/watch?v=yAvDC6BqbYQ

On August 25, 2002, in the Kaluga region, “an object in the form of three bright lights descended from the sky. It hovered over the trees and burned a triangular mark measuring 22 m × 25 m on their tops”.²³

Figure 7 shows video frames of the movement of two connected ball lightning over a wheat field.²⁴ The film shows that ball lightning moves randomly, but the distance between them remains unchanged, as if they are connected by an invisible thread. The ratio of the distance between the balls to their diameter is approximately 15.

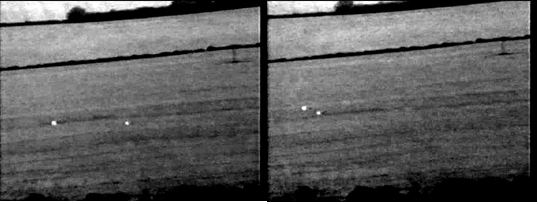
Figure 7 Video footage of the movement of a pair of ball lightning over a wheat field.²⁴ (Crop Circles, 2006). Crop Circles – Crossover from Another Dimension, 2006. Toftenes Multivision a. s. Oslo, Norway, 2006. www.toftenes.no.

Despite the fact that the property of ball lightning to form groups has been known to the scientific community since the publication of Brand’s book¹ (100 years ago), for some unknown reason, the creators of ball lightning models have not yet paid attention to explaining this phenomenon. An exception is the work of Japanese researcher Satoshi Kawano, who believes that ball lightning is the lower end of the undeveloped leader of linear lightning. If several such undeveloped leaders are nearby, this will be perceived by the observer as a group of ball lightning.²⁵ However, according to the examples above, groups of ball lightning were sometimes observed in the absence of lightning discharges and even indoors.

Let's see how the electrodynamic model can cope with explaining the existence of groups of ball lightning.^{14,15,21,26−30} According to this model, an indispensable property of ball lightning is the presence of an uncompensated electric charge. At first glance, the idea of constructing a stable system of charges of the same sign is not feasible, since these charges will repel according to Coulomb’s law. Such a system can be constructed only if it is possible to find forces that act opposite to the Coulomb forces. One of these forces may be the “gradient” force, which arises due to the polarization of the material of ball lightning in a non-uniform electric field created by the charge of another lightning. We tested this idea by measuring the pushing force of two plastic balls with a diameter 4 cm and a mass 2.72 g suspended on thin wires.³¹ Experiments have shown that in a real system of macroscopic charged bodies the repulsive force turned out to be approximately two times less than what follows from the result of calculating the repulsive force of point charges of the same value. The reduction in the repulsion force of plastic balls occurred due to the polarization of the ball material and the appearance of a “gradient” force $F_{gr}$ . However, this force is unable to completely compensate for the force of Coulomb repulsion of charges $F_C$ . Firstly, it is less than the Coulomb force and, secondly, it decreases with distance as $F_{gr}~R^{-3}$ , that is, faster than the Coulomb force $F_C~R^{-2}$ . Therefore, to explain the reason for the existence of stable configurations of charged bodies, we must turn to the search for another force that causes charges to approach each other. This force should decrease with distance R slower than the Coulomb force. The reason for the appearance of such a force can be the flow of a body’s charge into the air and the repulsion of the body from the resulting cloud of charges. Due to friction with the air, these charges do not immediately leave the space near the body. The sign of the charge of the resulting cloud coincides with the sign of the charge of the body. When two similarly charged bodies (for example, balls) are close together, their charges will flow in the direction of the line connecting the centers of the balls. This will lead to the formation of charge clouds on the outer sides of the system. The repulsion of charged balls from these clouds creates a force directed opposite to the Coulomb force, which tends to push the balls apart. At a certain distance between the balls, when the Coulomb repulsion force becomes quite weak, this new “cloud” force can stop the scattering of like charges and lead to the formation of a stable configuration of two, three (and so on) balls. Let us pay attention to the fact that we do not violate the conclusion of Earnshaw’s theorem (about the impossibility of creating a stable configuration from stationary charges), since we are dealing with charges immersed in a medium whose properties have been changed by the same charges.

In the first part of the article, we described the process of formation of a cloud of charges in the space between ball lightning and a conductor. The same cloud should appear in the area outside the group of ball lightning. Consider, for example, a system, consisting of two ball lightning, which possess equal charges Q (see Figure 7). In this case, on the outer sides of this group of ball lightning, two clouds of charges will appear on a straight line passing through their centers. The force $F_{cl}$ , which tends to bring ball lightning closer together, practically does not depend on the distance R between the ball lightning and is determined only by the amount of charge Q and the strength of the current flowing from the surface of the ball lightning. Figure 8 shows how the square of the sum of forces acting on the charges changes with the distance R between the centers of two ball lightnings with charges values $Q={10}^{-3}C$ . The force of Coulomb repulsion of charges is $F_c=Q^2/4\pi {\epsilon }_0{R}^2$ . The following forces act opposite to this force: the gradient force caused by the polarization of the ball lightning substance, $F_{gr}=–\left(D.2Q\right)/4\pi {\epsilon }_0{R}^3$  (the dipole moment is taken equal to $D={2.5}^{.}{10}^{-4}C·m$ ), and the force of repulsion of the ball lightning charge from cluster cloud charge $F_{cl}=const={1.5}^{.}{10}^{3}N$ . For the indicated charge values $\left(Q={10}^{-3}C\right),$ the equilibrium position is observed at a distance between the centers of ball lightning $R_{min}=2m$ . This is comparable to the value of the distance between ball lightning with a diameter about 20 cm shown in Figure 7.

Figure 8 Dependence of the square of the sum ${\left(F_c–F_{gr}–F_{cl}\right)}^2$ of forces acting on two ball lightning with a charge of each $Q={10}^{-3}C$ , located at a distance R from each other.

Movement of ball lightning indoors

The movement of ball lightning indoors obeys some general rule. “In July 1965, ball lightning with a diameter 8-10 cm flew through an open door into a construction trailer with walls measuring 4 m × 8 m. Having flown into the trailer, it turned left and began to move at a height one meter from the floor along the walls at a distance 20 - 30 cm from them. It moved, smoothly rounding the corners, and went outside through the same door”.³ “In July 1952, during a thunderstorm, an orange ball with a diameter 5 cm passed into the room through the glass of the balcony door, glowing like a 25 W lamp. Having entered the room, it stopped as if in thought, stood for a moment and slowly, at a speed of about 1 m/s, swam along the walls at a level of two meters from the floor. Sometimes it stopped, as if “treading water” and, after “thinking” a little, smoothly moved on again. At the same time, it either stretched out in length, like a drop of mercury, then again took a spherical shape. So it made one circle around the room and went to the second... Suddenly its “attention” was attracted by a shiny orchestral trumpet hanging on the wall. The smooth movement of the ball noticeably accelerated, and it rushed towards this pipe. The ball flew into a wide bell, and there was an explosion, accompanied by a deafening metallic ringing.⁵

According to the electrodynamic model,^{14,15,21,26−30} ball lightning has an electric charge that gradually drains from it. The presence of a charge leads to the appearance of a force that attracts ball lightning to conductors and dielectrics. This force arises due to the polarization of the material in the non-uniform electric field created by the ball lightning charge. When objects (walls, metal surfaces) are close together, the charge flows predominantly in the direction towards them. In this case, a “cushion” of charges of the same sign as the ball lightning charge appears between the ball lightning and the object. The closer the ball lightning is to an object, the more “concentrated” the flow of flowing charge becomes. Therefore, ball lightning will tend to occupy a middle position between the floor and ceiling of the room. For a trailer with a ceiling height of just over two meters, its distance from the floor was equal to one meter, and in a room with a ceiling height 4 meters - two meters. The distance from the wall (20-30 cm), apparently, is the equilibrium position between the polarization force of attraction of ball lightning to the wall and the force of its repulsion from the space charge between it and the wall. The force of attraction of ball lightning to a metal surface (to an orchestra trumpet) is much greater than the force of attraction to a brick wall. The horizontal movement of ball lightning occurs due to the movement of air, which circulates along the walls in rooms with windows and doors.³²

Movement of ball lightning near an airplane

 In the 20th century, with the development of aviation, reports of observations of ball lightning, flying near aircraft appeared. Thus, Jennison^3,33 spoke about the case of observing a ball with a diameter of 20 cm, which for some time moved at a speed 1 m/s along the trailing edge of the wing of a flying aircraft, being away from it at a distance 50 cm. The ball did not deflate, despite the high speed of movement relative to the air. The book²³ describes a case when an IL-14 aircraft, flying at an altitude of 3600 m, was accompanied by a bright red ball the size of a soccer ball. Another incident occurred on June 27, 1978, when a white matte ball with a diameter 3 meters “attached” to the trailing edge of the right wing of the AN-30 laboratory aircraft, flying at an altitude 5000 m at a speed 380 km/h. For four minutes, the ball slowly moved towards the end of the wing, increasing in size and seemingly losing density. After this, the diameter of the ball increased to 10 meters, and it disappeared.²³ Once, during the flight of a passenger plane, a red ball with a diameter of about 40 cm landed on its wing. The ball rolled along the wing towards the hull of the plane. Passengers wondered why the balloon was not deflated by the air flow. After 5 seconds the ball disappeared.⁵ Cases of ball lightning flying near an airplane were studied by Gaidukov.^6,34 He came to the conclusion that the surface of ball lightning has special properties that ensure laminar air flow around the ball. Because of this, the ball does not experience any resistance to movement in a gaseous environment.

According to the electrodynamic model of ball lightning,^{14,15,21,26−30} it behaves in a gas flow like an ordinary body. The friction force $F_f$  of a ball of radius R flying in the air with speed v can be determined by the formula $F_f=C_x.{(\rho v^2/2)}^{.}\pi R^2$ . Here $C_x$  is a dimensionless coefficient, let us take it equal to 0.3, ρ is the air density. For a ball with a radius 1.5 m, moving at a speed 105 m/s at an altitude of 5000 m (for the case of the AN-10 aircraft), the air resistance force is $F_f=8600N$ . (For air density, the value taken here is $\rho =0.736kg/m^3$ ). Let us assume that the ball is held near the wing due to the presence of an electric charge Q. The force of attraction of the charge to the metal wing is $F_Q=Q^2/4\pi {\epsilon }_0{\left(2l\right)}^2$ . Here ${\epsilon }_0={8.854}^{.}{10}^{-12}F·m$  is the dielectric constant, and l is the distance between the center of the ball and the wing. At $l=1.5m$ , the forces $F_f$  and $F_Q$  will become equal at a charge value $Q={2.93}^{.}{10}^{-3}C$ . Such a charge can be contained in ball lightning. The question remains open: what ensures the stability of the position of ball lightning at a distance $l=1.5$ m from the wing? The position created by the electrical force of attraction is unstable. A possible explanation for the stable position of ball lightning could be a cylindrical vortex that broke off from the edge of the wing. According to the laws of aerodynamics, when an aircraft flies, lift is created due to the formation of air movement around the wing (the so-called Zhukovsky’s force).³⁵ When this vortex is formed, a vortex with the opposite direction of air rotation breaks off from the trailing edge of the wing. The reduced air pressure inside this vortex can hold ball lightning in it for some time. The same vortices are formed behind a flying aircraft. This may explain cases of an aircraft being accompanied by ball lightning.^36,37 In conclusion, we note that our assumption that ball lightning behaves in an air flow like an ordinary body is applicable only for rough estimates. In fact, the conditions on the surface of ball lightning differ markedly from the conditions on the surface of an ordinary physical body. The surface of ball lightning emits electrical charges, which, moving in a strong electric field, ionize the air. Due to this, a layer of plasma is formed near the surface, which can significantly change the conditions of movement of air flows and reduce the force of its friction with the air.³⁴

Ball lightning inside an airplane

There are many reports of ball lightning penetrating into an airplane cabin.^4,38,39 This occurs when flying in the clouds at altitudes 1000-6000 m. Often this is preceded by a linear lightning strike on the plane, but sometimes the ball appears in the cabin of the plane in the absence of a thunderstorm. So in 1946, while flying in clouds at an altitude 1200 m, “a dazzling white ball hung, pulsating and swaying, inside the cockpit of a four-engine Pe-8 aircraft. It changed color to greenish, went down the hatch into the navigation radio room and exploded under the radio operator’s seat. The internal telephone connection on the plane was lost, the walkie-talkie was out of order, and the legs of the radio operator's seat melted. Before this event, ball lightning was seen on the right wing console. It slowly crawled along the front edge of the plane and disappeared under the nose of the plane. It is unclear how it got inside the plane because all portholes and hatches were tightly closed”.³⁸ “In 1963, during a thunderstorm, after a lightning strike, a luminous ball with a diameter $22\pm 2$ cm appeared inside the passenger plane, emerging from the cockpit. It moved at a constant speed $1.5\pm 0.5$ m/s at a height 75 cm above the floor in the center of the cabin towards the tail of the aircraft. Nobody of the passengers were injured”.⁴ “In January 1984, during the takeoff of an IL-18 aircraft from Adler, a luminous ball with a diameter 10 cm appeared on the fuselage in front of the cockpit. It disappeared with a deafening roar, but a few seconds later it re-emerged in the passenger’s lounge. It slowly floated over the heads of the frightened passengers. At the tail of the plane, it split into two luminous crescents, which then connected again, and silently left the plane. The radar and other instruments failed, and the plane was forced to return to the airport. In result of inspection of the aircraft two holes were found in the fuselage - one in the nose section and other in the tail of the plane”.⁴ “During the flight of the LI-2 aircraft in the clouds in the absence of thunderstorm activity, a white luminous ball with a diameter 30 cm suddenly appeared under the instrument panel in the cockpit. The pilot ordered the mechanic to remove the ball. The mechanic took a piece of tarpaulin and kicked the ball into the tail of the plane. This took one or two minutes. After this, two holes 3 cm in size appeared in the floor of the tail section”.^39,40 “During the flight of the LI-2 at low altitude, a yellow ball the size of a soccer ball was attracted to the nose of the plane. The plane shook slightly. The ball broke away from the hull and, stretching out, flew into the cabin through the half-open window. It walked through the open cockpit door into the plane, moving in the middle of the hull. Then the ball headed towards the door and, stretching out again, went through the crack. The plane landed safely. The traces of melting were found on its nose”.³⁹ “In the summer of 1967, the plane collided with ball lightning. Holes with a diameter of about 1 cm were found near the cockpit lights. The edges of the holes were smooth, as if they had been drilled rather than burned. They were different from the typical bullet holes”.³⁹ Let us pay attention to the fact that the existence of ball lightning inside an aircraft with a metal hull (in a “Faraday cage”) absolutely excludes its model in the form of plasma, powered by electromagnetic radiation from an external energy source. Attempts to build such a model, considering the transfer of electric field energy through the glass of the cockpit, end in explaining its penetration a short distance from the glass, but are powerless to explain its movement in the aircraft cabin.⁴¹

According to the electrodynamic model,^{14,15,21,26−30} the behavior of ball lightning inside aircraft is explained by the presence of two properties: an electric charge and the ability to generate high-frequency radio emission. In the cases described above, ball lightning flew near the plane for some time before entering in the cockpit. This is only possible when there is a force that attracts it to the hull of the aircraft. If ball lightning has a charge, then this force is the force of attraction of the charge to the conductor. The charge (and its constant loss) “forces” the ball lightning to move through the center of the aircraft cabin. Its movement from nose to tail can be explained by the potential difference between the nose and tail of the aircraft, as well as the air flow in the cabin. Ball lightning enters the aircraft through existing openings (air intakes, antenna inputs) or through openings made by it. Passing through them, it changes its shape (stretches into a thread). The movement of ball lightning is controlled by the electric field of the atmosphere (figuratively speaking, it moves along electric lines). At a close distance from the metal hull of the aircraft, the ball lightning’s shape changes - it extends towards the hull and emits a powerful pulse of radio emission. Its power is sufficient to instantly evaporate metal over an area of about one square centimeter. Since the metal sublimates (instantly going through the melting stage), the edges of the hole are smooth. Ball lightning that passed through the cabin and left the plane through a hole in the tail, as a rule, does not affect the operation of the aircraft's electronic equipment. When ball lightning explodes inside an aircraft, due to the action of a powerful pulse of radio emission, metal objects melt, organic matter ignites, and electronic devices fail.

All observations described in the article are explained by just one property of ball lightning - the fact that it is a unipolarly charged object. In fact, this is a development of the idea of de Tessan, who in 1859 suggested that ball lightning is an electrical capacitor.⁴² The model of ball lightning, developed by our team, is an ensemble of charges of the same sign, located inside a spherical shell of polarized water molecules. Coulomb repulsion and pressure caused by the movement of charge carriers is compensated by the force of attraction of water molecules to the center of the charge region. This formation represents one of the poles of a huge electrical capacitor, the second pole of which is the surface of the earth. The insulator between the electrodes of this capacitor is a water shell and air. Thus, this configuration is fully the embodiment of the de Tessan’s idea, only in a new form. This capacitor, assembled from ordinary materials, like any industrial capacitor, is imperfect. The shell has finite conductivity, the capacitor is constantly discharging, and its lifetime is limited. But it is precisely this imperfection that gives it (that is, ball lightning) unusual properties. Ball lightning changes the physical characteristics of the medium in which it is immersed, and this medium acquires new properties. As a result, a unipolarly charged object “hangs” above the conducting surface, and balls with a charge of the same sign form figures of regular geometric shape. It is possible that studying this ability of ball lightning will help to understand the reasons for the appearance of luminous objects called UFOs. With a little imagination, a chain of ball lightning located in a circle can easily be mistaken for the lights of spaceship’s windows. The force of attraction of a charged ball to the metal wing of an airplane can be so strong that it will move next to the airplane, overcoming the friction of the incoming air. Assessments of the processes discussed in the article are only the first approximation to understanding the causes of the observed phenomena. It is possible that a more thorough analysis will reveal their new features. In particular, it is possible that on the surface of ball lightning, surrounded by a layer of corona discharge and a flow of emitted charges, conditions may be created that reduce the friction force when moving in the air.

None.

The author declares there is no conflict of interest.

1. Brand W. Der Kugelblitz. Hamburg: Verlag von H. Grand; 1923.
2. Singer S. The nature of ball lightning. New York: Plenum; 1971.
3. Stakhanov IP. About the physical nature of ball lightning. Moscow: Nauchnyi Mir; 1996.
4. Stenhoff M. Ball lightning. An unsolved problem in atmospheric physics. London: Kluwer Acad; 1999.
5. Grigoriev AI. Ball lightning. Yaroslavl: Publishing of YarGU; 2006.
6. Gaidukov NI. Steady motion of ball lightning observed by M.T. Dmitriev at Onega River. J Techn Fiziki. 1994;64(2):38−48.
7. Stekolnikov IS. Thunderstorm at Black Sea. Ogonyok. 1960;(2):34.
8. Sokolov V. Ball lightning in Mitino, 2015. http://www.youtube.com/watch?v=eyRL0BimsHM
9. Nikitin AI, Velichko AM, Nikitina TF, et al. Analysis of the unique case of ball lightning observation in Mitino, the northwest district of Moscow. J Atm Solar-Terr Phys. 2018;179:97−104.
10. Nikitin AI, Velichko AM, Vnukov AV, et al. Estimation of ball lightning parameters on the base of analysis of its photo. Khimicheskaya Fizika. 2007;26(8):80−89.
11. Uman MA. Lightning. New York: Mc Graw-Hill Co; 1969.
12. Tsaryov I, Tsaryova I. A mystery of witch’s circles. Moscow: Ripol Klassik; 2005.
13. Nikolaev GV. Mysteries of the electro-magnetism. Tomsk: OOO ”Znamya Mira”; 2001.
14. Nikitin AI. An electrical capacitor as the element of the power core of ball lightning. Electrical Technology of Russia. 1998;(4):70−85.
15. Nikitin AI. Electrodynamical model of ball lightning. Khimicheskaya Fizika. 2006;25(3):38−62.
16. Nikitin AI, Leipunsky IO, Nikitina TF. Role of eddy currents in lifting force creating for an autonomous emitter of radio-waves. Prikladnaya Fizika. 2010;(2):15−22.
17. Nikitin AI, Nikitina TF, Velichko AM. Corona discharge and levitation of ball lightning. Elektrichestvo. 2010;(3):16−22.
18. Rayle WD. Ball lightning characteristics. NASA Tech. Note, NASA-TN-D-3188, National Aeronautics and Space Administration, Washington, DC; 1966.
19. McNally JR. Preliminary Report on Ball Lightning//Report No ORNI-3938. Oak Ridge National Laboratory, Oak Ridge, Tennessee; 1996.
20. Dmitriev MT. Nature of ball lightning. Priroda. 1967;(6):98−106.
21. Nikitin AI. My ball lightning. Moscow: Nauka; 2022.
22. Egely G. Analysis of Hungarian ball lightning observations. In: Progress in ball lightning research. Editors. Keul AG. Proc. VIZOTUM. Salzburg, Austria; 1993.
23. Chernobrov V. Chronics of the UFO’s visits. Moscow: Drofa; 2003.
24. Crop circles – Crossover from another dimension. Toftenes Multivision A.S. Oslo, Norway; 2006.
25. Kawano S. Basic study of multiple ball lightnings in line by leader tip models. In: Proc. 7th Intern. Conference “Atmosphere, Ionosphere, Safety” (AIS-2020). Kaliningrad, Russia; 2020. 205−208 p.
26. Nikitin AI. An electrical capacitor as the element of the power core of ball lightning. Elektrichestvo. 1998;(11):14−23.
27. Nikitin AI. The dynamic capacitor model of ball lightning. In: Proc. 6th Intern. Symp. on Ball Lightning (ISBL-99). Antwerp, Belgium; 1999. 91−95 p.
28. Nikitin AI. Stability and maximum energy content of autonomous ball lightning. Elektrichestvo. 2004;(3):29−36.
29. Nikitin AI. The principles of developing the ball lightning theory. J. Russian Laser Res. 2004;25(2):169−191.
30. Nikitin AI. Possible process of ball lightning training in nature. J Atm Solar-Terr Phys. 2019;190:54−61.
31. Nikitin AI, Velichko AM, Nikitina TF, et al. Conditions for the existence of connected structures from bodies with an electric charge of the same sign. Prikladnaya Fizika, Uspekhi. 2017;5(1):28−40.
32. Shepelev IA. Aerodynamics of air streams in the premise. Moscow: Stroyizdat; 1978.
33. Jennison RC. Can ball lightning exist in a vacuum? Nature. 1973;245:95.
34. Gaidukov NI. Hydrodynamic model of ball lightning interaction with the air flow of a flying aircraft. J Techn Fiziki. 1993;63(9):16−18.
35. Vinogradov BS. Applied das dynamics: tutorial. Moscow: EKOLIT; 2011.
36. Kuzovkin AS, Semenov AE. Is UFO an invisible reality? Krylia Rodiny. 1988;(8):29−30.
37. Vostrukhin V. Precisely at 4.10. Newspaper “Trud”;1985. 3 p.
38. Akkuratov V. Meeting with a fairy ball. Tekhnika Molodyizhi. 1982;1:47−49.
39. Bychkov VL, Nikitin AI. Ball lightning. A new step in understanding. In: The Atmosphere and Ionosphere. Elementary Processes, Monitoring and Ball Lightning. Bychkov VL, Golubkov GV, Nikitin AI, Editors. Cham: Springer; 2014. 201−367 p.
40. Imianitov I, Tikhiy D. Beyond a boundary of laws of a science. Moscow: Atomizdat; 1980.
41. Lowke JJ, Smith D, Nelson KE, et al. Birth of ball lightning. J Geophys Res. 2012;117:D19107.
42. Tessan de M. Sur la foudre en boule. Compte Rendu. 1859;49:189−191.