There are at least four major and long-standing problems in solar physics, which require crucial considerations. These problems have been pursued during the last fifty years or more. They are: (1) The high temperature of the solar corona (since 1942), (2) The cause of the solar wind (since 1958), (3) The formation of a pair of sunspots (since 1961), (4) The cause of solar flares (since 1958). The last two have well-accepted theories (thus, it may be considered to be understood), but there are actually many unsolved issues. Thus, it may be worthwhile to examine the four problems together. Although each problem is different and is extremely difficult, there seems to be one common reason, which has delayed the progress. It is almost complete lack of considering electric currents, in spite of the that they are all electromagnetic phenomena and curl B = J. The purpose of this paper is to suggest that the introduction of electric currents, instead of magnetic field lines, may open a new way to consider these long-standing problems.

Keywords:solar wind corona sunspot flare

There are at least four major problems in solar physics, which have been pursued during the last half century or even more, but their understanding has been unsatisfactory. The reasons for this situation are many, in spite of the fact that these are extremely difficult problems. They are:

1. The high temperature of the corona
2. The cause of the solar wind
3. The cause of solar flares
4. The cause of sunspots

However, by examining them together, there seems to be at least one common reason for the slow progress of understanding these problems. It is almost complete lack of considering electric currents, in spite of the fact that they are all electromagnetic phenomena. In this short paper, we review these past studies and introduce electric currents. It is suggested that electric currents is one of the ways to improve the understanding these problems.

The basic reason for considering the high temperature of the corona is the discovery of highly ionized Fe and others cf. Van de Hulst¹ On the basis of those observations, the temperature of the corona is estimated to be 2x10⁶K, corresponding to 170 ev. Highly ionized atoms such as Fe^XIv require the ionization potential of about 280 ev, corresponding to temperature of 3.3 x 10⁶K. All attempts to explain the high temperature of the corona in the past have been to inject heat energy from the photosphere (6000K, corresponding to 0.5 ev) by various processes. However, in the most recent review, Doorsselaere et al.² showed that MHD waves cannot solve the problem. Further, the fact that the corona has many loop structures is another problem; Figure 1. The high temperature of the corona may be somewhat similar to state that the high temperature of the ionosphere of 1000K is based on the presence of ionized oxygen atoms (the ionization potential of 4 ev, corresponding temperature 3.3 x 10⁴K). The ionospheric oxygen atoms are ionized by the impact of auroral energetic electrons in field-aligned currents (10 keV), which are accelerated by the double layer above the ionosphere, not by heating from below. In most studies in the past, the presence of highly ionized Fe and some others is the reason for the high temperature. Their past efforts consider the injection of heat energy from the photosphere. They have not considered the fact that an energetic electron beam in field-aligned currents along magnetic field lines can ionize and produce highly ionized Fe atoms, rather than by injecting heat energy from below.

Figure 1 The image of the total eclipse of February 16, 1980 (Courtesy of Gordon Newkirk, the High Altitude Solar Observatory).

The acceleration of current-carrying electrons is made by the double layer. This acceleration process of electrons in the auroral field-aligned currents is proven by many satellites (Karlsson³). The double layer accelerates auroral electrons from about 300 ev to 10 keV or more to ionize oxygen atoms. The equation rate q for the ionization of energetic electrons in the corona is given by q=F Erd/ 30 ev , where F=electron flux, E=electron energy, r=mass density and d = penetration distance. For the corona, let us take F =6.2 x 10⁸/cm² s¹ (corresponding to 1mA/cm² ), E (5 keV), r(=1.6 x 10^-22 g (=10²/cm³ x 1.6 x10^-24 g), d=5 x 10⁹cm), q=6.0 x 10^-2/cm³ per 1mA/cm² A. Thus, this coronal ionization rate of 6.0 x 10^-2/cm³ is enough by the current intensity 1mA/cm², because the recombination is 10³ /cm³s^-1, so that the life time of ions (hydrogen atoms) is about 6.0 x 10⁹ seconds. Therefore, a current-carrying electron beam of 5 keV can ionize Fe atoms to the FeX^Iv stage; an electron loses about 30 ev. In each collision with Fe atoms. It is hoped that the above consideration may be useful for considering the high temperature of the corona. The high temperature of the corona may be due to the ionization by energetic current-carrying electrons, rather than heating from the photosphere. Further, since the field-aligned currents flow along loops of magnetic field lines, the loop structure of the corona can be explained.

There seems to be so far no acceptable theory on the cause of the solar wind during last 50 years or so. All attempts are obviously based on the assumption of internal causes. Most researchers consider both the heating of the corona and the generation of the solar wind together. Recently, Viall et al.⁴ made the most extensive review of physics of the solar wind and presented nine outstanding questions, but without specific suggestions of the cause. Since the coronal heating may not be heating from below, it is difficult to pursue such an approach.

Basically, the problem is that it is very difficult to overcome the solar gravitation by internal forces, either hydrodynamic or MHD forces from the photosphere. Thus, at this stage, it may be worthwhile to consider outside causes of the solar wind, instead of internal causes. One of the most promising forces is J x B force in considering outside causes. The basic conditions required for the solar wind by the J x B force are:

1. The J x B force must be directed outward.
2. The velocity of the solar wind is fairly uniform as a function of latitude during the solar minimum period (cf. McComas et al.⁵.

In order to satisfy the conditions (1) and (2), one possibility is to consider a spherical surface (considering it as the outer boundary of the heliosphere), on which (i) electric currents flow from the top of the heliosphere toward the equatorial plane along the surface of the heliosphere, and (ii) there is an eastward-directed latitudinal (azimuthal) magnetic field. Alfven⁶ suggested a unipolar (or homopolar) induction current system around the sun. In the solar unipolar induction system, the electric current flows out (or in depending the polarity of the solar dipole) from the northern pole of the sun along the polar axis; its intensity is estimated to be 1.5 x 10⁹ A. After reaching the pole of the heliosphere, the current flows along the assumed spherical surface of the heliosphere to its equatorial plane and then flows back to the solar equator along the magnetic equator. Akasofu et al.⁷ examined the magnetic field produced by such a unipolar current system, assuming that the radius was taken to be 20 au (so considered at that time) within in the interstellar magnetic field. Figure 2 shows an example of the configuration of magnetic field lines, which originate at less than 10° from the pole. It can be seen that the eastward-directed magnetic field line (B) tightly surrounds the boundary of the heliosphere. Thus, since the current J flows equatorward along the spherical surface from the heliospheric pole toward the equator, the J x B force on the boundary can accelerate plasma outward from the boundary. The above is just an example of the outside forces. Since the corona cannot be heated from the photosphere, such an idea of outside causes might be considered in the future.

Figure 2 Two views of a magnetic field line originating at 10^o from the pole.

The presently accepted theory of the formation of sunspots Babcock⁸ relies on the undetected and thus unproven magnetic flux below the photospheric surface. There are several problems associated with this theory, which must be addressed. Figure 3(A) shows magnetic fields on the solar disk. (1) There are unipolar regions, which are large-scale longitudinal bands, aligned alternately in longitude. (2) There are locally concentrated and scattered fields; they are pores and single spots (often called independent spots or isolated spots). (3) There are clustered single spots located mostly at the boundaries of neighboring (positive and negative) unipolar regions. They form pairs among themselves. The most important point here is that positive local fields of (2) and (3) are present in a positive unipolar region (vice versa). These fields are schematically shown in Figure 3(B). In the magnetic tube theory, spots should always appear as a pair. Thus, the presence of single spots is contrary to the tube theory. Further, one can see the pair formation occurs at the boundaries of unipolar regions (positive and negative), where positive and negative clusters of single spots are respectively present. The pairs of spots do not form in the middle of unipolar regions. If magnetic buoyancy is the cause for the magnetic tube to rise above the photospheric surface, pairs of spots can appear any place. This is not the case. When we examine single spots in high resolution images, they consist of several pores; Figure 3(C). As noted earlier, positive single spots appear only in a positive unipolar region. It is likely that positive spots are born in a positive unipolar region by the coalescence process of pores. Thus, it is suggested that unipolar regions are the source of single spots. Further, it seems that clusters of single spots are formed at the boundary of neighboring unipolar regions, forming pairs; Figure 3(B).

Figure 3 (A) The distribution of magnetic fields on the solar disk. (B) Schematic distribution of magnetic fields, pores, single spots and clusters of single spots in both positive and negative unipolar regions, respectively. (C) A high resolution image of a single spot. (D) The distribution of electric currents around a single spot Kotov⁹

It is known also that large spots are coalescence of single spots McIntosh¹⁰ In most cases, they form in the clusters at the boundary, not in the middle of a unipolar regions. In considering the coalescence, it is interesting to know that Kotov⁹ showed that a single spot is surrounded by electric currents of about 10¹² A; Figure 3d. The presence of the current around a spot may provide a hint of a radial motion associated with Vx B, in which V represents converging motions, which are crucial in the coalescence process from pores to single spots and single spots to large spots. Thus, it is crucial to study the formation of sunspots in terms of electric currents. Unfortunately, sunspots have been hardly discussed in terms of electric currents in the past. The basic difference between the tube theory and the present morphological consideration is that the present discussion is developed on the basis of the observed unipolar regions on the phosphoric surface, while the tube theory is based on an assumed magnetic flux tube below the photospheric surface, which has not been detected yet.

Solar flares are an electromagnetic energy dissipation phenomenon, so that the process should be discussed as a chain of processes, which consists of power supply (dynamo), transmission (currents/circuit) and dissipation (solar flares). However, such a basic approach, which considers a photospheric dynamo, has long been dismissed in the past; it had been thought that a dynamo process cannot explain the explosive feature of flares. Instead, solar flares are long been discussed almost exclusively in terms of magnetic reconnection, in which an anti-parallel magnetic configuration annihilates itself. They could not consider that a photospheric dynamo can accumulate the power for explosive flare phenomena. Akasofu and Lee¹¹ considered a photospheric dynamo in a magnetic arcade. Figure 4(A) shows an example of photospheric dynamos, which can produce a two-ribbon flare. Figure 4(B) shows an example of spotless flares. Spotless flares are most basic form of flares, because they are the directly produced phenomenon by the dynamo, but have been dismissed as weakest flares. They show also an important fact that sunspots are not needed in producing solar flares, and thus the dynamo process is crucial in generating the flare energy. Figure 4c shows the electric currents along the arcade field lines , which are generated by the dynamo for a two-ribbon flares. Two ribbon flares occur where the currents are directed upward (by descending electrons). The estimated field-aligned current density in this case is 0.5 x 10^-4 A/m². The power of the photospheric dynamo is given by the Poynting flux P (w or erg/s):

 P = ò (E × B)·dS = V(B²/8π)S,

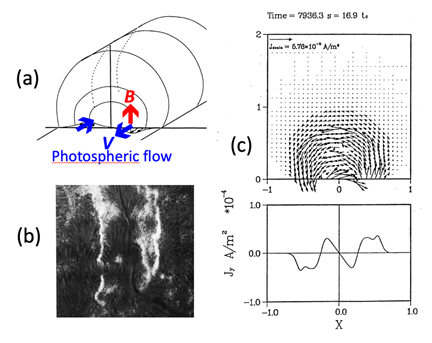
Figure 4 (A) The photospherc dynamo process along the magnetic arcade (Akasofu and Lee¹¹). (B) An example of spotless flares. (C) The current distribution in the magnetic arcade dynamo.

where S is the cross section. Akasofu and Lee¹¹ estimated that the power P is about 10²⁶ erg/s, which is enough for weak tow-ribbon flares. The photospheric dynamo in the above produces another current system. It is a loop current, which flows along the dark filament between the two ribbons, but above them; Figures 5 (A, B). The loop current can accumulate the power. In several hours, the accumulated power will have magnetic energy of W=(1/2)J²L=10³² erg for the current J=10¹¹ A and L=2000H, which can be supplied by such a dynamo. Thus, there is no reason to abandon a photospheric dynamo. When the loop current becomes unstable, it can explode, releasing the loop energy. There is one phenomenon, which has long been forgotten. This phenomenon is described in detail by Svestka¹² as ”dispartions brusques, (DB)”; it is basically the explosion of the dark filament, which coincides with a great enhancement of the two-ribbon emission, flare onset. Figure 5C shows the disappearance of a dark filament at flare onset (it reappears as an exploding prominence beyond the solar disk). DBs are likely be caused by a current instability in the loop current, which releases its magnetic energy. Chen and Krall (2003) estimated the current intensity along the loop filament can be as large as 10¹² A, when the expanding loop becomes coronal mass ejections (CMEs).

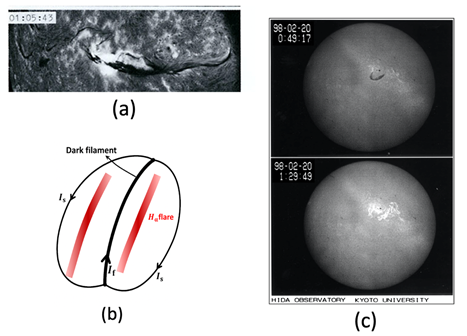
Figure 5 (A) An example of solar flares. An activated filament is present. Figure 5 (B) The magnetic arcade dynamo produces another current system, which includes a loop current, flowing along the dark filament. Figure 5 (C) The phenomenon called “dispartions brusque”. (Courtesy. E. Hiei, Norikura Solar Observatory).

It was Alfven¹³ who emphasized the importance of considering electric currents in space physics. In as early as 1967, he stated in his paper titled “The second approach to cosmical electrodynamics”: “Hence in order to understand the properties of a current-carrying plasma we must take account of the properties of the whole circuit in which the current flows”. In the same paper, he mentioned also that we can understand the physics involved better in terms of electric currents, than magnetic field lines; he had been very critical of the magnetic field line approach (MHD approach). It is unfortunate that his emphasis has long been dismissed. Therefore, the fundamentally different approach, the electric current approach, is taken in this paper to consider the four major problems in understanding each difficult subject.

The author thanks the Sydney Chapman and Hannes Alfven for their guidance in his study of electric current approach. He acknowledge many discussions on the topic with a large number of his colleagues.

Author declares there is no conflict of interest.

1. Van de Hulst HC. In The Sun. In: By GP Kuiper, editor. Univ. Chicago Press. 1953. p.207–321.
2. Van Doorsselaere T, Srivastava Abhishek K, Antolin Patrick, et al. Coronal heating by MHD waves. Space Sci Rev. 2020;216–140.
3. Karlsson T. Auroral Phenomenology and Magnetospheric Processes, Earth and Other Planets. In: A Keiling et al., editors.  2012, Geophys. Monograph Series 197, AGU, Washington, DC. 2012. p. 227–239.
4. Velli M, LK Harra, A Vourlidas, et al. Understanding the origins of the heliosphere: integrating observations and measurements from Parker solar probe, Solar orbiter, and other space- and ground-based observations. A & A. 2020;642:A4.
5. McComas DJ, Angold N, Eliott HA. Weakest solar wind of the space age and the current “mini” solar maximum. Astrophys J. 2013;779:2–10.
6. Alfven H. Cosmic Plasma. D Reidel Pub. Co.  Dordecht, Holland. 1981.
7. Akasofu SI, Gray PC, Lee LC. A model of the heliospheric magnetic field configuration, Planet. Space Sci. 1980;28:609–615.
8. Babcock HW.  The topology of the sun’s magnetic field and the 22-year cycle. ApJ. 1961;133:572–558.
9. Kotov VA.  IAU Symposium No.41, held at the College de France, August 31-September 4, 1970, 213-219. In: Howard RDD, editor. Reidel Pub. Co., Dordrecht-Holland. 1971.
10. McIntosh SW. The physics of sunspots. Sacramento Peak Observatory conference, held at Sunspot, New Mexico, 14 - 17 July 1981. 1981;7:7–54.
11. Akasofu SI, LC Lee. On the explosive nature of auroral  substorms and solar flares: The electric current approach. J Atmos and Space Phys. 2019;186:104–115.
12. Sveska Z. Solar Flares. D.Reidel Publishing co. 2019.
13.  Alfven H. The second approach to cosmical electrodynamics. The Birkeland Symposium on Aurora and Magetic Storms. In: A Egeland,  et al., editors. Centre National de la Resercherche, Paris, 1967. p. 439–444.