The usual circular Sagnac experiment is pictured in Figure 1. Consider a disk rotating at constant angular velocity ω and an observer with a clock O∗ stationary on a point at radius R, the origin of a co-rotating reference frame S∗. As indicated in Figure 1, this observer sends two light signals (as in the Sagnac experiment), or two particles (or two clocks, as in the Hafele-Keating experimen28) at velocity C around the disk circumference in opposite directions, respectively. Equivalently, we may consider the Sagnac experiment performed in a conveyor belt, as shown in Figure 2, which has been used to describe a modified, but equivalent, "linearized" version of the Sagnac effect.29
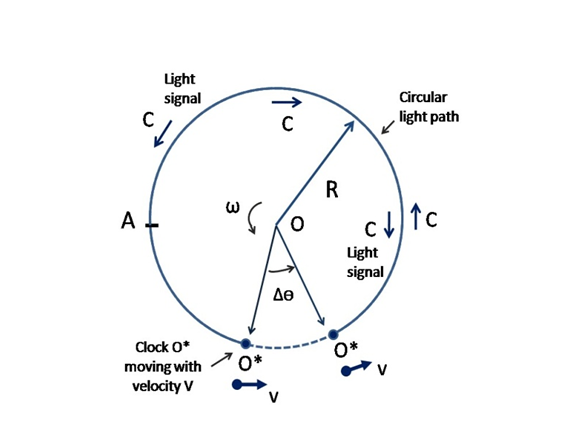
Figure 1 Clock O∗(originally at point A) is fixed on the circumference of the disk of radius Rrotating counter-clockwise at the angular velocity ωwith respect to the laboratory frame S. Two light signals are sent around the circumference of the disk in opposite directions starting from clock O∗, which measures the time span on the return of each signal to O∗.
We wish to know the time span observed by O∗ on the return of each signal on the disk. First, we consider the description given by an inertial observer O at the center of the disk stationary in the laboratory frame S. The observer O∗ at R is co-moving with the disk with angular velocity ω and possesses the tangential speed
. If the laboratory frame S is chosen as the preferred frame, the description of the Sagnac effect and corresponding time span variation
in such a frame is the same for Newtonian mechanics and relativistic theories with any synchronization. In general, with different speeds
and
in the counter-rotating (+ clockwise) and co-rotating (- counterclockwise) directions, respectively, the result, for the propagation time
and
of the signal in S, is
(1)
where
and
The local time of O∗ runs slower than the time of O by the time dilation factor
, so that for O∗ the light signals take the times
and,
(2)
where
is the time span variation measured by the clock O∗ and the last term corresponds to the case
.
With reference to Figure 1, for the observer O∗ on the disk at
, the circumference length corresponds to the proper ground length of the path followed by the signals and is given by
. For the usual case
, the time spans are
. Then, for the Sagnac effect, the average speeds of light in the counter- and co-rotating senses are
≃
,(3)
Where the results
correspond to the speed of light in vacuum,
Different interpretations of the sagnac effect
Besides other properties, the Sagnac effect is widely recognized as an optical experiment capable of indicating the state of rotation of the interferometer.30 We mention here only some of the several contrasting interpretations of the Sagnac effect, originally thought to disprove special relativity.20 Landau and Lifshitz31 trace the physical cause of the existence of the Sagnac effect in the rotating reference system as due to a difference between the velocities of counter-propagating waves. This velocity difference, which refers to the average velocities in (3), leads to a paradox according to the interpretation of Selleri,22 who shows that when the radius of the rotating disk is increased to R → ∞ keeping constant
, the local speed of light in an inertial frame would be c + vor c − vdepending on the direction of propagation, in disagreement with the unique value c foreseen by the second postulate of special relativity. The existence of Selleri’s paradox has been supported by other papers32-37 where the authors claim that the phase difference of counter-propagating waves in the reference system co-rotating with a ring interferometer, calculated in the context of standard SR, is equal to zero. Instead, Malykin38 argues that we are rather in the presence of a Zeno paradox because, considering that the rotating disk is not an inertial frame, the interpretation of the Sagnac effect is reliant on the equivalence principle and the effect of time dilation in a gravitational field.
The argument against Einstein synchronization can be resumed as follows. Because of the motion of O∗, for the observer O of the laboratory frame S, the paths ± of the signal have different lengths,
and, since the velocity c of the signal is the same in both directions, it appears obvious that we must have different propagation times t±. For O∗, instead, the propagation paths have the same length
but still we have different propagation times,
. Thus, for propagation on paths of the same lengths, if the respective propagation times,
, are different, different speeds
(and not the same speed c) are expected. This argument, which seems to cast doubts about the validity of a universal constant speed of light c, has been expanded by Selleri who claims that the paradox can be solved only if relativistic theories adopt the "natural" absolute synchronization that foresees the different speeds
of expression (3).
In this context, it is worth recalling the arguments of Kassner about Selleri’s paradox. In rebutting Selleri’s claim, Kassner21 attempts to refute the paradox by explaining the Sagnac effect in the frame of the co-moving observer O∗ in two ways: through Minkowsky’s analysis and by means of the absolute synchronization. The point is that, if Einstein synchronization is applied along the closed circular path, the resulting average speed in (3) is c, and not c + v or c - v. Thus, in this case, the Sagnac effect invalidates standard SR. After his Minkowsky analysis, Kassner concludes by acknowledging that “Einstein synchronization fails when performed along a path around a full circle”, i.e., on a closed path on the rotating disk, a failure that has also been observed by Weber39 and earlier by Anandan.40
Thus, in order to account for the resulting unphysical time discontinuity arising from the speeds
and
and solve Selleri’s paradox, Kassner introduces the unusual concept of a “time gap” on the rotating disk and states, “the speed of light is c everywhere except at the point on the circle where we put the time gap. The position of this point is arbitrary but there must inevitably be such a point.”
The solution based on Minkowsky’s analysis presented by Kassner has been objected to by Gift41 who rejects Kassner’s adjustable “time gap” based on an unphysical time discontinuity claiming that is a theoretical construct that has no basis in reality. Similarly, Selleri has claimed that "it is not true that the synchronization procedure can be chosen freely because Einstein convention leads to an unacceptable discontinuity in the physical theory". Moreover, according to Selleri "Very probably the above discontinuity is the origin of the synchronization problems met with by the Global Positioning System". In relation to this, Gift emphasizes that the failure of Einstein synchronization is well known to GPS engineers who discovered41,42 that they cannot synchronize GPS clocks fixed on the rotating Earth using Einstein synchronization.43,44
The second argument presented by Kassner21 to solve Selleri’s paradox consists of replacing Einstein synchronization with the absolute synchronization. Thus, instead of the Lorentz transformations and without citing the related literature, Kassner makes use of the Tangherlini transformations. Considering that the one-way speed of light is synchronization dependent, for Kassner a difference from c does not constitute a problem because the observable two-way (average) speed of light remains c, as in the interpretation of the Michelson-Morley experiment. In fact, if synchronization is arbitrary (as also assumed by Kassner), the two synchronizations are equivalent and provide the same observable result, as they actually do within Kassner’s basic set up and hypotheses. Then, although acknowledging the anisotropy due to the different speeds c+v and c−v in Selleri’s paradox, Kassner argues that the difference is to be interpreted in terms of the arbitrariness of synchronization and, thus, does not invalidate the second postulate of special relativity. Yet, in Sect. 4 we demonstrate that, in general, Einstein synchronization and the absolute synchronization are not physically equivalent. Therefore, the assumption of conventionalism is groundless, so that Kassner’s argument based on it is untenable. Moreover, while adhering to the conventionality of the speed of light and the equivalence of the two synchronizations, Kassner does not discuss the consequences of this assumption on the countless physical theories traditionally based on the LT. These physical theories would be substantially modified if they were based on and developed by means of the TT. In the next section, we review the arguments in favor and against Einstein synchronization and the problem of superluminal speeds within the context of the "linearized" Sagnac effect, shown in Figure 2.
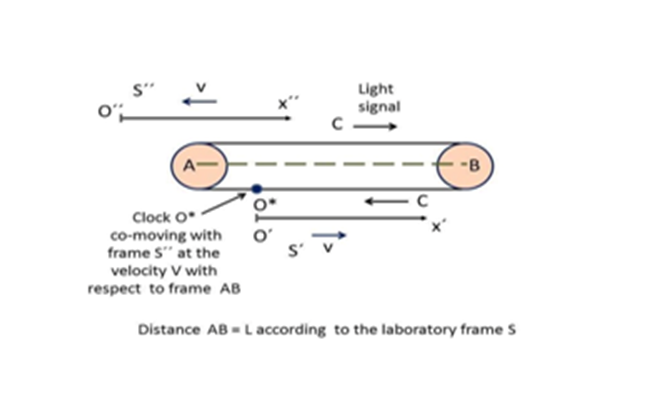
Figure 2 The conveyor belt system represents a "linearized" version of the Sagnac effect. The belt stands for a flexible tube and the signals propagate in opposite directions along the closed path inside the tube, acting as a wave-guide and rotating counter-clockwise while leaning over the two pulleys separated by the arm AB of fixed length L. The inertial frame S’’ is co-moving with the upper part of the tube with velocity vwith respect to the arm AB, stationary in the laboratory frame S, while frame S’ and clock O*are co-moving with the lower part of the tube in the opposite direction.
With reference to Figure 2, we assume that, if the distance L between the two pulleys of small radius r is long enough (r << L),
the time
spent by the signals in the short portion of the tube in contact with the pulleys will be negligible with respect to the total time of flight and we may omit it. Furthermore, the acceleration
that could cause a change in the flow of time of clocks co-moving with the tube is constant and depends on vand r. Therefore, the change due to the effect of the acceleration does not depend on L and, if the distance L is long enough, becomes negligible with respect to the time variation
, proportional to L, observed in the Sagnac effect.
If the tube where the light signals propagate is filled with a co-moving medium (for example, a fluid of refractive index n where the local speed of light is
) the speed of light is no longer c but C0. In this more general case, the two signals circumnavigate the tube in opposite directions with the same local (ground) speed
with respect to the tube.
- For special relativity with the LT.
For simplicity we consider here the case
We have,
(4)
(5)
It can be shown that the Sagnac effect, related to
is foreseen to be independent of the signals ground speed
, for both the circular and linear cases.
- For a preferred frame theory based on the TT.
The TT between the preferred frame Sand the moving frame S′ are:
. Using the TT, with
and
, we have
which implies no Sagnac effect independently of
, the same result as in Newtonian mechanics.
From a mathematical perspective, the internal consistency of the LT is not questionable and not questioned here. In the following, we try to adopt an objective point of view, simply presenting the arguments in favor or against Einstein synchronization, with the aim of clarifying the issue of superluminal average speeds in the context of the Sagnac effect. The incompatibility of Einstein synchronization over a closed path has been discussed above in Sect. 2. The objection that could be made in the context of the circular Sagnac effect is that the rotating frame is a non-inertial frame and, thus, Einstein synchronization is not applicable in such a frame. Here, with the linear Sagnac effect, we may apply Einstein synchronization separately to the inertial frames S′′ and S′ co-moving respectively with the upper and lower part of the tube. Still, we find that the mentioned incompatibility subsists and we are met once more with superluminal speeds and the conflicting point of views, or "realities", of S’and S’’.
The Lorentz transformations from S’’ to S’ are,
(6)
with
and
.
The crucial question for standard SR is: How does the observer O∗ manage to explain the observed time span difference for two signals counter-propagating along a path of the same length and with the same ground speed
For our example, we choose a special case where the effect of non-conservation of simultaneity is made apparent. For theexperimental set-up shown in Figure 3, we assume that the three origins O, O′, O′′ of frames S, S’, S’’, coincide at the initial time
, while the pulley at A is positioned at the distance
to the left with respect to O, and the pulley at B at the distance
to the right. For our purposes, it is sufficient to consider the clockwise propagation of a light signal and determine its time of flight starting from the clock
, co-moving with the tube and coincident with the origin
at
, and returning to
after a complete cycle. The single clock O∗ alone is used to determine the time interval of the closed trip and, therefore, no synchronization procedure is involved. The two physical realities of S’’ and S’ will be confronted.

Figure 3 The linear Sagnac effect described from the perspective of frame S′′. The arm AB of length L, fixed in the laboratory frame S, moves with velocity vwith respect to S’’, stationary with the upper part of the tube. Frame S’, co-moving with the lower part of the tube, moves with velocity w with respect to S′′ and velocity v with respect to S. The origins O′′, O′ coincide with the origin O of frame S at t = t′ = t′′=0, when
the light signal is sent counter-propagating at the velocity
. At the time
, the signal has reached point B while clock O∗ has simultaneously reached point A.
Physical reality and total time for the clockwise trip according to S’’
Clocks at rest in some inertial reference frame may be “internally” synchronized according to Einstein’s procedure. Without reference to other inertial frames in relative motion, to say that the clocks at rest along the x′′ of the inertial frame S’’ are synchronized, it implies, for both Newton and Einstein, that in S’’ the clocks show the same readings simultaneously. Within a given inertial frame, “internal” simultaneity or, simply, simultaneity, is a reflection of internally synchronized clocks. Then, physical phenomena are described in S’’ as a function of the time t′′, the same time simultaneously displayed by all the synchronized clocks. If a hypothetical signal is sent at infinite speed along the x′′ axis of S’’, all the synchronized clocks on the x′′ axis will display the same time simultaneously when the signal passes by. At least in principle, clocks can be properly (internally) synchronized by signals with either finite or infinite speed, if the latter were available. The same conclusions apply for S’. For both Newton and Einstein, the concept of (internal) simultaneity applies when keeping within the physical reality of any inertial frame. The difference between Newton and Einstein shows up only when we relate two inertial frames in relative motion by means of the space-time coordinates transformations. In fact, as is well-known, if the hypothetical signal sent along the x′′ axis of S’’ had infinite speed also along the x′ axis of S’, there would be a conflict with the Lorentz transformations that assume the relativity of time and foresee different, non-simultaneous time readings between the two sets of clocks of S’’and S’. Supporters of special relativity overcome this problem by arguing that a signal with infinite speed is not operationally acceptable within the theory, which requires c to be the maximum speed permitted. However, given that the concept of simultaneity holds (internally) for both Newton and Einstein when keeping within the physical reality of a given frame, we may say that physical phenomena are portrayed in that frame as a function of the same "absolute" time. Thus, the restriction imposed by the finite speed c does not impede to conceive that, if spatially separated clocks are synchronized, they will display the same time simultaneously when a hypothetical infinite-speed signal passes by. The same is true for the synchronized clocks of any other inertial frame.
What is to expect in any case, is that the related physical realities of the two inertial frames in relative motion are compatible and reflect the same objective physical reality. In our analysis of the linear Sagnac effect, we shall consider the compatibility of the internal simultaneity of an inertial frame S’’ with that of another generic inertial frame S’ in relative motion with respect to S’’. As shown below, we find that internal simultaneity is not compatible with Einstein synchronization and the Lorentz transformations, because the latter imply, for S’’ and S’, two contrasting and irreconcilable physical realities.
We calculate now the time taken by the signal to reach point B after leaving the clock O∗. Since, at
point B is at the distance L(1−v/c)/γ
to the right from the origin
and point A is at vL/γc
to the left, the signal reaches B at the time
(7)
and at the same time
clock
reaches point A, the two events at A and B being simultaneous. In the time span
the signal has covered the ground length
of the upper part AB of the tube at the local speed c. As shown in Figure 3, after the clock O∗, initially co-moving with the origin O′′, reaches point A, it turns around the pulley and goes toward point B, co-moving now with the lower part AB of the tube and the x′ axis of S′.
Frame S’’and S′ agree on and share the common value for the total invariant proper length 2γL of the tube where propagation takes place. It follows that both S’’ and S′ agree that, after changing direction at B, the signal is bound to cover the remaining proper length
from B to O∗ by traveling entirely in the lower part of the tube until clock O∗ is met, as apparent from Figures 3, 4, and 5. For S’’, the ground length of the lower part of the tube is
, which (because of its relative motion) is seen as being contracted by the factor
to
and, thus, it fits exactly the length
of the segment AB. Conversely and in contrast with the reality of S’’, frame S′ estimates that it is the upper part of the tube that has ground length
, seen by S′ as being contracted by the factor
to the value
. At this point, detractors of Einstein synchronization may point out the following two arguments against the validity of Einstein synchronization.
- They may first argue that the time interval
, corresponding to propagation in the lower part of the tube, can be inferred by S’’, knowing that the ground speed is c also in that lower section of the tube. In fact, the ground length of the lower part of the tube,
, to be covered by the signal, and the corresponding ground speed c of the signal are known. Thus, an observer of S’’ may say that, whatever may be the time displayed by a clock of S′ when the signal starts propagating along the x′ axis, this clock will have advanced by the time interval
, after the signal has completely covered the ground distance L′. About the corresponding elapsed time of clock
, we need to consider that, when co-moving in the lower part of the tube, clock
is sharing the same properties of the clocks fixed to the x′ axis. Therefore, since for S’’clock
is always co-moving with the lower part of the tube while the signal is propagating along this lower part, the time interval by which
advances must be the same as that of any other clock of S′. It follows that, as inferred by S’’, the proper length
must be covered by the signal in the time interval
(8)
as measured by clock O∗ or any other clock of S′.
S’’ can claim the above result to be a necessary consequence of the observed physical reality shown in Fig. 3, reflecting the simultaneity of the two events: signal at point B and clock
at point A. For S’’, this reality requires that the signal has still to travel the ground distance
at the ground speed c after the clock
reaches point A and, therefore, result (8) must inevitably hold. Then, for
, the total time
displayed by clock
for the clockwise signal propagation must be,
(9)
For the counter-clockwise propagation, the result is the same. Therefore, the propagation time difference for the light signals is
, and no Sagnac effect is foreseen if the local speed of the signal is
everywhere along the tube.
b) The second argument that detractors of standard special relativity may point out are the following.
Let us suppose that in (9) we have
, in agreement with special relativity (see (4) and (5)) and experimental observation. Then, we may write
(10)
and, correspondingly,
(11)
where, on the lhs of (11),
is the total length covered by the signal, and c/(1−v/c) ≃c+v
is the corresponding superluminal average speed. On the rhs of (11), the length L/γ
of the first term represents the length covered by the signal in the upper part of the tube and
the corresponding local speed. The length
in the second term represents the length covered by the signal in the lower part of the tube and
the corresponding local speed. Simple algebra indicates that the local speed
, in the lower part of the tube, must be , i.e., superluminal, in contradiction with Einstein’s second postulate, as pointed out by Selleri. It follows that internal simultaneity, as assumed by both Newton and Einstein, is not compatible with the Lorentz transformations based on Einstein synchronization.
Physical reality and total time for the trip according to S′
We have shown in the previous section that, according to the physical reality of S’’, the local speed of light in the lower part of the tube, co-moving with S’, must be superluminal. In order to restore the local speed of light to c in this lower section of the tube, frame S’ has to synchronize clocks ("de-synchronize clocks", would argue a detractor) by means of Einstein synchronization, so that the LT can be used.
In this way, the physical reality of S’corresponds now to the reality of S’’changed, or "transformed", by means of the LT (6) as shown in Figure 4. It follows that, according to S’, simultaneity no longer holds because the signal reaches point B at the time
while clock
reaches point A at the later time
being
(12)
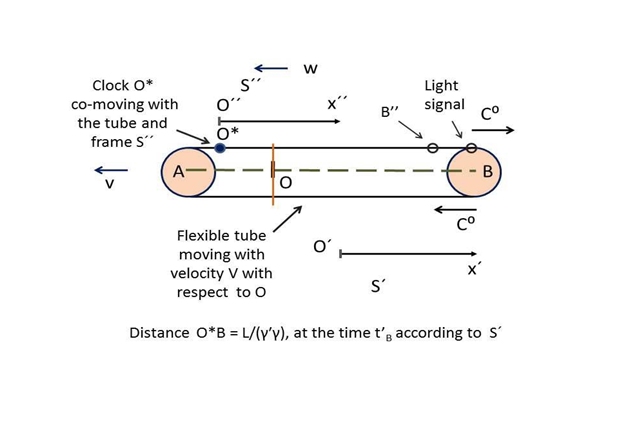
Figure 4 The linear Sagnac effect described from the perspective of frame S′ at the time
. The origins O′′ and O′ were coinciding with the origin O at t = t′ = t′′= 0. At the time
the signal has reached point B while clock O* has not yet reached point A. At time
, the reading ofclock
is
, while the proper ground length
is
. Hence, the corresponding ground speed is
the time interval (Kassner’s time gap) introduced by non-conservation of simultaneity. Fig. 4 pictures the physical reality of S’ at the time indicating that O∗ is at the distance
from B and at the distance
from A. The physical reality of S’ at the later time
is pictured by Figure 5, indicating that when clock
reaches A the signal has already traveled from B toward point A in the lower part of the tube during the time interval δt′ and is now at the shorter distance
from A and clock
. According to S’, as a consequence of
non-conservation of simultaneity the signal is not located at point B at the time
, but has already moved in the lower part of the
tube from B toward
by the amount
, as shown in Figure 5. Meanwhile, in the same time interval
point A has moved with respect to O'
by
. Thus, at the time
, the remaining shorter distance to be covered by the signal is
′, so that when the signal finally reaches
this clock must have advanced by the time interval
(13)
Then, according to S’, the total time displayed by clock O∗ at the end of the clockwise cycle is
(14)
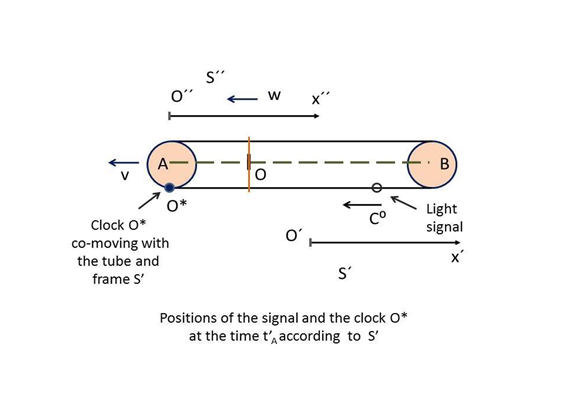
Figure 5 The linear Sagnac effect described from the perspective of frame S’ at the time
. Clock
has reached point A and, after turning around the pulley, starts co-moving with the lower part of the tube and frame S’. Because of the swifter ground speed
in the upper part of the tube, the signal has already moved along the lower part of the tube advancing by
from B toward
.
In agreement with (10) and (11) as foreseen by special relativity. For the counterclockwise propagation, we find
and thus, being
as in (5), the LT applied in frame S′ correctly foresee the Sagnac effect. It should be pointed out that the difference between (8) and (13) is precisely the time interval (12),
, introduced by non-conservation of simultaneity. As pointed out above, according to S′ and in agreement with standard special relativity, result (14) indicates that, for
, the average counter-propagation speed, along the invariant ground length
of the closed path, is superluminal and given by
.
Supporters of special relativity may claim that, although the average speed is superluminal, after Einstein synchronization the local speed in the lower part of the tube co-moving with S’is c, as it should be. Nevertheless, detractors of Einstein synchronization may argue that the local speed in the lower part of the tube has been achieved by re-setting the clocks in that part of the tube in such a way that the speed be c. For doing this, frame S’ has to pay a price because now the average superluminal speed
along the closed path
cannot be justified by S’, unless the ground speed in the upper part of the tube is not only superluminal, but greater than the average value
and of the order
. In fact, by synchronizing clocks with Einstein procedure, S’ has introduced the kinematical mechanism of non-conservation of simultaneity and the physical reality of
has been changed. The resulting effect consists of modifying the time interval (8), making it shorter by a first order contribution in
in order to equal the value (13). Because of non-conservation of simultaneity, when seen from the snapshot perspective of S’shown in Fig. 4, the clocks of S’’ in the upper part of the tube display readings predicted by the Lorentz time transformations. If the perspective of S’ is taken realistically (and is not a mere mathematical makeup), the reading of O*, at the time
, can be inferred by means of the inverse Lorentz transformations, so that the time reading of clock O*
(at x′′ = 0) turns out to be
(15)
Obviously, the clock reading
represents the time of flight
of the signal from O* to the point of the tube reached by the signal at that time. According to S’’, at the time
the light signal has not yet reached point B but has covered only shorter ground length
to the point B′′ shown in Fig. 4. For the Newtonian mindset, the fact that, at the displayed time
, the signal can be in two different places, at B or B′′, depending on the point of view of S’ or S’’, hints at an inconsistency between the two physical realities. However, if we admit non-conservation of simultaneity and focus on the reality of S’, it is a fact that, at the time
, the signal has already reached point B, past B′′.
Thus, we may conclude that the signal has been sped up by non-conservation of simultaneity, while propagating in the upper section of the tube from clock O∗ to point B, past B′′. Considering that the ground length O*B is
and that
is the time of flight of the signal, we are compelled to infer that the local ground speedof the signal along O∗B is,
(16)
i.e., superluminal for
, in conflict with the postulate of the constancy of c
In short, the argument discussed in b) in the above subsection, holds here also. If the average speed over the total length
is
, and, over the partial length
of (13) in the lower part of the tube, the local speed is c, it follows that the local speed in the remaining part must be
, i.e., superluminal.
Preliminary conclusions
The two physical realities of Figure 3 (for S’’) and Figure 4 (for S’) are physically incompatible and lead to different results and interpretations of the Sagnac effect. For both Newton and Einstein, in an inertial reference frame the physical reality is described synchronously, i.e., at the same simultaneous time in every point of space of the inertial frame. The contrasting results obtained by S’’ and S’((9) and (14), respectively) are simply the reflection of the incompatibility between the concept of simultaneity and Einstein’s concept of relative time.
Non-conservation of simultaneity and the Lorentz transformations are direct consequences of Einstein synchronization and the validity of the second postulate of special relativity. Detractors of Einstein synchronization may claim that the resulting average propagation speed
of (14) implies signal propagation at a superluminal local ground speed along some section of the closed path, in line with Selleri’s paradox for the circular Sagnac effect. We have shown that, for the equivalent linear Sagnac effect, if in one of the inertial frames, S’’or S’, the local ground speed is c, the local speed in the other inertial frame must be superluminal and of the order of
, in contrast with Einstein’s second postulate. This result is seen as reflecting the incompatibility of Einstein synchronization applied to the closed path of the Sagnac effect, even when applied separately to the upper and lower parts of the tube. Therefore, since Einstein synchronization implies the existence of superluminal local speeds, the second postulate of SR is invalid.
Nevertheless, thinking beyond the controversy about the validity of the postulate of the universal light speed c in all inertial systems, supporters of SR may shift the debate to a more pragmatic scenario by highlighting the correctness of the predictions of the theory. They could argue that, even though the second postulate of SR is questionable because of the superluminal speeds involved in the interpretation of the Sagnac effect, from an operational standpoint, Einstein synchronization can still be applied to the upper and lower parts of the tube. Therefore, from a pragmatic point of view, followers of standard special relativity may claim that the theory is sound because, by means of non-conservation of simultaneity, it predicts for the Sagnac effect the observed results, derived either in the laboratory frame S or in the tube co-moving frame S’’or S’. Along these lines, assuming the physical equivalence between absolute and Einstein synchronization, Kassner argues that the difference between a local speed c and a superluminal average or local speed
is to be interpreted in terms of the arbitrariness of synchronization and, thus, does not invalidate the second postulate of special relativity.
Thus, the debate of relative versus absolute time is at a standstill and it is unlikely that historically long debates such as this can be settled through mere theoretical arguments. Fortunately, physicists agree that, if there is a meaningful difference between opposing points of view, it must be ultimately observable, i.e., experimentally testable. Therefore, the best clear-cut way to solve the controversy is by means of a test capable of discriminating absolute versus Einstein synchronization. As shown in the next section, such a test exists and is precisely the Sagnac effect.
Regardless of the feasibility or not of experimental verification, the mere existence in principle of such a test proves that the Lorentz transformations are not physically equivalent to transformations based on absolute synchronization. An immediate consequence is that it is conceptually unfeasible to use the Tangherlini transformations and absolute synchronization in trying to conciliate, as claimed by Kassner, the superluminal average speed of the Sagnac effect with the postulate of the constancy of the speed of light. In principle, the two synchronizations are physically distinguishable and the arbitrariness of synchronization can no longer be invoked to substitute the LT with the TT in order to explain Selleri’s paradox, which remains unsolved.
Non-equivalence of Einstein and absolute
synchronization
In relativistic theories, the coordinate transformations between inertial frames of reference in relative motion may take into account the synchronization procedure adopted (for example, internal and external ) through the dependence of the time ton a convenient synchronization parameter ε. The contention of conventionalism is that clock synchronization is arbitrary and, thus, the one-way speed of light, that can be expressed as
, is conventional and not measurable in principle. It follows that, within this scenario, an observable quantity must be synchronization-independent in order to be physically meaningful. If the observable quantities of relativistic theories are independent of the chosen synchronization procedure, all the experiments supporting standard special relativity also support a preferred frame theory with absolute synchronization. Then, non-conservation of simultaneity, which is a consequence of Einstein synchronization procedure, should not be observable per se. If, instead, synchronization is not arbitrary, it is feasible that some observable quantities are dependent on synchronization and, thus, their measurement would allow for the determination of the "natural" synchronization that fits with observation. In the case of the Sagnac effect, the propagation times over a cycle are measured by means of a single clock and there is no need to perform synchronization of distant, spatially separated clocks. If the laboratory frame S is chosen as the preferred frame where space is isotropic, the time relation t*= t/γ between clock O∗ in motion with relative velocity v and a clock stationary in Sis the same for TT and LT. Therefore, both theories foresee the same results, independent of ε. However, it is unlikely that the laboratory where the Sagnac experiment is performed, located somewhere on the rotating Earth, should coincide with the preferred frame of some ether theory. Thus, it is justified to consider the hypothetical case when, in frame S, space is no longer isotropic, as a consequence of its possible motion with respect to some preferred frame. Let us then envision the existence of the preferred inertial frame
where space is isotropic and the one-way speed of light is c. In the Figure 2 we have to suppose that the laboratory frame S, where the set-up (arm AB) of the linear Sagnac effect is stationary, moves with velocity Win the Xdirection with respect to Σ. The clock
, co-moving with frame S′, has velocity
with respect to Σ. The following transformations8 apply to relativistic theories (special relativity with arbitrary synchronization parameter ε):
(17)
In Equation (17), when
we recover the time relation
of the LT and, when
, the relation
of the TT. After taking the derivative
, for the components of the velocity in the x, X direction, we obtain from (17),
(18)
,p>Where
is the velocity with respect to the lab frame
Sand
is the velocity with respect to the preferred frame Σ. If
is the velocity with respect to Σof the clock
(or interferometer) co-moving with
S′, from (18) we find that the corresponding relative velocity
of the clock with respect to
Sis,
(19)
We can see that for the
the velocity composition (19) gives, as expected,
, while for the
we have
. Expressed in terms of Wand v, the velocities of S′ and S′′ with respect to Σ are respectively,
;
(20)
The following results, which depend on ε, are obtained for the linear Sagnac effect. As measured in the preferred frame Σ, the time intervals for the counter-propagating signal (clockwise propagation in Figure 2) with one-way speed c from A to B and from B to are respectively:
.
The time T+ for counter-propagation and T− for co-propagation turns out to be, respectively,
(21)
where
is the time span difference. The corresponding time difference
measured by the clock
for the Sagnac effect is,
(22)
By means of (20), we may substitute in (22) W ′ expressed in terms of Wand
and obtain,
(23)
Result (23) indicates that the optical path difference for two counter-propagating electromagnetic waves depends on the synchronization parameter ε in the Sagnac effect. If the procedure
used above is applied to other optical tests, for example, to the Michelson-Morley experiment, we find instead that ∆t′ is independent of ε, as also shown by Mansouri and Sexl.
If we set
in (23), the term
becomes γWγ and as foreseen by the LT, we obtain
independent of W. Withε=0
, the coupled term vW/c2
does not vanish and, being attributable to the absolute synchronization, is present also in the Newtonian case. Thus, for the TT, from (23) we obtain,
(24)
To the order
and with
, the term [...]−1/2 in(24) becomes,
(25)
When, after reaching point B, the clock O∗ changes direction and moves with velocity −v with respect to S, the relation
has to be replaced by
, while the factor
has to be replaced by
in the
calculations. Furthermore, instead of the time interval ∆t′ = ∆t∗, we have now ∆t′′ = ∆t∗, which is the same as the expression ∆t′ of (24) with the term −vW/c2 replaced by +vW/c2.
Result (24) can be immediately extended to the circular Sagnac effect, where the clock moves with tangential speed
and its x-component is
, by writing
Then, with the help of (25), the time span (24) measured by clock
in the Sagnac effect can be expressed as
(26)
Precision measurements of (26) can provide the value of the component W of the absolute velocity of the laboratory frame with respect to Σ.
Concerning the smallest measurable time interval, there are techniques capable of resolving femtosecond (
) [45] or even attosecond (
)46,47 pulses of laser light while better limits may be achieved by means of advanced interferometer. In terms of phase shift, we may write
where λis the vacuum wavelength and c is the free-space velocity of light [30]. Then, result (26) can be expressed as
(27)
The amplitude Ain (27) could be more easily measured if interferometer techniques were available where the constant amplitude K is locked and fixed and we could estimate Wby resolving the relative fluctuation proportional to A=vW/c2
and alternating with frequency ω. In any case, the stability of the amplitude K, is probably the limiting factor in any such possible experiment because the inevitable vibrations of the table or pillar on which the experiment is mounted will be hard to cancel out altogether. Therefore, the "constant" Kwill have its own fluctuations which can exceed A. Hopefully, we can transfer some of the advanced techniques developed for gravitational wave detection,49 to the scenario of our experiment. There are vibration cancellation schemes available for wave interferometers, although very expensive. The phase measuring gravity wave detectors (which are the best in this category) keep Knear zero and, in special conditions by various enhancement techniques, one can theoretically hope for 10−9−10−11 of a fringe. Sometimes adding an optical cavity multiplies the signal by factor of 102.
Already at the time of Sagnac, for similar experiments [30, 49-50] the fringe shift was
. With this value for Kin (27) and with an optimal resolution of 10−13 of a fringe, requiring a lot of work and money, we can get an idea of the value of W that can be detected in some experimental conditions. Assuming that the smallest detectable value of
is
, with a velocity
(a value of the order of the tangential speed of the Earth at the equator), we have
as the minimum absolute velocity W detectable. Historically, the velocity W with respect to the preferred frame has been expected to be that of the Earth’s orbital speed
, in relation to the Michelson-Morley experiment) or the absolute speed of the Earth through space
, in relation to the observed cosmic background-radiation anisotropy). Therefore, we could hope to verify these two assumptions and even check if the hypothetical preferred frame is identifiable with the inertial rest frame of the center of the Earth
. Although the realization and the cost of the experiment are certainly quite challenging factors, we believe this test not to be completely outside the reach of present technology. We may conclude that the two synchronizations (Einstein and absolute) are not physically equivalent in principle, but almost equivalent in practice and, should experimental evidence favor the absolute synchronization, it is understandable why experimental discrepancies with Einstein synchronization have not been evidenced so far. In this case, we may state that, from an operational perspective, Einstein synchronization represents a useful simple approach to the procedure of clock synchronization, capable of reproducing approximately the results of relativistic theories based on absolute synchronization.
Nevertheless, we will show in a future contribution that, with a modified experiment of the Sagnac type, the variation in which should be more easily measurable.
Consequences of the non-equivalence of Einstein and absolute synchronization
In the case of the Michelson-Morley experiment, the observed null result rules out the Galileo transformations, which foresee a non-null outcome. Instead, the TT and LT are confirmed because both predict the observed null result. In the case of the Sagnac effect, the non-null result
W= 0
MathType@MTEF@5@5@+=
feaagKart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn
hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr
4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9
vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x
fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaqcfaieaaaaaa
aaa8qacaWGxbGaeyypa0Jaaeiiaiaaicdaaaa@39E3@
would confirm absolute synchronization, i.e., Galileo transformation and the TT, while disproving standard SR. If the experimental result indicates that
W= 0
MathType@MTEF@5@5@+=
feaagKart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn
hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr
4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq=Jc9
vqaqpepm0xbba9pwe9Q8fs0=yqaqpepae9pg0FirpepeKkFr0xfr=x
fr=xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGcbaqcfaieaaaaaa
aaa8qacaWGxbGaeyypa0Jaaeiiaiaaicdaaaa@39E3@
, the conclusion that can be drawn is the same as that of the Michelson-Morley experiment: the preferred frame coincides with the laboratory frame Sand both LT and TT are confirmed. However, it would be a conceptual error to conclude that they are equivalent, after having shown above that in principle they are physically distinguishable. A conclusive experimental proof consists of repeating the experiment having the Sagnac apparatus in motion with respect to S, identified as the preferred frame by the first experiment. Regardless of experimental verification, the mere existence in principle of such a test proves that absolute and Einstein synchronization are not physically equivalent. Even if the outcome of the experiment indicates the existence of a preferred frame of reference, the principle of relativity of physical laws holds. However, in this case, the principle has to reflect the invariance under transformations adopting absolute synchronization.
In conclusion, the countless theoretical approaches to modern physics based on the Lorentz covariance are substantially different from, and cannot be equivalently substituted by, approaches based on the invariance of physical laws under the TT. Therefore, it is conceptually unfeasible to use the Tangherlini transformations in trying to conciliate, as claimed by Kassner, the superluminal average speed of the Sagnac effect with the postulate of the constancy of the speed of light by invoking the arbitrariness of synchronization.







